
e-ISSN 2277-2685, p-ISSN 2320-976
IJESR/August 2014/ Vol-4/Issue-8/595-601
Aniveni Mahesh et. al./ International Journal of Engineering & Science Research
*Corresponding Author www.ijesr.org 595
DOUBLE DENSITY DUAL TREE COMPLEX WAVELET TRANSFORM BASED
SATELLITE IMAGE RESOLUTION ENHANCEMENT
Aniveni Mahesh*
1
, Ch. Sreedhar
2
, Ahmed Zeeshan
3
1
M.tech student, Global Institute of Engineering & Technology, Moinabad (A.P), India.
2
HOD, Project guide, Global Institute of Engineering & Technology, Moinabad (A.P), India.
3
Project coordinator, Assoc. Prof, Global Institute of Engineering & Technology, Moinabad (A.P), India.
ABSTRACT
Image Resolution enhancement (RE) schemes which are based on waveletshave an advantage overconventional methods
which suffer from the losing of high frequency contents which causes blurring. The discrete wavelet transform-based
(DWT) RE scheme generates artifacts due to a DWT shift-variant property. A wavelet-domain approach based on double
density dual-tree complex wavelet transform (DDDT-CWT) is proposed for RE of the satellite images and compared
with dual-tree complex wavelet transform(DT-CWT). A satellite image is decomposed by DDDT-CWT to obtain high-
frequency subbands. The high frequency subbands and the low-resolution (LR) input image are interpolated and the high
frequency subbands are passed through a low pass filter. The filtered high-frequency subbands and the LR input image
are combined using inverse DT-CWT to obtain a resolution-enhanced image. Objective and subjective analyses reveal
superiority of the proposed technique over the conventional and state-of-the-art RE techniques.
INTRODUCTION
Resolution of satellite images has always been a limiting factor for utilizing remote sensing data such as satellite imaging
etc.Spatial and spectral resolutions of satellite unprocessed images are related with each other that is a high spatial
resolution is associated with a low spectral resolution and vice versa[1]. So spatial and spectral resolution
enhancement(RE) are desirable.Another technique which is widely used for RE is interpolation [2,3].The interpolation
techniques which are commonly used are based on nearest neighbours which include nearest neighbour, bilinear, bi-
cubic, and Lanczos. These can also perform reduction of aliasing, sharpness and ringing [4].
Vector-valued image regularization based methods with partial differential equations(VVIR-PDE) [5] and inpainting and
zooming which uses sparse representations [6] are now the state of the art in the field.RE schemes which are not based on
wavelets suffer from the drawback of losing the high frequency contents which results in blurring effect.
In the research area of RE in the wavelet domain many algorithms such as discrete wavelet transform (DWT) [7],
stationary wavelet transform (SWT) [8], and dual-tree complex wavelet transform (DT-CWT) [9] have been proposed
[7–11]. Using DT-CWT an RE scheme was proposed in[9] and bicubic interpolations, and results were compared with
the conventional schemes i.e., nearest neighbour,bilinear, and bicubic interpolations and wavelet zero padding. Based on
DWT and bicubic interpolation a scheme was proposed in [7] and results were compared with conventional schemes and
the state of art schemes.Dwt is shift variant, which causes artefacts in the RE image,and has a lack of directionality,
whereas DT-CWT is almost shift and rotation invariant [13].
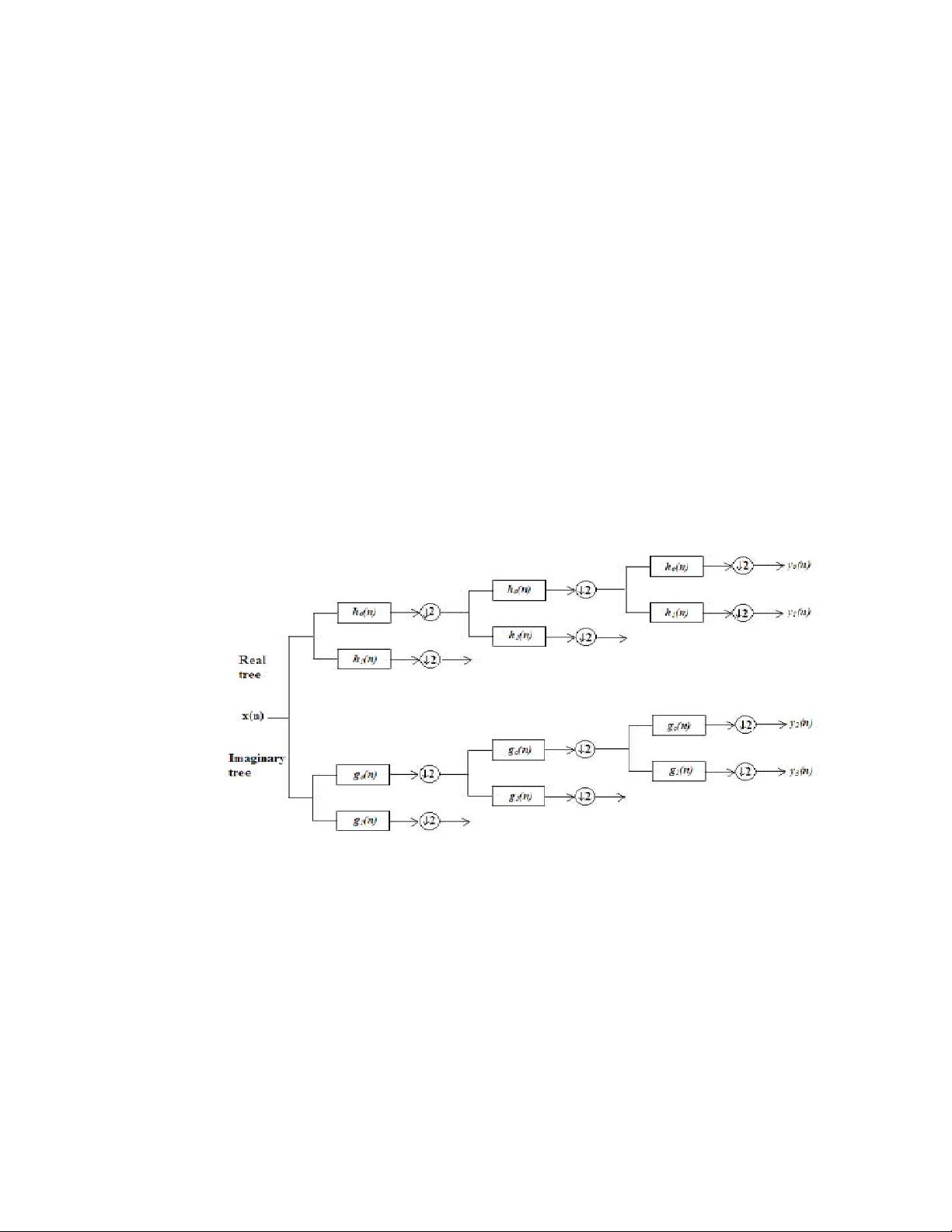
In this Paper a Double Density DT-CWT based lowpass filtering based RE technique is proposed using DT-CWT,
Lanczos interpolation, and lowpass filtering.Double Density DT-CWT is nearly shift invariant and directional selective,it
preserves the usual properties of perfect reconstruction with well-balanced frequency responses [13, 14]. As compared
with traditional DWT, DDDT-CWT provides promising results after the modification of the wavelet coefficients and
provides less artefacts.For spatial RE of optical images the results are compared with best performing techniques [5,7–9].
2. WAVELET TRANSFORM DOMAIN
Using Fourier Transform (FT) only the global frequency content of a signal is retrieved, the time information is lost. By
using wavelet analysis a multi-resolution analysis is possible. The frequency and time content of a signal is retrieved by


 dual-tree-complex-wt.rar (1个子文件)
dual-tree-complex-wt.rar (1个子文件)  dual tree complex wt.pdf 1.09MB
dual tree complex wt.pdf 1.09MB
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助 
 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜

 信息提交成功
信息提交成功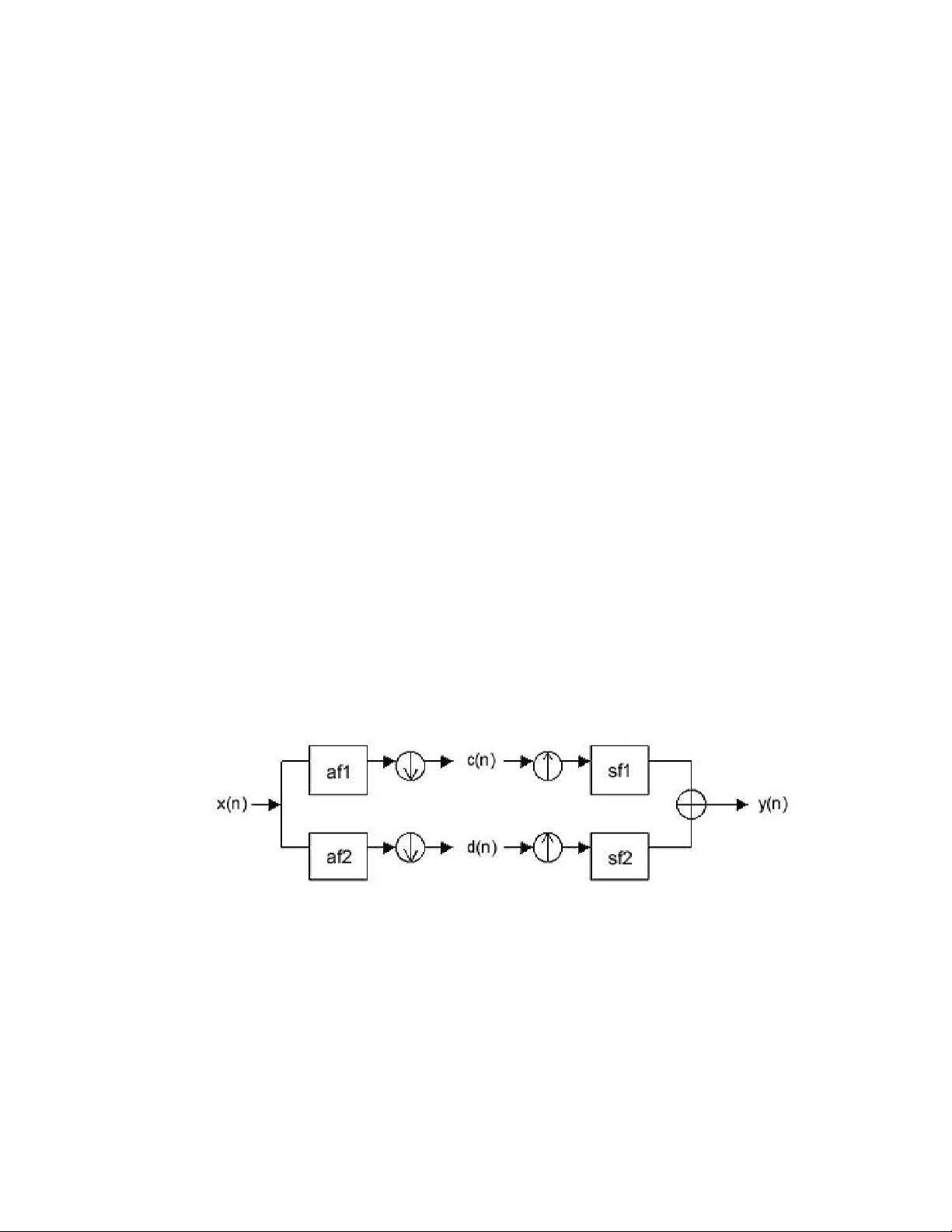
评论0