没有合适的资源?快使用搜索试试~ 我知道了~
我们在二维(2D)大型中心电荷保形场理论(CFT)中计算了表征长度为that的短间隔的密度降低矩阵的不相似性的各种量。 这些量包括Rényi熵,纠缠熵,相对熵,Jensen-Shannon发散以及Schatten 2范数和4范数。 我们采用扭曲算子的算子乘积展开法,并根据真空保形系列的贡献计算出这些量的短期扩展,直到ℓ9的数量级。 还给出了这些相异度量的形式形式以及从一般运营商的贡献中得出的Fisher信息度量。 作为结果的应用,我们使用这些相异性度量来比较激发态和热态,并通过显示它们在高温极限下的行为来检查本征态热化假设(ETH)。 这将有助于了解如何更精确地定义2D CFT中的ETH。 我们讨论了比较激发态和广义Gibbs系综热态的密度约简矩阵时,此处考虑的所有相异性度量消失的可能性。 我们还讨论了在2D大型中央电荷CFT中微规范集合热态的ETH,发现对于一个小型子系统它近似满足,而对于一个大型子系统则违反。
资源推荐
资源详情
资源评论

JHEP12(2017)073
Published for SISSA by Springer
Received: September 11, 2017
Accepted: December 4, 2017
Published: December 14, 2017
Dissimilarities of reduced density matrices and
eigenstate thermalization hypothesis
Song He,
a,b
Feng-Li Lin
c
and Jia-ju Zhang
d,e
a
Max Planck Institute for Gravitational Physics (Albert Einstein Institute),
Am M¨uhlenberg 1, 14476 Golm, Germany
b
CAS Key Laboratory of Theoretical Physics,
Institute of Theoretical Physics, Chinese Academy of Sciences,
55 Zhong Guan Cun East Road, Beijing 100190, China
c
Department of Physics, National Taiwan Normal University,
No. 88, Sec. 4, Ting-Chou Road, Taipei 11677, Taiwan
d
Dipartimento di Fisica, Universit`a degli Studi di Milano-Bicocca,
Piazza della Scienza 3, I-20126 Milano, Italy
e
INFN, Sezione di Milano-Bicocca,
Piazza della Scienza 3, I-20126 Milano, Italy
E-mail: hesong17@gmail.com, fengli.lin@gmail.com,
jiaju.zhang@mib.infn.it
Abstract: We calculate various quantities that characterize the dissimilarity of reduced
density matrices for a short interval of length ` in a two-dimensional (2D) large central
charge conformal field theory (CFT). These quantities include the R´enyi entropy, entangle-
ment entropy, relative entropy, Jensen-Shannon divergence, as well as the Schatten 2-norm
and 4-norm. We adopt the method of operator product expansion of twist operators, and
calculate the short interval expansion of these quantities up to order of `
9
for the contribu-
tions from the vacuum conformal family. The formal forms of these dissimilarity measures
and the derived Fisher information metric from contributions of general operators are also
given. As an application of the results, we use these dissimilarity measures to compare the
excited and thermal states, and examine the eigenstate thermalization hypothesis (ETH)
by showing how they behave in high temperature limit. This would help to understand how
ETH in 2D CFT can be defined more precisely. We discuss the possibility that all the dis-
similarity measures considered here vanish when comparing the reduced density matrices
of an excited state and a generalized Gibbs ensemble thermal state. We also discuss ETH
for a microcanonical ensemble thermal state in a 2D large central charge CFT, and find
that it is approximately satisfied for a small subsystem and violated for a large subsystem.
Keywords: AdS-CFT Correspondence, Conformal Field Theory, Field Theories in Lower
Dimensions
ArXiv ePrint: 1708.05090
Open Access,
c
The Authors.
Article funded by SCOAP
3
.
https://doi.org/10.1007/JHEP12(2017)073

JHEP12(2017)073
Contents
1 Introduction 1
2 Prescriptions of the method 2
2.1 CFT basics 3
2.2 OPE of twist operators 3
2.3 Partition function from twist operators 7
2.4 The n → 1 limit 10
2.5 R´enyi and entanglement entropies on various Riemann surfaces 11
3 Dissimilarities of reduced density matrices 13
3.1 Relative entropy 13
3.2 Jensen-Shannon divergence 15
3.3 Schatten 2-norm and 4-norm 16
4 ETH for canonical ensemble thermal state 16
5 ETH for GGE thermal state 19
6 ETH for microcanonical ensemble thermal state 21
7 Conclusion and discussion 24
A Relative entropy from modular Hamiltonian 24
B Contributions from general operators 26
C Collection of results in section 3 29
1 Introduction
Motivated by the eigenstate thermalization hypothesis (ETH) [1, 2] or its generalization,
the subsystem ETH [3, 4], it is important to characterize quantitatively the difference
between the excited state and the thermal state. One such characterization is to quantify
the difference between reduced density matrices over a local regions of these two states. This
is also an interesting question by itself in quantum information theory. For two-dimensional
(2D) conformal field theory (CFT), many other quantities of examining ETH have been
adopted, such as correlation functions [5, 6], entanglement entropy, R´enyi entropy, relative
entropy [3, 4, 7–10], trace square [11], etc. Due to the infinite number of degrees of freedom
in CFT, not every quantity is good for the use of examining the ETH [3, 4], unless its
behaviors for both excited and thermal states are known precisely.
– 1 –

JHEP12(2017)073
It was proposed in [12] to use correlation functions of twist operators to calculate the
R´enyi entropy in a 2D CFT, i.e., the partition function of the Riemann surface resulting
from the replica trick. When there is no compact form for these twist-operator correlation
functions, one can use operator product expansion (OPE) of twist operators to calculate
the short interval expansion of R´enyi entropy [13–17]. Following this method, in this paper
we will calculate various quantities which are just the sums of some partition functions,
and moreover can be used to characterize the dissimilarity of the reduced density matrices
of thermal and excited states, and other states on various Riemann surfaces.
Our results can be used to examine ETH. The ETH and subsystem ETH are originally
defined by comparing the highly excited state with the microcanonical ensemble thermal
state [1–4]. Motivated by [18, 19], as well as [5–8], we compare in [10] the excited state
with the canonical ensemble thermal state, and adopt the so-called weak ETH [18, 19].
In [10] the short-interval ` expansions of the entanglement entropies for the excited state
and canonical ensemble thermal state are calculated to order `
8
, and it was found that
their difference, which is just the relative entropy, is only suppressed by the powers of
large central charge c, instead of exponential suppression. In this paper we show that
there are similar behaviors for the Jensen-Shannon divergence and Schatten 2-norm. For
the more refined consideration, one should compare the excited state with the generalized
Gibbs ensemble (GGE) thermal state [20–25]. We will discuss the possibility that all the
dissimilarities considered in this paper vanish when comparing the reduced density matrices
of an excited state and a suitably defined GGE thermal state. As a by-product, we also
check ETH for the microcanonical ensemble thermal state with the dissimilarity measures
of comparing with the energy eigenstate.
The rest of this paper is arranged as follows. In section 2 we give prescriptions of the
method and show how to get the partition functions from OPE of twist operators. More-
over, in subsection 2.5 we apply the prescriptions to evaluate the R´enyi and entanglement
entropies. In section 3 we calculate the various dissimilarity measures between reduced
density matrices. In section 4 we apply our results to examine ETH for the canonical
ensemble thermal state. In section 5 we discuss the possible scenarios ETH for the GGE
thermal state. In section 6 we discuss ETH for a microcanonical ensemble thermal state
in a 2D large central charge CFT, and find that it is approximately satisfied for a small
subsystem and violated for a large subsystem. We conclude with discussion in section 7.
In appendix A we calculate the relative entropy from modular Hamiltonian as a consistent
check. In appendix B we consider the contributions from general operators, and get the
formal forms of the various dissimilarity measures and the Fisher information metric. Some
lengthy and not so enlightening results in section 3 are collected in appendix C.
2 Prescriptions of the method
In this section we first give the useful basics of the vacuum conformal family in two-
dimensional large central charge CFT and then show how we calculate the partition func-
tions on various Riemann surfaces using OPE of the twist operators.
– 2 –

JHEP12(2017)073
level 0 2 4 6 8 9
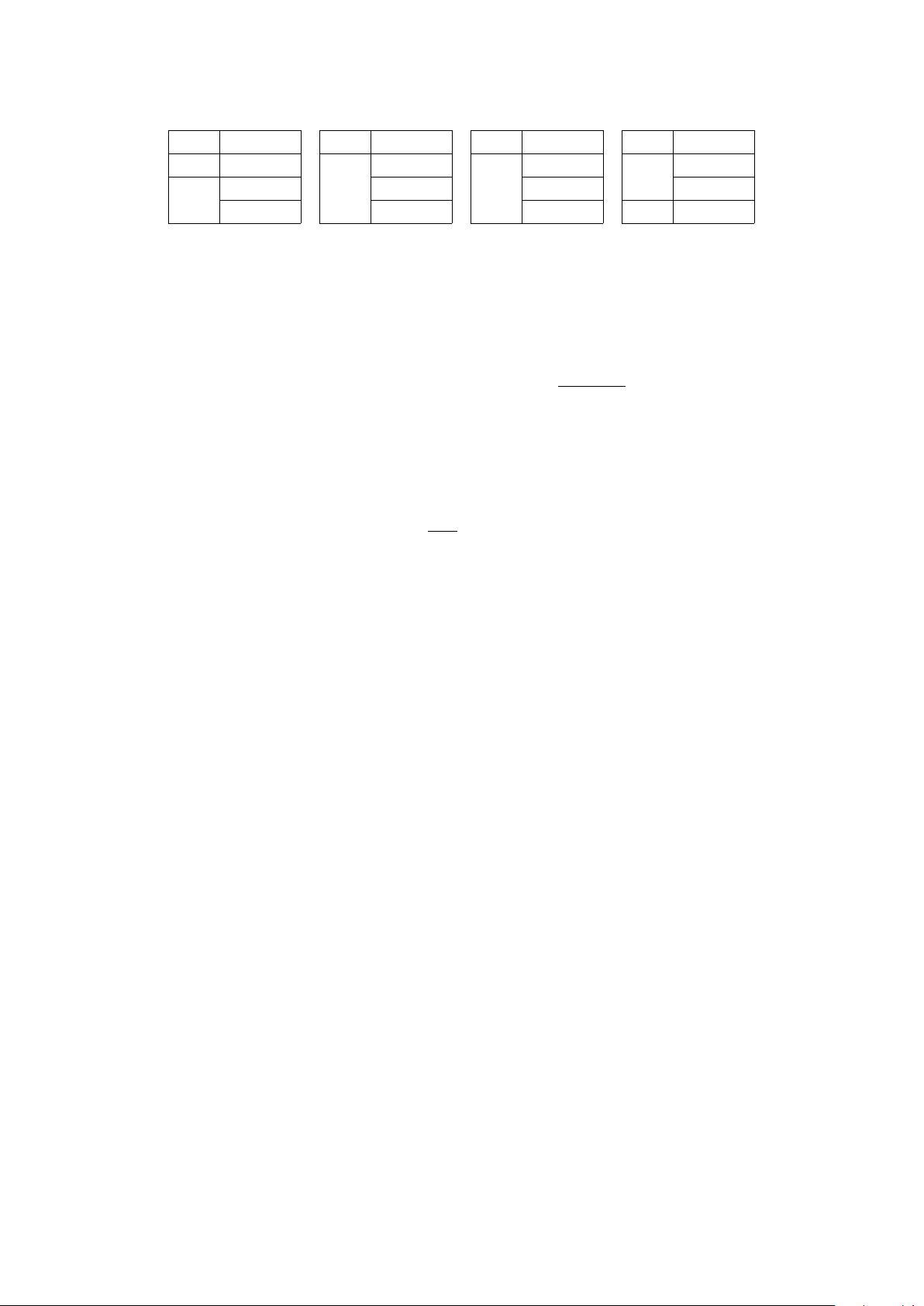
operator 1 T A B, D E, H, I J
Table 1. The holomorphic quasiprimary operators to level 9 in vacuum conformal family of a
two-dimensional large central charge CFT.
2.1 CFT basics
In this paper we only consider the contributions from the holomorphic sector of the vacuum
conformal family in a two-dimensional large central charge CFT, and the generalization to
antiholomorphic sector can be figured out easily. We need the quasiprimary operators to
level 9, i.e., T , A, B, D, E, H, I and J as shown in table 1. The definitions, normalization
factors, and conformal transformations of the quasiprimary operators up to level 8, as well
as some useful structure constants, can be found in [10, 16, 17, 26].
In this paper, we need the additional structure constants
C
T T E
=
20c(105c + 11)
63
, C
T T H
= C
T T I
= 0,
C
AAE
=
8c(5c + 22)(525c + 2419)
315
,
C
AAH
= −
8c(5c + 22)(8400c
2
+ 44575c − 6961)
125(105c + 11)
, (2.1)
C
AAI
=
3c(2c − 1)(3c + 46)(5c + 3)(5c + 22)(7c + 68)
2(1050c
2
+ 3305c − 251)
.
Furthermore, at level 9 we have the operator and its normalization
−iJ = (∂T (∂T ∂T ))−
6
5
(∂
2
T (∂T T ))+
4
15
(∂
3
T (T T ))−
1
10
(∂
4
T ∂T )+
1
100
(∂
5
T T )−
1
3150
∂
7
T,
α
J
=
224c(2c−1)(5c+22)
25
, (2.2)
with (XY) denoting normal ordering of two operators X and Y. Under a general conformal
transformation z → f(z) it transforms as
J(z) = f
09
J(f) + ··· +
c(2c − 1)(5c + 22)(4s
2
s
000
+ 15s
03
− 18ss
0
s
00
)
259200
, (2.3)
where s denotes the Schwarzian derivative
s(x) =
f
000
(x)
f
0
(x)
−
3
2
f
00
(x)
f
0
(x)
2
, (2.4)
and ··· represents the omitted terms that are proportional to T , A, B, D and their
derivatives.
2.2 OPE of twist operators
For one short interval A = [0, `] on a Riemann surface R, replica trick leads to a CFT on
an n-fold Riemann surface R
n
. The partition function on R
n
can be written as a two-point
– 3 –
JHEP12(2017)073
level operator level operator level operator level operator
2 T B, D E, H, I
8
T T A
4
A 6 T A 8 T B, T D T T T T
T T T T T AA 9 J
Table 2. The holomorphic nonidentity quasiprimary operators to be considered in this paper for
CFT
n
and up to level 9. We have omitted the replica indices and their constraints, which can be
easily figured out and can also be found in [26].
function of twist operators T and
˜
T in an n-fold CFT on R [12]
tr
A
ρ
n
A
= hT (`)
˜
T (0)i
R
, h
T
= h
˜
T
=
c(n
2
− 1)
24n
, (2.5)
and the n folds of the CFT, which we call CFT
n
, are independent except the connection
by the twist operators. In this paper we only consider Riemann surface R with trans-
lation symmetry, and so the one-point functions are all constants. Using OPE of twist
operators [13–17], we may get
hT (`)
˜
T (0)i
R
=
c
n
`
2h
σ
X
K
d
K
`
h
K
hΦ
K
(0)i
R
, (2.6)
and in the summation we only need to consider the quasiprimary operators Φ
K
in CFT
n
that are the direct products of the quasiprimary operators in different replicas of the
CFT. Only considering the contributions from the vacuum conformal family, we list the
quasiprimary operators in CFT
n
to level 9 in table 2. To level 8, the coefficients d
K
can
be found in [16, 26], and using the method in [15] and (2.2), (2.3) we can easily get
d
J
= 0. (2.7)
Interestingly, there is no contribution from level 9 operators, which consist of J only.
Each of the CFT
n
quasiprimary operator Φ
K
in (2.6) has the form
Φ
j
1
,j
2
,··· ,j
k
K
= X
j
1
1
X
j
2
2
···X
j
k
k
, (2.8)
with X
1
, X
2
, ···, X
k
being nonidentity quasiprimary operators in table 1 and there are also
some constraints for the k replica indices j
1
, j
2
, ··· , j
k
. We have the one-point functions
that are independent of the replica indices
hΦ
j
1
j
2
···j
k
K
i
R
= hX
1
i
R
hX
2
i
R
···hX
k
i
R
, (2.9)
and so we can define b
K
from the OPE coefficient d
j
1
j
2
···j
k
K
by summing over the replica
indices [17]
b
K
=
X
j
1
,j
2
,··· ,j
k
d
j
1
j
2
···j
k
K
with some constraints for 0 ≤ j
1
, j
2
, ··· , j
k
≤ n − 1. (2.10)
To level 8 the form of b
K
can be found in [10, 17], and from (2.7) we know
b
J
= 0. (2.11)
– 4 –
剩余37页未读,继续阅读
资源评论

weixin_38696196
- 粉丝: 9
- 资源: 872
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
- 学生成绩管理系统--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 足球社区管理系统pf-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学生成绩分析和弱项辅助系统设计--论文pf-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学生宿舍管理系统-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于VUE的农产品预售平台设计_v8557-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学生网上选课系统的设计与实现-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.rar
- 学生信息管理系统_06bg9--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于springboot框架的电影订票系统_wqc3k--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于Web的就业管理系统_w3209-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于web的多媒体素材管理系统--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于Web的电子产品销售系统设计与实现--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学生综合测评系统--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学生信息管理系统论文_20230609_075750--论文pf-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 学院个人信息管理系统_21j0r--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 基于web的铁路订票管理系统--论文-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
- 养老院管理系统_1w568-springboot毕业项目,适合计算机毕-设、实训项目、大作业学习.zip
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功