关于黑洞地平线附近希尔伯特空间对观察者的依赖性资源-CSDN文库

Open

Access

84 浏览量 2020-03-25 09:26:55 上传评论收藏 1.04MB PDF 举报

资源推荐

资源详情

资源评论

Prog. Theor. Exp. Phys. 2019, 023B01 (60 pages)

DOI: 10.1093/ptep/pty146

On the observer dependence of the Hilbert space

near the horizon of black holes

Kanato Goto

1,∗

and Yoichi Kazama

1,2,3

Institute of Physics, University of Tokyo, Komaba, Meguro-ku, Tokyo 153-8902 Japan

Research Center for Mathematical Physics, Rikkyo University, Toshima-ku, Tokyo 171-8501 Japan

Quantum Hadron Physics Laboratory, RIKEN Nishina Center, Wako 351-0198, Japan

∗

E-mail: kgoto@hep1.c.u-tokyo.ac.jp

Received July 23, 2018; Revised December 12, 2018; Accepted December 14, 2018; Published February 11, 2019

...................................................................................................................

One of the pronounced characteristics of gravity, distinct from other interactions, is that there

are no local observables which are independent of the choice of the spacetime coordinates. This

property acquires crucial importance in the quantum domain in that the structure of the Hilbert

space pertinent to different observers can be drastically different. Such intriguing phenomena as

Hawking radiation and the Unruh effect are all rooted in this feature. As in these examples, the

quantum effect due to such observer dependence is most conspicuous in the presence of an event

horizon and there are still many questions to be clariﬁed in such a situation. In this paper we

perform a comprehensive and explicit study of the observer dependence of the quantum Hilbert

space of a massless scalar ﬁeld in the vicinity of the horizon of Schwarzschild black holes in

four dimensions, both in the eternal (two-sided) case and in the physical (one-sided) case created

by collapsing matter. Speciﬁcally, we compare and relate the Hilbert spaces of three types of

observers, namely (i) the freely falling observer, (ii) the observer who stays at a ﬁxed proper

distance outside of the horizon, and (iii) the natural observer inside of the horizon analytically

continued from outside. The concrete results we obtain have a number of important implications

on black hole complementarity pertinent to the quantum equivalence principle and the related

ﬁrewall phenomenon, on the number of degrees of freedom seen by each type of observer, and

on the “thermal-type” spectrum of particles realized in a pure state.

...................................................................................................................

Subject Index B22, B39

1. Introduction

A quantum black hole is a fascinating but as yet an abstruse object. Recent endeavors to identify

it in a suitable class of conformal ﬁeld theories (CFTs) in the AdS/CFT context [1–3][4–7]orby

an ingenious model such as the one proposed by Sachdev, Ye, and Kitaev [8–10] have seen only

a glimpse of it, to say the most. Unfortunately, string theory, at the present stage of development,

does not seem to give us a useful clue either. This difﬁculty is naturally expected since an object

whose proﬁle ﬂuctuates by quantum self-interaction would be hard to capture. We must continue

our struggle to ﬁnd an effective means to characterize it more precisely.

Although the quantization of a black hole itself is still a formidable task, some analyses of quantum

effects around a (semi-)classical black hole have been performed since a long time ago, and they

have already uncovered various intriguing phenomena. Among them are the celebrated Hawking

radiation [11][12–14] and the closely related Unruh effect [15–17]. These effects revealed the non-

trivial features of the quantization in curved spacetimes, in particular in those with event horizons.

This is an Open Access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0/),

which permits unrestricted reuse, distribution, and reproduction in any medium, provided the original work is properly cited.

Funded by SCOAP

PTEP 2019, 023B01 K. Goto and Y. Kazama

At the same time, they brought out new puzzles of deep nature, such as the problem of information

loss, the ﬁnal fate of an evaporating black hole, and so on.

More recently, a further unexpected quantum effect in the black hole environment was argued to

occur, namely that a freely falling observer encounters excitations of high-energy quanta, termed

a “ﬁrewall,” as he/she crosses the event horizon of a black hole [18,19][20]. This is clearly at

odds with the equivalence principle, which is one of the foundations of classical general relativity.

An enormous number of papers have appeared since then, both for and against the assertion.

The

various arguments presented have all been rather indirect, however, making use of the properties

of the entanglement entropy, application of the no-cloning theorem, use of information-theoretic

arguments, etc.

At the bottom of these phenomena lies the strong dependence of the quantization on the frame of

observers, which is one of the most characteristic features of quantum gravity. This is particularly

crucial when the spacetime of interest contains event horizons as seen by some observers, and leads

to the notion of black hole complementarity [21].

The main aim of the present work is to investigate this observer dependence in some physically

important situations as explicitly as possible to gain some ﬁrm and direct understanding of the

phenomena rooted in this feature. For this purpose, we shall study the quantization of a massless

scalar ﬁeld in the vicinity of the horizon of the Schwarzschild black hole in four dimensions as

perfomed by three typical observers. They are (i) the freely falling observer crossing the horizon,

(ii) the stationary observer hovering at a ﬁxed proper distance outside the horizon (i.e. one under

constant acceleration), and (iii) the natural analytically continued observer inside the horizon.

Such an investigation, we believe, will be important for at least two reasons. One is that we will

deal directly with the states of the scalar ﬁelds as seen by different observers and will not rely on

any indirect arguments alluded to above. This makes the interpretation of the outcome of our study

quite transparent (up to certain approximations that we must make for computation). Another role

of our investigation is that the concrete result we obtain should serve as the properties of quantum

ﬁelds in the background of a black hole, which should be compared, in the semi-classical regime, to

the results to be obtained by other means of investigation, notably and hopefully by the AdS/CFT

duality.

For some progress, and intriguing proposals in the related directions, see Refs. [22–29].

This is important since, as far as we are aware, there has not been a serious attempt to understand

how the observer dependence is described in the context of AdS/CFT duality.

We will perform our study both for the case of a two-sided eternal Schwarzschild black hole and for

that of a one-sided physical black hole modeled by a simple Vaidya metric produced by collapsing

matter or radiation at the speed of light

[30–32]. What makes such an investigation feasible explicitly

is the well-known fact that near the horizon of the Schwarzschild black hole (roughly within the

Schwarzschild radius from the horizon; see Sect. 3.1 for a more precise estimate) there exists a

coordinate frame in which the metric takes the form of the ﬂat four-dimensional Minkowski spacetime

1,3

. Thus, one can make use of the knowledge of the quantization in the ﬂat space for observers

It is practically impossible to list all such papers on this subject. We refer the reader to those citing the

basic papers, Refs. [18,19].

As far as the vicinity of the horizon is concerned, the Schwarzschild black hole and the AdS black hole

have the same structure.

Actually, we shall make an inﬁnitesimal regularization to make the trajectory of the matter slightly timelike

in order to avoid a certain singularity.

2/60

PTEP 2019, 023B01 K. Goto and Y. Kazama

corresponding to the various Rindler frames. As this will serve as the platform upon which we

develop our picture and computational methods for the black hole cases, we will give, in Sect. 2, a

review of this knowledge together with some further new information about the relations between

the quantizations by the three aforementioned observers.

In making use of this ﬂat space approximation to the near-horizon region of a black hole, an impor-

tant care must be taken, however. Although the scalar ﬁeld and its canonical conjugate momentum

are locally well-approximated by those in the ﬂat space for the region of our interest, and hence

the canonical quantization can be performed without any problem, as we try to extract the physical

modes that create and annihilate the quantum states, such local knowledge is not enough in general.

This is because the notion of a quantum state requires the global information of the wave function.

Technically, this is reﬂected in the fact that the orthogonality relation needed for the extraction of

the mode is expressed by an integral over the entire spacelike surface at equal time, and depending

on the region of interest such a surface may not be totally contained within the region where the ﬂat

space approximation is valid.

One such problem, which, however, can be easily dealt with, stems from the simple fact that

the approximation by the four-dimensional ﬂat space includes that of the spherical surface of the

horizon by a tangential plane around a point. Clearly, since the physical modes of the scalar ﬁeld

should better be classiﬁed by the angular momentum, not by the linear momentum, we shall use

1,1

× S

instead of M

1,3

as the more accurately approximated spacetime, where R

1,1

stands for

a portion of two-dimensional ﬂat spacetime realized near the horizon and

is the sphere at the

Schwarzschild radius. Various formulas reviewed and/or developed in Sect. 2 for M

1,3

can be readily

transplanted to this case by replacing the plane waves by spherical harmonics.

The problem pointed out above of the extraction of the modes within the ﬂat region is much

more non-trivial in the near-horizon region of

1,1

, since the ﬂat region which extends to inﬁnity

is only along the direction of the light cone. The problem with this situation is that the use of the

trajectory along the light cone leads to the quantization of a chiral boson, which is known to be

notoriously complicated. In addition, such a trajectory is not connected by a Lorentz transformation

to the trajectory of a general observer, which is timelike. This problem is particularly severe when

we deal with the one-sided black hole produced by a massless shock wave, the effect of which will

be treated by the imposition of an effective Dirichlet boundary condition on the scalar ﬁeld along the

trajectory of the shock wave. To solve this problem, we have made a careful regularization of taking

the trajectory of the shock wave to be slightly timelike.

Then we are able to treat the quantization

for the observers freely falling with arbitrary velocity by making a suitable Lorentz transformation.

Such a proper anaysis has not been performed in the literature and this allowed us to obtain ﬁrm

results for the question of major interest.

Although we cannot summarize here all the results on how the different observers see their quanta

and how they are related, let us list two that are of obvious interest:

◦

Under the assumption that the metric of the interior of a physical Schwarzschild black hole, in

particular one large enough so that the curvature at the horizon is very small, can be described

by a Vaidya-type solution, our results indicate that the equivalence principle still holds quantum

mechanically near the horizon of the black hole, and the freely falling observer ﬁnds no surprise

as he/she goes through the horizon.

Evidently this corresponds to the case of slightly massive falling matter, which is physically reasonable.

3/60

PTEP 2019, 023B01 K. Goto and Y. Kazama

◦

For a physical (one-sided) black hole, the vacuum

0

−

for the freely falling observer is a pure

state which is not the same as the usual Minkowski vacuum |0

. Nevertheless, the expectation

value of the number operator for the observer in the frame of the right Rindler wedge in

0

−

has an Unruh-like distribution, which contains a “thermal” factor together with another

portion depending on the assumed interaction between the scalar ﬁeld and the collapsing matter,

effectively expressed as a boundary condition. This is in contrast to the case of the two-sided

eternal black hole, where tracing out of the modes of the left Rindler wedge must be performed

and the resultant mixed state density matrix produces the usual purely thermal form of the Unruh

distribution. The effect for the physical black hole occuring in the pure state described above is

essentially of the same origin as the Hawking radiation seen by the asymptotic observer, who

is a Rindler observer.

The plan of the rest of the paper is as follows: In Sect. 2, we begin by describing the quantization

of a massless scalar ﬁeld in four-dimensional ﬂat Minkowski space from the point of view of various

observers, and provide explicit relations between them. Although this section is mostly a review,

we also derive some useful relations that have not been discussed in the literature. This includes

the construction of the explicit unitary transformation between the Minkowski mode operators and

those of the future Rindler wedge, and how the Poincaré algebra is realized in various wedges. Next,

in Sect. 3, this knowledge about the quantization in ﬂat spacetime will be utilized to discuss how

the scalar ﬁeld is quantized by various observers in the vicinity of the event horizon of a two-sided

Schwarzschild black hole, which by a suitable choice of coordinates can be approximated by part

1,1

× S

. In Sect. 4, we study the similar problem in the case of a Vaidya model of the physical

one-sided black hole that is produced by a collapse of matter with inﬁnitesimal mass, introduced as

a regularization. The effect of this collapse is treated as an effective boundary condition on the scalar

ﬁeld along a slightly timelike trajectory of such a shock wave. Even though we focus on the ﬂat region

near the horizon, the quantum states, which depend on the global situation, show different properties

as compared with the two-sided case studied in Sect. 3. In Sect. 5, we disucss the implications

of the results obtained in the previous sections on some important questions, such as the quantum

equivalence principle, the ﬁrewall phenomenon, and the Unruh effect near the horizon. Finally, in

Sect. 6, after summarizing the results, we re-emphasize that the effect of the observer dependence

of quantization is one of the most crucial characteristics of any theory of quantum gravity, and it

should be seriously investigated, in particular, in the framework of the AdS/CFT approach. Several

appendices are provided to give further useful details of the formulas and calculations discussed in

the main text.

2. Quantization of a scalar ﬁeld in the Rindler wedges and the degenerate Kasner

universes

We begin by describing the quantization of a massless

scalar ﬁeld in the four-dimensional Minkowski

space from the standpoint of uniformly accelerated Rindler observers for the right and the left wedges

and W

, and their appropriate analytic continuations for the future and the past wedges W

and

, which can be identiﬁed as degenerate Kasner universes. In Fig. 1, we draw the trajectories of

the corresponding observers and the equal-time slices in each wedge.

The vacuum referred to here will be explained in Sect. 4.2.3.

For related work, though in a different setting, see Ref. [33].

The massive case can be treated in an entirely similar manner.

4/60

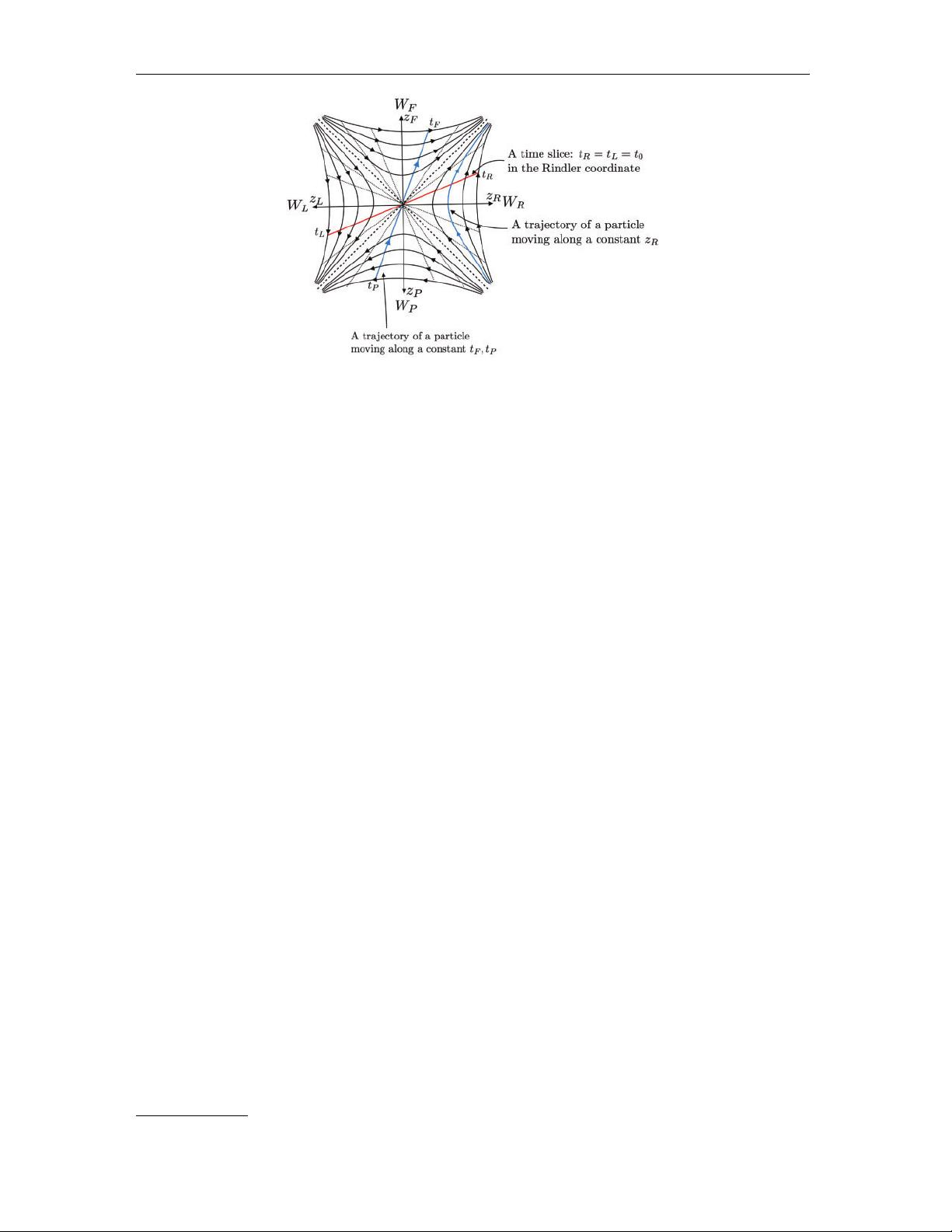
PTEP 2019, 023B01 K. Goto and Y. Kazama

Fig. 1. Trajectories and equal-time slices of the Rindler observers in various wedges. The boundaries of the

wedges W

, and W

are shown by dotted lines. The blue arrowed lines represent the trajectories of a

particle, while the red line is a typical time slice at t

= t

for W

and W

The subject of quantization by Rindler observers has a long history [17,34–36] and hence the

content of this section is largely a review.

However, part of our exposition supplements the descrip-

tion in the existing literature by providing some clarifying details and new relations. The results of

this section will serve as the foundation upon which to discuss the observer-dependent quantization

around the horizon of Schwarzschild black holes, both eternal (two-sided) and physical (one-sided),

as will be performed in Sect. 3.

2.1. Relation between Minkowski and Rindler coordinates

Before getting to the quantization of a scalar ﬁeld, we need to describe the relationship between the

Minkowski coordinates and the Rindler coordinates in various wedges.

The d-dimensional Minkowski metric is described in the usual Cartesian coordinate as

=−(dt

)

+ (dx

)

d−1



i=2

(dx

)

. (2.1)

Since we will be mostly concerned with the ﬁrst two coordinates, and the roles of the rest of the

d − 2 coordinates are essentially the same, hereafter we will deal with the four-dimensional case,

i.e. d = 4.

As for the Rindler coordinates, we begin with the one in the right wedge W

shown in Fig. 1.As

is well known, it is related to the coordinates of the observer who is acclerated in the positive x

direction with a uniform acceleration. The trajectory of the observer in the (t

, x

) Minkowski plane

with a value of acceleration κ(>0) is given by

)

− (t

)

= (1/κ)

= z

. (2.2)

Here, the symbol z

is introduced as a variable, meaning that different values of z

describe different

trajectories. Thus the Rindler coordinate system is spanned by the proper time τ

of the observer

For a review article closely related to this section, see Ref. [37].

5/60

剩余59页未读，继续阅读

评论收藏

内容反馈

weixin_38641366

粉丝: 4
资源: 893

关于黑洞地平线附近希尔伯特空间对观察者的依赖性

沿黑洞地平线发射的光子的红移

自发破碎的渐近对称性和对地平线动力学的有效作用

一眼瞥见黑洞地平线

极端Kerr-Taub-NUT黑洞近地平线区域的电磁辐射

重力作用对近地平线黑洞极端的熵函数

一般静态球对称黑洞附近的循环和非斜流

最亮的黑洞的地平线

Mac地平线---驱动文件.part01.rar

kodi20皮肤，地平线2.0完整安装包（Arctic Horizon 2.0 ）

地平线DVD导航网络营销推广策略.pptx

摄动理论在地平线附近的失败：Rindler的例子

使用事件地平线望远镜极化测量探测轴

黑洞视界附近的能量和信息

【俯视地平线,阅读答案】 俯瞰地平线.docx

隧穿范式中具有修正色散关系的黑洞辐射：静态框架

地平线：架构创新BPU 算法 芯片 云一体化.pdf

《地平线》课件.ppt

《穿过地平线》教学设计.pdf

IBM“绿色地平线”让地球家园更健康

《地平线 零之曙光》的实时体积云景

罗兰贝格地平线_智能座舱发展趋势白皮书.pdf

弦论中的黑洞内部

量子从奇点跳到黑洞外面

AdS / CFT中用于黑洞的砖墙

极端黑洞摄动的定标和普遍性

黑洞波功能的分支不需要包含防火墙

最新资源

【俯视地平线,阅读答案】俯瞰地平线.docx

地平线：架构创新BPU 算法芯片云一体化.pdf

《地平线零之曙光》的实时体积云景