没有合适的资源?快使用搜索试试~ 我知道了~
资源推荐
资源详情
资源评论

228
The
UMAP
Journal
26.3
(2005)
For
Whom
the
Booth
Tolls
Brian
Camley
Bradley
Klingenberg
Pascal
Getreuer
University
of
Colorado
Boulder,
CO
Advisor:
Anne
Dougherty
Summary
We
model
traffic
near
a
toll
plaza
with
a
combination
of
queueing
theory
and
cellular
automata
in
order
to
determine
the
optimum
number
of
tollbooths.
We
assume
that
cars
arrive
at the
toll
plaza in
a
Poisson
process,
and that
the
probability
of
leaving
the
tollbooth
is
memoryless.
This
allows
us
to
completely
and
analytically describe
the
accumulation
of
cars
waiting
for
open
tollbooths
as
an
MIMIn
queue.
We
then
use
a
modified
Nagel-Schreckenberg
(NS)
cellu-
lar automata
scheme
to
model
both
the
cars
waiting
for tollbooths
and
the
cars
merging
onto
the
highway.
The
models
offer
results
that
are
strikingly
consis-
tent,
which
serves
to
validate
the
conclusions
drawn
from
the
simulation.
We
use
our
NS
model
to
measure
the
average
wait
time
at the
toll
plaza.
From
this
we
demonstrate
a
general
method
for
choosing
the
number
of
toll-
booths
to
minimize the
wait
time.
For
a
2-lane
highway,
the
optimal
number
of
booths
is
4;
for
a 3-lane
highway,
it
is
6.
For
larger
numbers
of
lanes,
the result
depends
on
the arrival
rate
of
the
traffic.
The
consistency
of
our
model
with
a
variety
of
theory and
experiment
sug-
gests
that
it
is
accurate
and robust.
There
is
a
high
degree
of
agreement
between
the
queueing
theory
results
and
the
corresponding
NS
results.
Special
cases
of
our
NS
results
are
confirmed
by
empirical
data
from
the literature.
In
addition,
changing
the
distribution
of
the
tollbooth
wait
time
and
changing
the
probabil-
ity
of
random
braking
does
not
significantly alter
the
recommendations.
This
presents
a
compelling
validation
of
our
models
and
general approach.
The
text of
this
paper
appears
on
pp.
373-390.
The
UMAPJournal
26
(3)
(2005) 228.
@Copyright
2005
by
COMAP,
Inc.
All
rights
reserved.
Permission
to
make
digital
or
hard
copies
of
part
or
all
of
this
work
for
personal
or
classroom
use
is
granted without
fee
provided
that
copies
are
not
made
or
distributed
for
profit
or
commercial
advantage
and that
copies
bear this
notice.
Abstracting
with
credit
is
permitted,
but
copyrights
for components
of
this
work
owned
by
others
than
COMAP
must
be
honored.
To
copy otherwise,
to
republish,
to
post
on
servers,
or
to
redistribute
to
lists
requires
prior
permission
from
COMAP.
更多数学建模资料请关注微店店铺“数学建模学习交流”
https://k.weidian.com/RHO6PSpA

For
Whom the
Booth
Tolls
373
For
Whom
the
Booth
Tolls
Brian
Camley
Bradley
Klingenberg
Pascal
Getreuer
University
of Colorado
Boulder,
CO
Advisor:
Anne
Dougherty
Summary
We
model
traffic
near
a
toll
plaza
with
a
combination
of
queueing
theory
and
cellular
automata
in
order
to
determine
the
optimum
number
of tollbooths.
We
assume
that
cars
arrive
at the
toll
plaza
in
a
Poisson
process,
and
that
the
probability
of
leaving
the tollbooth
is
memoryless.
This
allows
us
to
completely
and
analytically
describe
the
accumulation
of
cars
waiting
for
open
tollbooths
as
an
MIMjn
queue.
We
then
use
a
modified
Nagel-Schreckenberg
(NS)
cellu-
lar
automata
scheme
to
model
both
the
cars
waiting
for
tollbooths
and
the
cars
merging
onto
the
highway.
The
models
offer
results
that
are
strikingly
consis-
tent,
which
serves
to
validate
the
conclusions
drawn
from
the
simulation.
We
use
our
NS
model
to
measure
the
average
wait
time
at the
toll
plaza.
From
this
we
demonstrate
a
general
method
for choosing
the
number
of
toll-
booths
to
minimize
the
wait
time. For
a
2-lane
highway,
the
optimal
number
of
booths
is
4;
for
a 3-lane
highway,
it
is
6.
For
larger
numbers
of
lanes,
the
result
depends
on
the
arrival
rate
of
the
traffic.
The
consistency
of
our
model
with
a
variety of theory
and
experiment
sug-
gests
that
it
is
accurate
and
robust.
There
is
a
high
degree
of
agreement
between
the
queueing
theory results
and
the
corresponding
NS
results.
Special
cases
of
our
NS
results
are
confirmed
by
empirical
data
from
the
literature.
In addition,
changing the
distribution
of
the
tollbooth
wait
time
and
changing
the
probabil-
ity
of
random
braking
does
not
significantly alter
the recommendations.
This
presents
a
compelling
validation
of
our
models
and
general
approach.
The
UMAPJournal26
(3)
(2005)
373-389.
@Copyright
2005
by
COMAP, Inc.
All
rights reserved.
Permission
to
make
digital
or
hard
copies
of
part
or
all
of
this
work
for
personal
or
classroom
use
is
granted
without
fee
provided
that
copies
are
not made
or
distributed
for
profit or
commercial
advantage
and
that
copies
bear
this
notice.
Abstracting
with
credit
is
permitted,
but
copyrights
for
components
of
this
work
owned
by
others
than
COMAP
must
be
honored.
To
copy
otherwise,
to
republish,
to
post
on
servers, or
to
redistribute
to
lists
requires
prior
permission
from
COMAP.

374
The
UMAPJournal
26.3
(2005)
Introduction
A
toll
plaza
creates
slowdowns
in
two
ways:
*
If
there
are
not
enough
tollbooths,
queues
form.
*
If
there
are
too
many
tollbooths,
a
traffic
jam
ensues
when
cars
merge
back
onto
the
narrower
highway.
We
use
queueing
theory
to
predict
how long
vehicles will
have
to
wait
before
they
can be
served
by
a
tollbooth. Using
cellular
automata
to
model
individual
cars,
we
confirm
this
prediction
of
wait
time.
This
vehicle-level
model
is
used
to
predict
how
traffic
merges after
leaving
the toll
plaza.
Initial
Assumptions
"*
The
optimal
system
minimizes
average
wait
time.
We
do
not
consider
the
cost of
operating
tollbooths.
"*
Cars
arrive at
the
toll
plaza
uniformly
in
time
(the
interarrival distribution
is
exponential
with
rate
A).
We
can
consider
rush
hour
by
varying
the
arrival
rate
A.
"*
Cars
have
a
wait
time
at
the
tollbooth
that
is
memoryless
(exponential
distribution
with
rate
f).
This
assumption
is
confirmed
by
the
study
of
tollbooths
by
Hare
[1963].
"o
Cars
are
indistinguishable.
All
cars
have
the same
length
and
the same
maximum
speed.
"*
The
toll
plazas
are
not
near on-ramps
or
exits.
We
do
not
consider
the
possibility
of
additional
cars
merging,
only
those
that
were
already on
the
main road.
"*
Two-way
highways
are
equivalent
to
two
independent
highways.
We
consider only
divided
highways.
Delays
Due
to
Too
Few
Tollbooths
Tollbooths
As
an
MIMIn
Queue
As
a
vehicle
approaches the
toll
plaza,
it
has
a
choice
of
n
tollbooths
for
service.
Cars
tend
toward
the
shortest queue
available.
We
simplify
this
be-
havior
by
supposing
that
all
vehicles
form
a single
queue,
and that
the
next
car
in
line
enters
a
tollbooth
as
soon
as
one
of
the
n
booths
becomes
available.
A
For
Whom the
Booth
Tolls
375
real
system
would
be
less
efficient,
and
therefore
we
expect
longer
times
in
a
more
detailed
simulation.
We
assume
that
vehicles
arrive
uniformly
distributed
in time.
We
addi-
tionally
suppose
that
the
length
of
service
time
is
exponentially
distributed
as
in
Hare
[1963].
This
class
of
model
is
called
a
memoryless
arrivals,
memory)ess
service
times,
n-server
or
"MI
M]n
"
queue
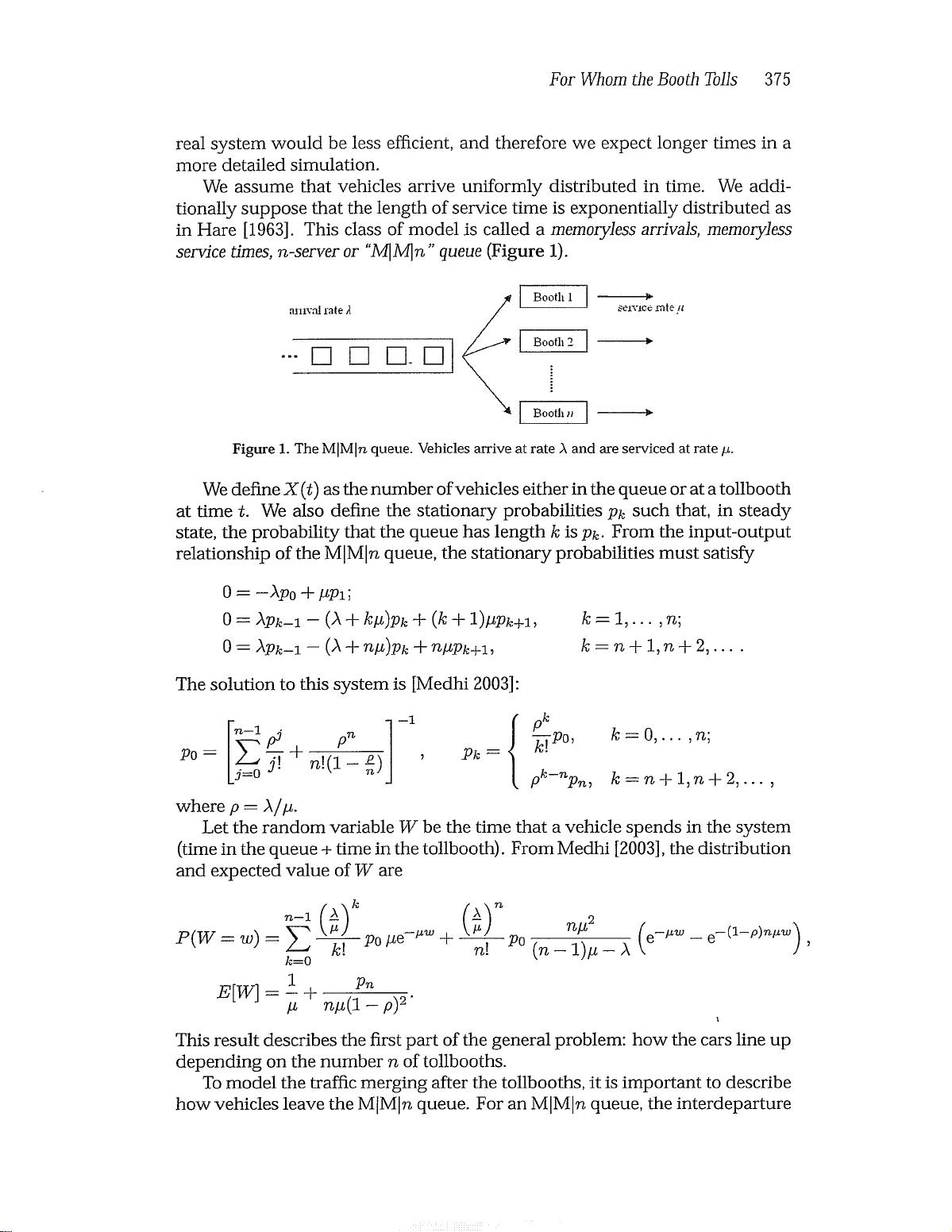
(Figure
1).
'11wl
ra(e
A
4-4Cerxice
n,te
P
Figure
1.
The
MIMIn
queue.
Vehicles
arrive
at
rate
A
and
are
serviced
at
rate
p.
We
define
X
(t)
as
the
number
of
vehicles
either
in
the
queue
or
at
a
tollbooth
at
time
t.
We
also
define
the
stationary
probabilities
Pk
such
that,
in
steady
state,
the
probability
that
the
queue
has
length
k
is
Pk-
From
the
input-output
relationship
of
the
MIMIn
queue,
the
stationary
probabilities
must
satisfy
0
=
-Apo
+
ppl;
0
=
APk-1
-- (A
+
kPL)Pk
+
(k
+
1)tLPk+1,
k
=l,...,
n,
0
=
APk-1
-
(A
+
np)pk
+
nppk+l,
k-=n+1,n+2,...
The
solution
to
this
system
is
[Medhi
2003]:
pP
[
pn
-po,
k
=
0,...],
n;
n=
p
k-npn,
k=n+l,n+2,...,
where
p
--
A/M.
Let
the
random
variable
W
be
the
time
that
a
vehicle
spends
in
the
system
(time
in
the
queue
+
time
in
the
tollbooth).
From
Medhi
[2003],
the
distribution
and
expected
value
of
W
are
P(W
=w)
ni
(A)
ope-
+
Po_
nyu
2
e-t
-
e(1-)/,
1
p____
E[Tq
I
+-+
This
result
describes
the
first
part
of the
general
problem:
how
the
cars
line
up
depending
on
the
number
n
of
tollbooths.
To
model
the
traffic
merging
after
the
tollbooths,
it
is
important
to
describe
how
vehicles
leave
the
MIMIn
queue.
For
an
MIMIn
queue,
the
interdeparture
剩余18页未读,继续阅读
资源评论

matlab科研社
- 粉丝: 2w+
- 资源: 2204
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
- 浮标、船、人检测10-YOLO(v7至v11)、COCO、VOC数据集合集.rar
- 四川采矿场安全管理规定.docx
- 采矿场职业卫生及职业危害管理规定.docx
- 四川采矿场安全管理制度手册.docx
- 四川采矿场安全教育培训管理规定.docx
- 四川采矿场安全生产奖惩管理规定.docx
- 四川采矿场安全生产目标管理制度.docx
- 四川采矿场安全技术措施审批制度.docx
- 四川采矿场安全检查管理规定.docx
- 四川采矿场班组安全建设管理规定.docx
- 四川采矿场顶板分级管理制度.docx
- 四川采矿场罐笼升降人员物料管理规定.docx
- 四川采矿场供配电系统安全管理规定.docx
- 四川采矿场防排水系统安全管理规定.docx
- 四川采矿场生产安全事故管理制度.docx
- 四川采矿场领导带班下井管理制度.docx
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功