
http://www.paper.edu.cn
-1-
Dynamic Analysis of Bernoulli–Euler Beam on Two-Parameter
Foundation Subjected to Moving Harmonic Load
Cao Changyong, Zhong Yang, Li Mingliang
Department of Civil Engineering, Dalian University of Technology, Dalian, P. R. China (116024)
E-mail:changyongcao@163.com
Abstract
The dynamic response of an infinite Bernoulli–Euler beam, placed on a two- parameter foundation and
subjected to a moving harmonic load, has been investigated in the paper. The double Fourier transform
technique has been employed to reduce the governing partial equation to algebraic equation and the inverse
Fourier transform is utilized to get the analytical solution in integral form. The closed-form analytical
solution of the problem is obtained by residue theorem and theory of complex analysis. Detailed analyses
based on a practical concrete beam are performed to research the effect of various parameters on the dynamic
response of the beam. Numerical results show that the maximum deflection of the beam resting on
two-parameter foundation increases slightly with the increasing of load velocity and decreases with the
increasing of the shear modulus of subgrade.
Keywords: Beam; Dynamic response; Two-parameter foundation; Moving harmonic loads.
1. Introduction
The vibration, buckling and bending problems of beams on elastic foundation have been investigated by
many researchers working on pavement, rail track and structural foundation analysis and design [1-4]. For
practical application, a Bernoulli–Euler beam resting on a soil foundation can be conveniently used to
represent the railway track and the highway pavement. The governing differential equation of the system can
be obtained by considering the dynamic equilibrium of beam resting on elastic ground and undergoing
transverse vibrations. The elastic foundation has been usually modeled by a Winkler foundation for
mathematical simplicity [5-8]. This kind of model proposed by Winkler, consisting of a system of mutually
independent linear springs, is assumed that the deflection of foundation at any point on the surface is directly
proportional to the stress and there is no interaction between the lateral springs. Therefore, it does not
accurately represent the continuous characteristic of many practical foundations. To find a more physically
close and mathematically simple foundation model, various two-parameter foundations were proposed by
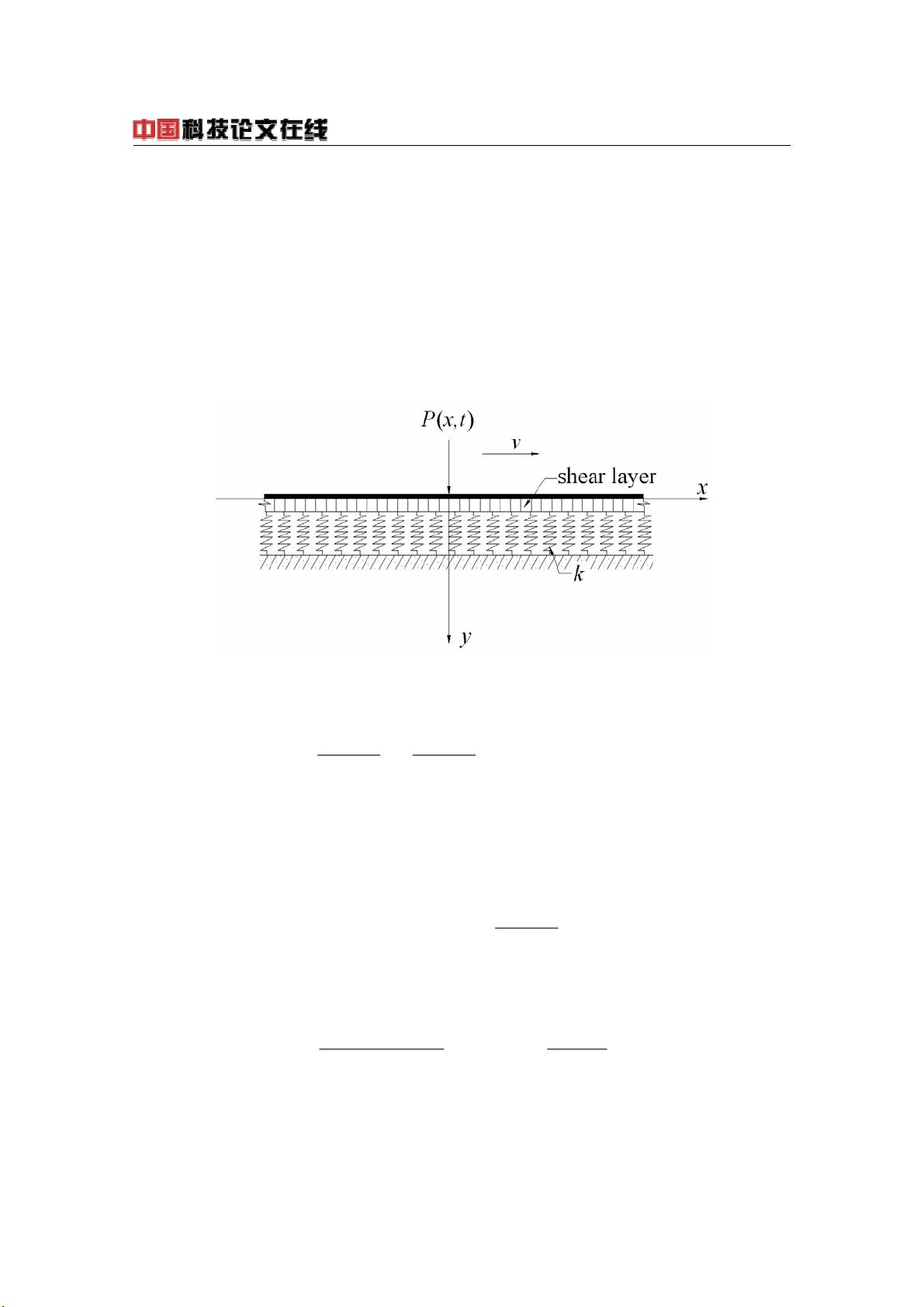
researchers [9-13]. In this paper, Pasternak’s model has been employed to represent the soil foundation, in
which shear interaction between the springs is considered. It is accomplished by connecting the spring
elements to a layer of incompressible vertical elements which develop transverse shear deformation only (Fig.
1).
Up to now, the cases of beams on two-parameter foundations subjected to moving loads have received less
attention, probably due to the model complexity and the difficulties in the estimation of the parameter values.
Results of the analytical solution of the problem were published in literatures [14-17]. Eisenberger and
Clastornik [18] solved the problem of a beam on a two-parameter elastic foundation and obtained the solution
by means of infinitive polynomial series. Yokoyama [19] studied the vibrations of a beam-column on
two-parameter elastic foundation and presented a finite element procedure for analyzing the flexural
vibrations. Eisenberger [20] presented an exact method for the vibration analysis of beams rested on one- and
two-parameter elastic foundations using the well-known power series solutions of differential equations with
variable coefficients.
In the present analysis, the dynamic response of a beam subjected to a moving harmonic load is investigated.
The beam is modeled as an infinite Bernoulli–Euler beam with constant cross-section. The soil is represented
by a two-parameter foundation model. Both beam and foundation are assumed to be homogeneous and


 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助 
 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜

 信息提交成功
信息提交成功