没有合适的资源?快使用搜索试试~ 我知道了~
温馨提示
We review some of the recent advances in the development of subwavelength plasmonic devices for manipulating light at the nanoscale, drawing examples from our own work in metal-dielectric-metal (MDM) plasmonic waveguide devices. We introduce bends, splitters, and mode converters for MDM waveguides with no additional loss. We also demonstrate that optical gain provides a mechanism for on/off switching in MDM plasmonic waveguides. Highly efficient compact couplers between dielectric waveguides and
资源推荐
资源详情
资源评论

302 CHINESE OPTICS LETTERS / Vol. 7, No. 4 / April 10, 2009
Metal-dielectric-metal plasmonic waveguide devices for
manipulating light at the nanoscale
Invi ted Paper
Georgios Veronis
1∗
, Zongfu Yu
2
, S¸¨ukr¨u Ekin Kocaba¸s
2
, David A. B. Miller
2
,
Mark L. Brongersma
3
, and Shanhui Fan
2
1
Department of Electrical and Computer Engineering and Center for Computation
and Technology, Louisiana State University, Baton Rouge, LA 70803, USA
2
Ginzton Laboratory, Stanford University, Stanford, CA 94305, USA
3
Geballe Laboratory of Advanced Materials, Stanford University, Stanford, CA 94305, USA
∗
E-mail: gveronis@lsu.edu
Received December 23, 2008
We review some of the recent advances in the development of subwavelength plasmonic devices for ma-
nipulating light at the nanoscale, drawing examples from our own work in metal-dielectric-metal (MDM)
plasmonic waveguide devices. We introduce bends, splitters, and mod e converters for MDM waveguides
with no additional loss. We also demonstrate that optical gain provides a mechanism for on/off switch-
ing in MDM plasmonic waveguides. Highly efficient compact couplers between dielectric waveguides and
MDM waveguides are also introduced.
OCIS codes: 130.2790, 240.6680, 260.2110.
doi: 10.3788/COL20090704.0302.
1. Introduction
Light-guiding structures which allow subwavelength
confinement of the optical mode ar e important for
achieving compact integrated photonic devices
[1−7]
. The
minimum confinement of a guided optical mode in di-
electric waveguides is set by the diffraction limit and
is o f the order of λ
0
/n, where λ
0
is the wavelength in
free space and n is the refractive index. As opposed to
dielectric waveguides, plasmonic waveguides have shown
the p otential to guide subwavelength optical modes , the
so-called surface plasmon pola ritons, at metal-dielectric
interfaces.
Several different plasmonic waveguiding structures
have bee n proposed, such as metallic nanowires
[2,3]
and
metallic nanoparticle arrays
[4,5]
. Most of these s truc-
tures support a highly-confined mode only near the
surface plasmon fr equency. In this regime, the optical
mode typically has low group velocity and short propa-
gation length. It has been shown however that a metal-
dielectric-metal (MDM) structure with a dielectric region
thickness of ∼100 nm supports a propag ating mo de with
a nanoscale modal size at a waveleng th range extend-
ing from zero-frequency (DC) to visible
[8]
. Thus, such a
waveguide could be potentially important in providing
an interface betwe en conventional optics and subwave-
length electronic and optoelectronic devices. Because
of the predicted attractive properties of MDM waveg-
uides, their modal structure has been studied in great
detail
[8−12]
, and people have also started to explore such
structures experimentally
[13−15]
. Recent resear ch work
has therefore focused on the development of functional
plasmonic devices, including active devices, for nanoscale
plasmonic integrated circuits.
Here we provide a review of some of our own rec e nt re-
search activities aiming to advance the state of the art of
plasmonics through the introduction of novel MDM plas-
monic waveguide dev ic e s for manipulating light at the
nanoscale
[16−19]
. We first briefly review the simulation
method used in our studies, then introduce bends, split-
ters, and mode converters for MDM waveguides with no
additional loss. We also demonstrate that optical gain
provides a mechanism for on/off switching in MDM
plasmonic waveguides. Finally, we introduce highly
efficient compact couplers betwe en dielectric waveguides
and MDM waveguides.
2. Simulation method
We study the pro perties of MDM plasmonic waveguide
devices using a two-dimensional (2D) finite-difference
frequency-domain (FDFD) method
[20,21]
. This method
allows us to directly use experimental data for the
frequency-dependent dielectric constant of metals such
as silver
[22]
, including both the real and imaginary parts,
with no further approximation. Perfectly matched layer
(PML) absorbing boundary conditions are used at all
boundaries of the simulation domain
[23]
.
Due to the rapid field variation at the metal-dielectric
interfaces, a very fine grid re solution of ∼1 nm is re-
quired at the metal-dielectric interfaces to adequately
resolve the local fields. On the other hand, a grid res-
olution of ∼ λ/20 is s ufficient in other regions of the
simulation domain. For example, the required grid size
in air a t λ
0
= 1.5 5 µm is ∼7 7.5 nm, which is almost
two orders of magnitude larger than the required gr id
size at the metal-dielectric interfaces. We therefore use a
nonuniform orthogonal grid
[24]
to avoid an unnecessary
computational cost. We fo und that by using such a grid
our results are accurate to ∼0.05 %.
3. Bends and splitters
In this section, we investigate the performance o f
bends and power splitters in MDM plasmo nic waveg-
1671-7694/2009/040302-07
c
2009 Chinese Optics Letters
April 10, 2009 / Vol. 7, No. 4 / CHINESE OPTICS LETTERS 303
uides. Waveguide be nds and s plitters are basic struc-
tures for optical interconnects and therefore essential
components of optical integrated c ircuits
[6,25]
. Here, the
relevant question is w hether MDM bends and splitters
will induce reflection or excess absor ption loss on top of
the propagation loss in the waveguides.
To answer this question, we ca lc ulate the transmis-
sion coefficient of bends and splitters and normalize it
with respect to the transmission coefficient of a straight
waveguide with the same length. In all cases, the waveg-
uide width d is much smaller than the wavelength so
that only the fundamental TM waveguide mode (with
magnetic field perp e ndicula r to the direction of propaga-
tion) is excited. In Fig. 1, we show the calculated power
transmission coefficient of a 90
◦
sharp MDM waveguide
bend (inset of Fig. 1) a s a function of wavelength. We
observe that there is no bending loss in a broad wave-
length range that extends from DC to near-infrared. This
range includes the optical communication waveleng th of
1.55 µm.
To explain the absence of bending loss in subwave-
length MDM bends, we introduce an effective char-
acteristic impedance model based upon the dispersion
relation of the MDM waveguide structures. The char-
acteristic impedance of the fundamental TEM mode in
a perfect electric conductor (PEC) parallel-plate waveg-
uide is uniquely defined as the ratio of voltage V to
surface current density I and is equal to
[26]
Z
TEM
≡
V
I
=
E
x
d
H
y
=
β
TEM
ωǫ
0
d =
r
µ
0
ǫ
0
d, (1)
where E
x
, H
y
are the transverse compo nents of the elec-
tric and magnetic fields, respectively, and we assumed a
unit-length waveguide in the y direction. For non-TEM
modes, such as the fundamental MDM mode, voltage
and current are not uniquely defined. However, metals
like silver satisfy the condition |ǫ
metal
| ≫ ǫ
diel
at the op-
tical communication wavelength of 1.55 µm
[22]
. Thus,
|E
x metal
| ≪ |E
x diel
| , so that the integral of the electric
field in the transverse direction can be approximated by
E
x diel
d and we may therefore define the characteristic
impeda nce o f the fundamental MDM mode as
Z
MDM
(d) ≡
E
x diel
d
H
y diel
=
β
MDM
(d)
ωǫ
0
d, (2)
where β
MDM
(d) = 2π/λ
g
(d) is the real part of the mode
propagation constant
[26]
, and λ
g
is the guide wavelength
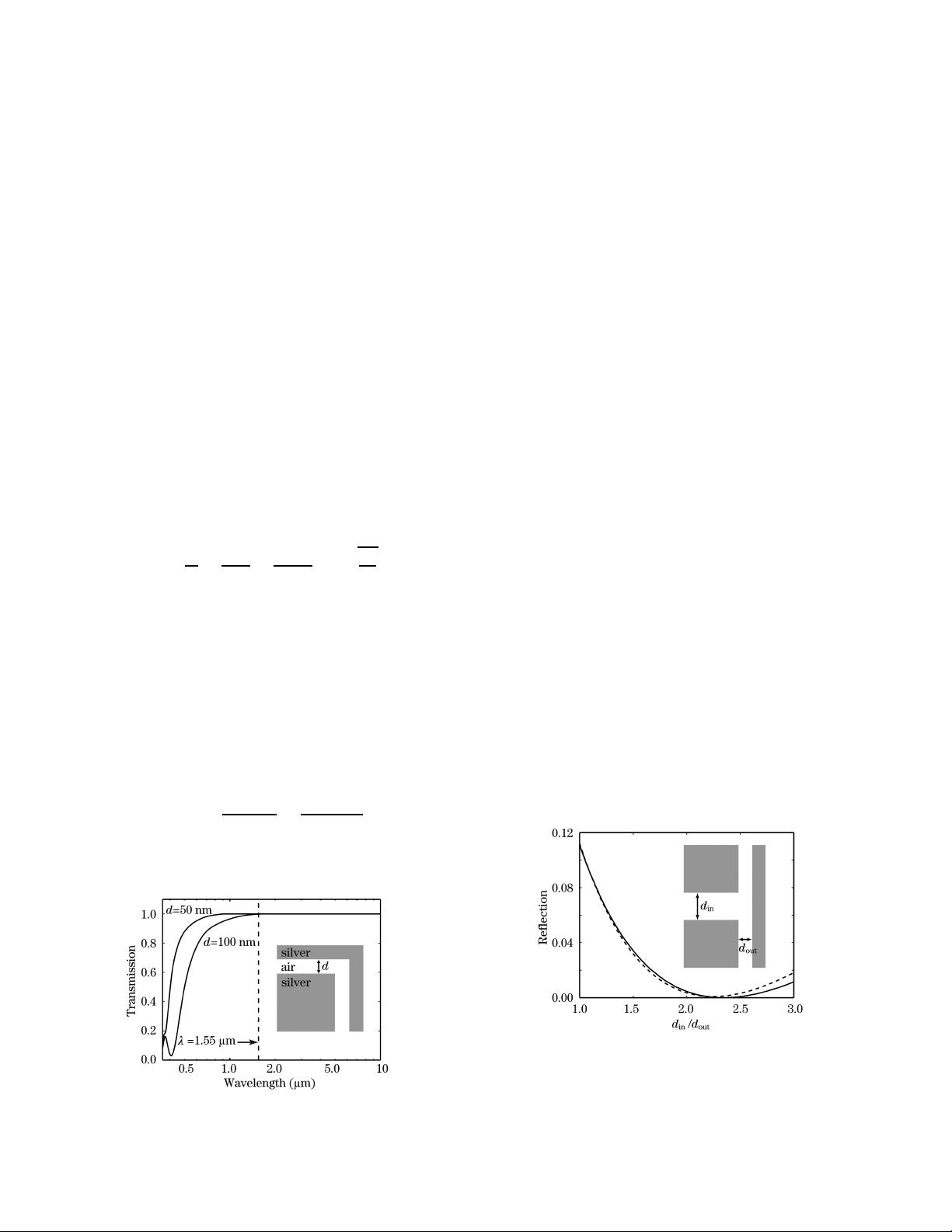
Fig. 1. Power transmission spectra of a MDM waveguide bend
(shown in the inset) calculated using FDFD.
of the fundamental TM mode in the MDM waveguide,
which is smaller than the free-space wavelength λ
0
[8]
.
In the ca se of a MDM bend, if the structure dimensions
are small in comparison with the wavelength, the qua-
sistatic approximation holds
[26]
. Under the quasis tatic
approximation, the bend is e quivalent to a junction be-
tween two transmission lines with the same character-
istic impeda nce , and there is therefore no bending loss.
The limiting wavelength λ
c
at which the trans mission
coefficient decreases below 99% is 1.27 µm (0.76 µm) for
d = 100 nm (d = 5 0 nm) (Fig. 1). The operating wave-
length range widens as d decreas e s, b ecause fo r thinner
structures the qua sistatic approximation holds over a
wider range of wavelengths.
We also c alculate the transmission s pectr a of MDM
splitters (inset of Fig. 2). The frequency response
of MDM splitters is quite similar to the response of
MDM bends. At long wavelengths the transmission is
equal to 44.4% for d
in
= d
out
. Under the quasistatic
approximation, which holds at long wavelengths, the
splitter is equivalent to a junction of three transmis-
sion lines with the same characteristic impeda nce Z
0
.
The load connected to the input transmissio n line at
the junction consists of the series combination of the
two output trans mission lines. Thus, the e quivalent load
impeda nce is Z
L
= 2Z
0
and the reflection coefficient is
¯
R = |(Z
L
− Z
0
)/(Z
L
+ Z
0
)|
2
= 1/9. Because of the sym-
metry of the structure, the transmitted optical p ower
is equally distributed between the two output waveg-
uide branches, so that the transmission coefficient is
T = 4/9 = 44.4%.
Based on the above discussion, in order to improve
the transmission coefficient of the MDM splitter, we can
adjust the characteristic impe dance of the input waveg-
uide Z
in
so that Z
in
≃ Z
L
= 2Z
0
. The input impedance
Z
in
can be adjusted by varying the thickness d
in
of the
input waveguide. In Fig. 2, we show the calculated
reflection coefficient R of the MDM T-s haped splitter
at λ
0
= 1.55 µm as a function of d
in
/d
out
, for d
out
=
50 nm (inset of Fig. 2). We note that at λ
0
=
1.55 µm the propagation length of the fundamental
MDM mode is much larger than the splitter dimen-
sions so that the contribution of excess absorption to
the splitter loss is negligible. We observe that the
Fig. 2. Reflection coefficient R of a MDM T-shaped split-
ter (shown in the inset) as a function of d
in
/d
out
at λ
0
=
1.55 µm calculated using FDFD. We also show with dashed
line the reflection coefficient
¯
R calculated based on the char-
acteristic impedance Z
MDM
and transmission-line theory. Re-
sults are shown for d
out
= 50 nm.
剩余6页未读,继续阅读
资源评论

weixin_38600460
- 粉丝: 5
- 资源: 955
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功