有关Mayer展开和矩阵模型的注释资源-CSDN文库

Open

Access

189 浏览量 2020-05-03 06:11:06 上传评论收藏 455KB PDF 举报

资源推荐

资源详情

资源评论

Available online at www.sciencedirect.com

ScienceDirect

Nuclear Physics B 880 (2014) 476–503

www.elsevier.com/locate/nuclphysb

Notes on Mayer expansions and matrix models

Jean-Emile Bourgine

Asia Paciﬁc Center for Theoretical Physics (APCTP), Pohang, Gyeongbuk 790-784, Republic of Korea

Received 30 October 2013; accepted 22 January 2014

Available online 24 January 2014

Abstract

Mayer cluster expansion is an important tool in statistical physics to evaluate grand canonical partition

functions. It has recently been applied to the Nekrasov instanton partition function of N = 2 4d gauge

theories. The associated canonical model involves coupled integrations that take the form of a generalized

matrix model. It can be studied with the standard techniques of matrix models, in particular collective ﬁeld

theory and loop equations. In the ﬁrst part of these notes, we explain how the results of collective ﬁeld theory

can be derived from the cluster expansion. The equalities between free energies at ﬁrst orders is explained

by the discrete Laplace transform relating canonical and grand canonical models. In a second part, we study

the canonical loop equations and associate them with similar relations on the grand canonical side. It leads

to relate the multi-point densities, fundamental objects of the matrix model, to the generating functions of

multi-rooted clusters. Finally, a method is proposed to derive loop equations directly on the grand canonical

model.

1. Introduction

The AGT correspondence [1] implies a relation between the canonical partition function of

a β-ensemble and the grand canonical partition function of a generalized matrix model. The

former represents a correlator of Liouville theory, according to the proposal of Dijkgraaf and

Vafa [2], further investigated in [3–11]. The latter describes the instanton partition function of

a4dN = 2 supersymmetric gauge theory in the Ω-background, as derived using localization

techniques in [12]. Here the term ‘generalized matrix model’ do not pertain to a matrix origin

E-mail address: jebourgine@apctp.org.

http://dx.doi.org/10.1016/j.nuclphysb.2014.01.017

Funded by SCOAP

Open access under CC BY license.

Funded by SCOAP

Open access under CC BY license.

J.-E. Bourgine / Nuclear Physics B 880 (2014) 476–503 477

for the model, but instead refers to a set of models that can be studied using techniques initially

developed in the realm of matrix models. Among these techniques, the topological recursion

[13] exploits the invariance of the integration measure to derive a tower of nested equations

satisﬁed by the correlators of the model. These equations, referred as loop equations,aresolved

employing methods from algebraic geometry. This technique has recently been extended to a

wide spectrum of coupled integrals models in [14].

In a suitable limit of the β-ensemble,

AGT-

equivalent to the Nekrasov–Shatashvili (NS) limit

of the Ω-background [15], loop equations are no longer algebraic but ﬁrst order linear differential

equations.

In this context, the β-ensemble is a natural quantization of the Hermitian matrix

model, to which it reduces at β = 1. The ﬁrst element of this tower of differential equations has

been mapped to the TQ relation derived in [16–18] that describes the dual SUSY gauge theory

in the NS limit [19–22]. It is then natural to ask about the existence of a structure similar to loop

equations on the gauge side of the correspondence.

But so far, the loop equation technique has

not been applied to grand canonical matrix models. On the other hand, the cluster expansion of

Mayer and Montroll [26] has been successfully employed to derived an effective action relevant

to the NS limit [15]. Can we relate this cluster expansion to the topological expansion of a

generalized matrix model? Is there an equivalent of the loop equations technique on the grand

canonical side? And more generally, how do canonical and grand canonical coupled integrals

relate to each other? These are the issues we propose to address in these notes.

For this purpose, we consider the following grand canonical generalized matrix model,

( ¯q) =

∞



N=0

¯q

(N), Z

(N) =





i=1

Q(φ

)

dφ

2iπ



i,j=1

i<j

K(φ

−φ

). (1.1)

In analogy with the Nekrasov partition function, integrals are understood as contour integrals

over the real line. The potential Q(x) and the kernel K(x) are free of singularities over the

real axis.

We propose to study the expansion of Z

(q) when the kernel is close to one. More

precisely, we assume the form

K(x) = 1 + f (x),  → 0, (1.2)

with f an even function, non-vanishing at x = 0. Although the results of these notes are very

general, what we have in mind for the function f is typically

f(x)=

−γ

, Im γ =0. (1.3)

It is crucial for our considerations that f is independent of . In this way, we exclude a class of

models more relevant to the study of Nekrasov partition functions. For instance, setting  = γ

one recovers the model proposed by J. Hoppe in [27]. This model is a one-parameter version

of the Nekrasov partition function that depends on two Ω-background equivariant deformation

parameters 

and 

[28,29].As →0, it exhibits a phenomenon referred as instanton clustering

Except for the ﬁrst (planar) equation, which is a Riccati equation, therefore non-linear. It is equivalent to a Schrödinger

equation, i.e. a linear differential equation of second order.

Such a structure should be related to the invariance of Nekrasov partition functions under transformations representing

the SHc algebra uncovered in [23] (see also [24,25]).

In the case of real singularities, a prescription should be given to move away the poles from the contour by a small

imaginary shift.

478 J.-E. Bourgine / Nuclear Physics B 880 (2014) 476–503

Fig. 1. First orders in the Mayer expansion of the free energy.

in the context of SUSY gauge theories [15]. It corresponds to poles coming from the kernel and

pinching the integration contour. Such poles should be avoided by a deformation of the contour,

picking up the corresponding residues. As a result, terms of the -expansions we are considering

are reshufﬂed and the results presented here are no longer valid.

These notes are organized as follows. In the second section, we compare the Mayer cluster

xpansion of the

grand canonical model with the collective ﬁeld theory describing the large

N limit of the canonical model. Taking the coupled limit  → 0 and N →∞with N ﬁxed,

we derive relations between the free energies at ﬁrst orders. These relations are a consequence

of the fact that the grand canonical partition function is the discrete Laplace transform of the

canonical one. We go on with the study of the canonical loop equations. We show that they relate

to graphical identities between generating functions of rooted clusters. Such generating functions

show up in the Mayer expansion and are identiﬁed with the multi-point densities. Finally, we

present a technique to derive directly the grand canonical loop equations. The main results are

summarized in the concluding section.

2. Comparison of the free energies at ﬁrst orders

2.1. Mayer expansion of the grand canonical model

The cluster expansion was introduced by Mayer and Montroll as a way to compute the free

energy knowing the form of the interaction between particles [26] (see also the book [30] and

the excellent review by Andersen [31]). It allows to derive the equation of state for various types

of ﬂuids. To do so, the kernel is expanded in , which corresponds to strength of molecular

interactions in the case of non-ideal gases. The terms of the series consist of coupled integrals

with the kernel f instead of K, and their expression is encoded into clusters. Here, a cluster is a

set of vertices connected by at most one link. The partition function is a sum over disconnected

clusters, but after taking the logarithm the summation is restricted to connected ones. We denote

by C

a generic connected cluster with l vertices, E(C

) the set of its links (or edges) and V(C

)

the set of vertices. To each vertex i of a cluster is associated an integration over the particle of

coordinate φ

with measure ¯qQ(φ

)dφ

/2iπ. The edge ij  between particles i and j represents

the kernel f (φ

−φ

). Thus, the logarithm of the partition function writes

logZ

( ¯q) =

∞



l=0

¯q



σ(C

)





i∈V(C

)

Q(φ

)

dφ

2iπ



ij ∈E(C

)

f (φ

−φ

), (2.1)

where the symmetry factor σ(C

) is the cardinal of the group of automorphism for the cluster, i.e.

the number of permutations of vertices that leave C

invariant. The ﬁrst terms of the expansion

and their symmetry coefﬁcients are given in Fig. 1.

The Mayer expansion (2.1) is an expansion

at small (bare) fugacity ¯q.Wewouldliketore-

formulate it as a ¯q-exact expansion in the parameter . We will also renormalize the fugacity,

keeping q =¯q ﬁxed. By analogy, ¯q would encode the gauge coupling constant of the Nekrasov

partition function, and the Mayer cluster expansion is an expansion upon the number of instan-

tons. More precisely, ¯q would correspond to q

gauge

(

+ 

)/



and should be renormalized

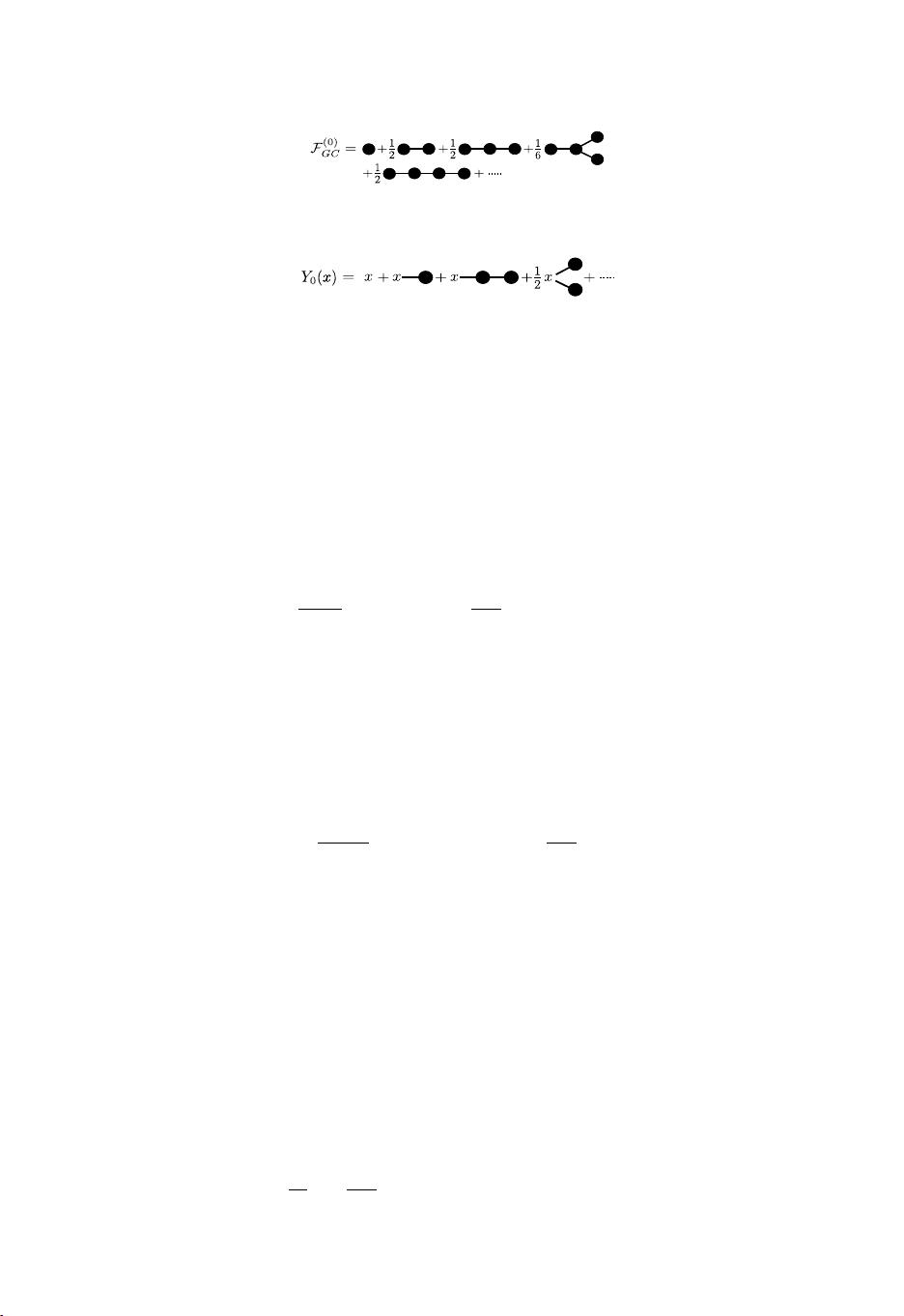
J.-E. Bourgine / Nuclear Physics B 880 (2014) 476–503 479

Fig. 2. The leading order free energy as a sum over trees.

Fig. 3. First terms in the expansion of the rooted tree generating function Y

(x).

by a factor 

in the NS limit 

→ 0. In this context, the -expansion we study corresponds

to an expansion in the Ω-background parameter 

, or in the AGT dual, to the semi-classical

expansion of Liouville correlators.

Since each link brings a factor of ,

at ﬁrst order

only the clusters with a minimal number of

links contribute. These clusters, denoted T

, have a tree structure, with l −1 links for l vertices.

Thus, at ﬁrst order in  the free energy is given by the following sum over trees,

(0)

(q) = lim

→0

 logZ

( ¯q)

∞



l=0



σ(T

)





i∈V(T

)

Q(φ

)

dφ

2iπ



ij ∈E(T

)

f(φ

), (2.2)

where we used the shortcut notation φ

= φ

− φ

. Note that we have renormalized the free

energy by a factor of , which is reminiscent of the volume of the Ω-background 



by which

the prepotential should be multiplied in order to be ﬁnite in the R

limit 

,

→ 0. The ﬁrst

terms of this expansion are given in Fig. 2.

To evaluate F

(0)

, it is convenient to consider the generating function of rooted trees T

deﬁned as

(x) =qQ(x)

∞



l=0



σ(T

)





i∈V(T

{x})

qQ(φ

)

dφ

2iπ



ij ∈E(T

{x})

f(φ

)



xi∈E(T

)

f(x−φ

), (2.3)

where with a slight abuse of notations we denoted the root and its coordinate by the same letter x.

The ﬁrst order terms of this expansion are given in Fig. 3. This function is interpreted as a

tree-level dressed vertex. We should also emphasize that ‘rooting’ a tree, or marking a vertex,

reduces the symmetry factor σ(T

)  σ(T

) since automorphisms are now constraint to leave

the root, or the marked vertex, invariant.

The function Y

(x) obeys an integral equation that can be obtained as follows. Let us assume

that the root x is directly connected to p vertices, and sum over the possible numbers p. Each of

these p vertices is the root of a new tree, and we deduce the relation,

(x) =qQ(x)

∞



p=0





2iπ

f(x−y)Y

(y)



, (2.4)

480 J.-E. Bourgine / Nuclear Physics B 880 (2014) 476–503

Fig. 4. Graphical representation of the recursion relation obeyed by Y

graphically represented on Fig. 4. The symmetry factor p! takes into account the possibility of

permuting the p vertices. Performing the summation, and taking the logarithm, we obtain the

integral equation satisﬁed by Y

log



(x)

qQ(x)





2iπ

f(x−y)Y

(y). (2.5)

It remains to relate the free energy to the generating function Y

. This is done using the

following formula due to B. Basso, A. Sever and P. Vieira [32],

(0)

(q) =



(x)

2iπ

−



2iπ

(x)Y

(y)f (x −y). (2.6)

It is easy to see that both terms in the RHS will produce a sum over clusters weighted by the same

integrals as in (2.2), but with different symmetry factors. A combinatorial proof of this formula

is given in Appendix A.

It is useful to reformulate the previous expression (2.6) of

the free ener

gy at ﬁrst order as the

value of an effective action S

] at its extremum Y

∗

(0)

(q) =S



∗



, such that

δS

δY



∗

=0. (2.7)

This action is obtained after introducing the integral equation (2.5) into (2.6),



2iπ

(x)Y

(y)f (x −y) −



2iπ

(x)



logY

(x) −1





2iπ

(x) log



qQ(x)



. (2.8)

It is remarkable that the saddle point equation derived from this action is nothing else than the

integral equation (2.5). It is also worth noticing that when instanton clustering phenomenon is

taken into account, one arrive at a similar expression, with logarithms replaced by dilogarithms

in the second term. For instance, the effective action derived by Nekrasov and Shatashvili to

describe N =2 SYM reads

[ρ]=



ρ(x)ρ(y)G(x −y) +







1 −e

−ρ(x)



−ρ(x)log



1 −e

−ρ(x)





ρ(x)log



qQ(x)



dx, (2.9)

This very useful formula was brought to my knowledge by B. Basso. So far, we were unable to ﬁnd a proper reference

in the previous literature. However, similar considerations were presented in [33].

剩余27页未读，继续阅读

评论收藏

内容反馈

weixin_38514872

粉丝: 6
资源: 879

有关Mayer展开和矩阵模型的注释

Nekrasov势的Mayer展开：次导ε2阶

纠缠，时空和Mayer-Vietoris定理

论文研究 - 2连通图族的Ree-Hoover和Mayer权重的其他公式

论文研究 - 图的无限族的Mayer和Ree-Hoover权重的新公式

论文研究 - 病例报告：Mayer-Rokitansky-Kuster-Hauser综合征的临床和诊断方面

通过Mayer-Vietoris定理进行多部分纠缠

新标准c++ Scott mayer

含氮煤焦边缘模型氧化生成NO途径研究

本数据包含一个多模态类比推理数据集MARS和一个多模态知识图谱数据集MarKG用来评估多模态模型的类比推理能力

论文研究 - 企业家作为信托的建设者：集成模型

《Python One-Liners》 by Christian Mayer .pdf

danmayer.github.com:丹·梅耶（Dan Mayer）的开发博客

mayer制版软件

A ReportPrinting Framework on Mike Mayer's ReportPrinting core library

QCD重子数波动的簇扩展模型：在μB/ T &lt;π时无相变

对N = 2 SYM位势的有限γ2-校正

12.-MRP-Workshop-Critical-Communications-Georg-Mayer-v0.1.pdf

Qt 5实现串口调试助手 （源工程文件、0积分下载）

【SystemVerilog】路科验证V2学习笔记（全600页）.pdf

AutoSAR标准协议4.2.2

光伏-储能并网系统仿真.rar

XCP协议的规范文档

GD32替换STM32注意事项.pdf

NPPJSONViewer.zip

蓝牙BLE协议中文版.pdf

CANoe通过CAPL脚本实现自动测试

电路分析基础第二版PDF电子书免费下载

最新资源

QCD重子数波动的簇扩展模型：在μB/ T <π时无相变

Qt 5实现串口调试助手（源工程文件、0积分下载）