# Binary Tree Traversal
## Overview
The combination of binary trees being data structures and traversal being an algorithm relates to classic problems, either directly or indirectly.
> If you can grasp the traversal of binary trees, the traversal of other complicated trees will be easy for you.
The following are some common ways to traverse trees.
- Depth First Traversals (DFS): In-order, Pre-order, Post-order
- Level Order Traversal or Breadth First or Traversal (BFS)
There are applications for both DFS and BFS.
Stack can be used to simplify the process of DFS traversal. Besides, since tree is a recursive data structure, recursion and stack are two key points for DFS.
Graph for DFS:
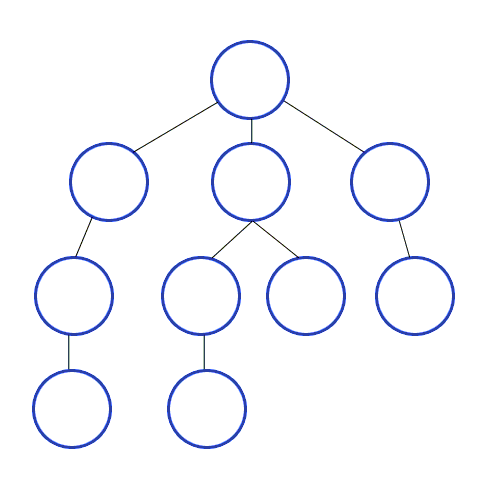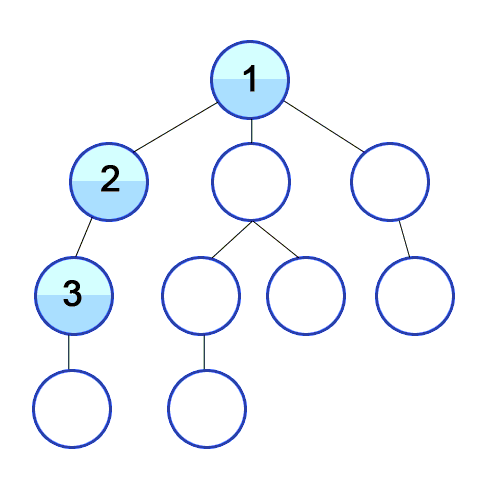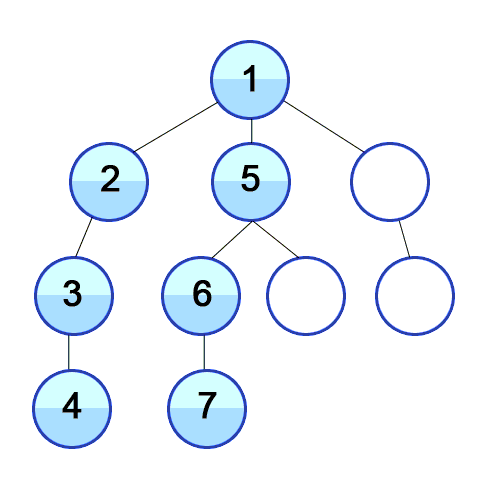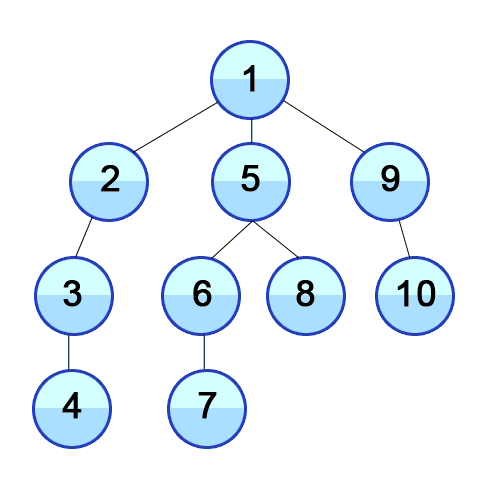
The key point of BFS is how to determine whether the traversal of each level has been completed. The answer is to use a variable as a flag to represent the end of the traversal of current level.
## Pre-order Traversal
The traversal order of pre-order traversal is `root-left-right`.
Algorithm Pre-order
1. Visit the root node and push it into a stack.
2. Pop a node from the stack, and push its right and left child node into the stack respectively.
3. Repeat step 2.
Conclusion: This problem involves the classic recursive data structure (i.e. a binary tree), and the algorithm above demonstrates how a simplified solution can be reached by using a stack.
If you look at the bigger picture, you'll find that the process of traversal is as followed. `Visit the left subtrees respectively from top to bottom, and visit the right subtrees respectively from bottom to top`. If we are to implement it from this perspective, things will be somewhat different. For the `top to bottom` part we can simply use recursion, and for the `bottom to top` part we can turn to stack.
## In-order Traversal
The traversal order of in-order traversal is `left-root-right`.
So the root node is not printed first. Things are getting a bit complicated here.
Algorithm In-order
1. Visit the root and push it into a stack.
2. If there is a left child node, push it into the stack. Repeat this process until a leaf node reached.
> At this point the root node and all the left nodes are in the stack.
3. Start popping nodes from the stack. If a node has a right child node, push the child node into the stack. Repeat step 2.
It's worth pointing out that the in-order traversal of a binary search tree (BST) is a sorted array, which is helpful for coming up simplified solutions for some problems.
## Post-order Traversal
The traversal order of post-order traversal is `left-right-root`.
This one is a bit of a challenge. It deserves the `hard` tag of LeetCode.
In this case, the root node is printed not as the first but the last one. A cunning way to do it is to:
Record whether the current node has been visited. If 1) it's a leaf node or 2) both its left and right subtrees have been traversed, then it can be popped from the stack.
As for `1) it's a leaf node`, you can easily tell whether a node is a leaf if both its left and right are `null`.
As for `2) both its left and right subtrees have been traversed`, we only need a variable to record whether a node has been visited or not. In the worst case, we need to record the status for every single node and the space complexity is `O(n)`. But if you come to think about it, as we are using a stack and start printing the result from the leaf nodes, it makes sense that we only record the status for the current node popping from the stack, reducing the space complexity to `O(1)`.
## Level Order Traversal
The key point of level order traversal is how do we know whether the traversal of each level is done. The answer is that we use a variable as a flag representing the end of the traversal of the current level.
Algorithm Level-order
1. Visit the root node, put it in a FIFO queue, put in the queue a special flag (we are using `null` here).
2. Dequeue a node.
3. If the node equals `null`, it means that all nodes of the current level have been visited. If the queue is empty, we do nothing. Or else we put in another `null`.
4. If the node is not `null`, meaning the traversal of current level has not finished yet, we enqueue its left subtree and right subtree respectively.
## Bi-color marking
We know that there is a tri-color marking in garbage collection algorithm, which works as described below.
- The white color represents "not visited".
- The gray color represents "not all child nodes visited".
- The black color represents "all child nodes visited".
Enlightened by tri-color marking, a bi-color marking method can be invented to solve all three traversal problems with one solution.
The core idea is as follow.
- Use a color to mark whether a node has been visited or not. Nodes yet to be visited are marked as white and visited nodes are marked as gray.
- If we are visiting a white node, turn it into gray, and push its right child node, itself, and it's left child node into the stack respectively.
- If we are visiting a gray node, print it.
Implementation of pre-order and post-order traversal algorithms can be easily done by changing the order of pushing the child nodes into the stack.
Reference: [LeetCode](https://github.com/azl397985856/leetcode/blob/master/thinkings/binary-tree-traversal.en.md)
没有合适的资源?快使用搜索试试~ 我知道了~
Python 算法集.zip

共1414个文件
py:1321个
txt:45个
md:23个
需积分: 5 0 下载量 87 浏览量
2024-09-14
17:49:28
上传
评论
收藏 8.19MB ZIP 举报
温馨提示
Python 算法集.zip
资源推荐
资源详情
资源评论
收起资源包目录
 Python 算法集.zip (1414个子文件)
Python 算法集.zip (1414个子文件)  sample_data.csv 70KB
sample_data.csv 70KB ex_data.csv 1KB
ex_data.csv 1KB perceptron.py.DISABLED 7KB
perceptron.py.DISABLED 7KB get_imdbtop.py.DISABLED 1KB
get_imdbtop.py.DISABLED 1KB Dockerfile 406B
Dockerfile 406B pytest.ini 60B
pytest.ini 60B example_wikipedia_image.jpg 476KB
example_wikipedia_image.jpg 476KB output.jpg 116KB
output.jpg 116KB PSNR-example-comp-10.jpg 104KB
PSNR-example-comp-10.jpg 104KB lena.jpg 102KB
lena.jpg 102KB input.jpg 59KB
input.jpg 59KB 2D_problems.jpg 57KB
2D_problems.jpg 57KB 2D_problems_1.jpg 40KB
2D_problems_1.jpg 40KB example_image.jpg 29KB
example_image.jpg 29KB lena_small.jpg 7KB
lena_small.jpg 7KB project_euler_answers.json 63KB
project_euler_answers.json 63KB devcontainer.json 1KB
devcontainer.json 1KB loudness_curve.json 812B
loudness_curve.json 812B settings.json 87B
settings.json 87B DIRECTORY.md 65KB
DIRECTORY.md 65KB CONTRIBUTING.md 11KB
CONTRIBUTING.md 11KB README.md 5KB
README.md 5KB README.md 5KB
README.md 5KB README.md 4KB
README.md 4KB local_weighted_learning.md 3KB
local_weighted_learning.md 3KB README.md 3KB
README.md 3KB README.md 3KB
README.md 3KB normal_distribution_quick_sort.md 2KB
normal_distribution_quick_sort.md 2KB README.md 1KB
README.md 1KB README.md 1KB
README.md 1KB README.md 894B
README.md 894B README.md 746B
README.md 746B README.md 726B
README.md 726B README.md 722B
README.md 722B ABOUT.md 452B
ABOUT.md 452B README.md 441B
README.md 441B README.md 415B
README.md 415B README.md 374B
README.md 374B README.md 303B
README.md 303B README.md 295B
README.md 295B README.md 264B
README.md 264B README.md 58B
README.md 58B PSNR-example-base.png 4.31MB
PSNR-example-base.png 4.31MB original_image.png 82KB
original_image.png 82KB gaussian.png 52KB
gaussian.png 52KB compressed_image.png 26KB
compressed_image.png 26KB red_black_tree.py 25KB
red_black_tree.py 25KB loss_functions.py 24KB
loss_functions.py 24KB graph_adjacency_matrix.py 22KB
graph_adjacency_matrix.py 22KB graph_adjacency_list.py 21KB
graph_adjacency_list.py 21KB wa_tor.py 20KB
wa_tor.py 20KB primelib.py 20KB
primelib.py 20KB sequential_minimum_optimization.py 20KB
sequential_minimum_optimization.py 20KB area.py 19KB
area.py 19KB index_calculation.py 19KB
index_calculation.py 19KB volume.py 17KB
volume.py 17KB linear_discriminant_analysis.py 17KB
linear_discriminant_analysis.py 17KB binary_search_tree_recursive.py 16KB
binary_search_tree_recursive.py 16KB convex_hull.py 16KB
convex_hull.py 16KB singly_linked_list.py 16KB
singly_linked_list.py 16KB directed_and_undirected_weighted_graph.py 15KB
directed_and_undirected_weighted_graph.py 15KB haralick_descriptors.py 15KB
haralick_descriptors.py 15KB word_search.py 15KB
word_search.py 15KB dijkstra_algorithm.py 15KB
dijkstra_algorithm.py 15KB mfcc.py 14KB
mfcc.py 14KB convolution_neural_network.py 14KB
convolution_neural_network.py 14KB lib.py 14KB
lib.py 14KB viterbi.py 14KB
viterbi.py 14KB double_ended_queue.py 14KB
double_ended_queue.py 14KB sol1.py 14KB
sol1.py 14KB k_means_clust.py 12KB
k_means_clust.py 12KB binomial_heap.py 12KB
binomial_heap.py 12KB resistor_color_code.py 12KB
resistor_color_code.py 12KB skip_list.py 12KB
skip_list.py 12KB multi_level_feedback_queue.py 12KB
multi_level_feedback_queue.py 12KB diffie_hellman.py 12KB
diffie_hellman.py 12KB input_data.py 12KB
input_data.py 12KB n_body_simulation.py 12KB
n_body_simulation.py 12KB binary_search.py 11KB
binary_search.py 11KB temperature_conversions.py 11KB
temperature_conversions.py 11KB two_hidden_layers_neural_network.py 11KB
two_hidden_layers_neural_network.py 11KB davis_putnam_logemann_loveland.py 11KB
davis_putnam_logemann_loveland.py 11KB simplex.py 11KB
simplex.py 11KB md5.py 11KB
md5.py 11KB matrix_class.py 11KB
matrix_class.py 11KB binary_search_tree.py 11KB
binary_search_tree.py 11KB frequent_pattern_growth.py 11KB
frequent_pattern_growth.py 11KB tabu_search.py 11KB
tabu_search.py 11KB weight_conversion.py 10KB
weight_conversion.py 10KB automatic_differentiation.py 10KB
automatic_differentiation.py 10KB lru_cache.py 10KB
lru_cache.py 10KB basic_graphs.py 10KB
basic_graphs.py 10KB lfu_cache.py 10KB
lfu_cache.py 10KB avl_tree.py 9KB
avl_tree.py 9KB decrypt_caesar_with_chi_squared.py 9KB
decrypt_caesar_with_chi_squared.py 9KB hamming_code.py 9KB
hamming_code.py 9KB binary_tree_traversal.py 9KB
binary_tree_traversal.py 9KB minimum_spanning_tree_prims2.py 9KB
minimum_spanning_tree_prims2.py 9KB enigma_machine2.py 9KB
enigma_machine2.py 9KB multi_heuristic_astar.py 8KB
multi_heuristic_astar.py 8KB共 1414 条
- 1
- 2
- 3
- 4
- 5
- 6
- 15
资源评论

YOLO数据集工作室
- 粉丝: 711
- 资源: 1590
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功