Matlab传染病模型资源-CSDN文库

共13个文件

m：8个

fig：4个

pdf：1个

5星 · 超过95%的资源需积分: 45 35 浏览量 2018-10-12 17:23:56 上传评论 19 收藏 2.48MB RAR 举报

在IT领域，尤其是在生物数学的研究中，Matlab是一种常用的工具，因其强大的数值计算和图形化界面而备受青睐。本文将详细探讨"Matlab传染病模型"的相关知识点，包括模型的基本概念、Matlab在生物数学中的应用以及如何通过提供的代码实现无病平衡点和地方病平衡点的稳定性分析。传染病模型通常用来模拟疾病在人群中的传播动态，帮助我们理解和预测疾病爆发的可能性、规模以及控制策略的效果。这些模型基于数学方程，如SIR（易感者-感染者-康复者）模型，SEIR（易感者-暴露者-感染者-康复者）模型等，其中每个字母代表一个群体状态。在Matlab中，我们可以利用ode或dde（延迟微分方程）求解器来模拟这些模型。"BM_dde23_wtl.m"可能就是一个使用dde23求解器的文件，这个求解器适用于处理具有常数或时间依赖延迟的二阶非线性微分方程。"xuejiede.m"可能是用于定义模型参数和方程的函数，"BM_Line.m"可能包含了模型的可视化部分，用于绘制传染病动态图。"商宁程序"可能是一位叫做商宁的作者编写的辅助程序或整个项目的集合。无病平衡点是指在没有疾病存在的情况下，人口中的易感者、感染者和康复者比例保持不变的状态。分析无病平衡点的稳定性是评估疾病是否会自发爆发的关键。这通常涉及到线性稳定性分析，通过计算系统的雅可比矩阵在平衡点处的特征值来确定。如果所有特征值的实部都为负，那么该平衡点是稳定的。地方病平衡点则是指疾病在小范围内持续存在的状态，即使没有新的输入，疾病也不会消失。分析这个地方病平衡点的稳定性有助于我们理解疾病的长期行为。这同样涉及线性稳定性分析，但可能需要考虑更多的数学细节，例如疾病的基本再生数R0，如果R0大于1，则地方病平衡点是不稳定的，疾病可能会在人群中持续传播。在Matlab中，这些稳定性分析可以通过编写自定义函数实现，结合ode或dde求解器的结果，计算出系统在平衡点附近的动态行为。"xuejiede.m"和"BM_dde23_wtl.m"可能包含了这些计算和分析的代码。 "Matlab传染病模型"涵盖了生物数学、传染病动力学和Matlab编程等多个领域的知识。通过理解并分析给定的代码，我们可以深入研究传染病的传播规律，并为疾病防控提供科学依据。对于学习和研究生物数学模型的人员来说，这是一个宝贵的资源。

资源推荐

资源详情

资源评论

收起资源包目录

新建文件夹.rar （13个子文件）

xuejiede.m 3KB

商宁程序

后向分支

shang1_0.m 740B

E2局部稳定，E1为鞍点，E0局部稳定

shang12.m 3KB

untitled.fig 1.08MB

E0全局稳定

untitled.fig 39KB

shang1.m 2KB

E2全局稳定

shang1_2.m 2KB

untitled.fig 160KB

E0不稳定

untitled.fig 675KB

shang1_1.m 2KB

hiv2.pdf 644KB

BM_dde23_wtl.m 23KB

BM_Line.m 2KB

J. Math. Anal. Appl. 434 (2016) 837–857

Contents lists available at ScienceDirect

Journal of Mathematical Analysis and Applications

www.elsevier.com/locate/jmaa

Bifurcations of a mathematical model for HIV dynamics

✩

Jianfeng Luo, Wendi Wang

∗

, Hongyan Chen, Rui F u

School of Mathematics and Statistics, Southwest University, Chongqing 400715, China

a r t i c l e i n f o a b s t r a c t

Article history:

Received 3 November 2014

Available online 28 September 2015

Submitted by Y. Du

Keywords:

Backward bifurcation

Bistable infections

Stability

Immune response

HIV

This paper investigates bifurcations and stability of an HIV model that incorporates

the immune responses. The conditions for the global stability of infection-free

equilibrium and infection equilibrium are respectively established by the Lyapunov

method and the geometric approach. The backward bifurcation from the infection-

free

equilibrium is examined by analytical analysis. More interestingly, with the

aid of mathematical analysis, we ﬁnd a new type of bifurcations from an infection

equilibrium, where a backward bifurcation curve emerges and can be continued to

the place where the basic reproduction number is less than unity. By numerical

simulations, we ﬁnd a variety of dynamical behaviors of the model, which reveal the

importance and complexity of immune responses in ﬁghting HIV replication.

1. Introduction

Since the emergence of human immunodeﬁciency virus (HIV) in the early 1980s, the disease of HIV

infection has been pandemic around the world and has caused 34 million infected and 25 million deaths so

far [36]. Due to its wide range of infectiousness, strong mortality and incurability, HIV has become a major

problem for human health. Biological studies indicate that HIV infects CD4

T helper cells. The phase of

primary infection is characterized by a strong viral replication, which is rapidly followed by a strong immune

response [3,15]. In the second phase of HIV infection, infected individual displays no symptoms, but has

persistent viral replications, which eventually results in the development of AIDS [15].

Mathematical

models have been employed to study the dynamics of HIV infection in vivo [4,7,8,18,29,

39,44,37,50].

The basic model of three state variables was proposed by [27,30]:

✩

The research is supported by the NSF of China (11171276).

* Corresponding author.

E-mail

address: wendi@swu.edu.cn (W. Wang).

http://dx.doi.org/10.1016/j.jmaa.2015.09.048

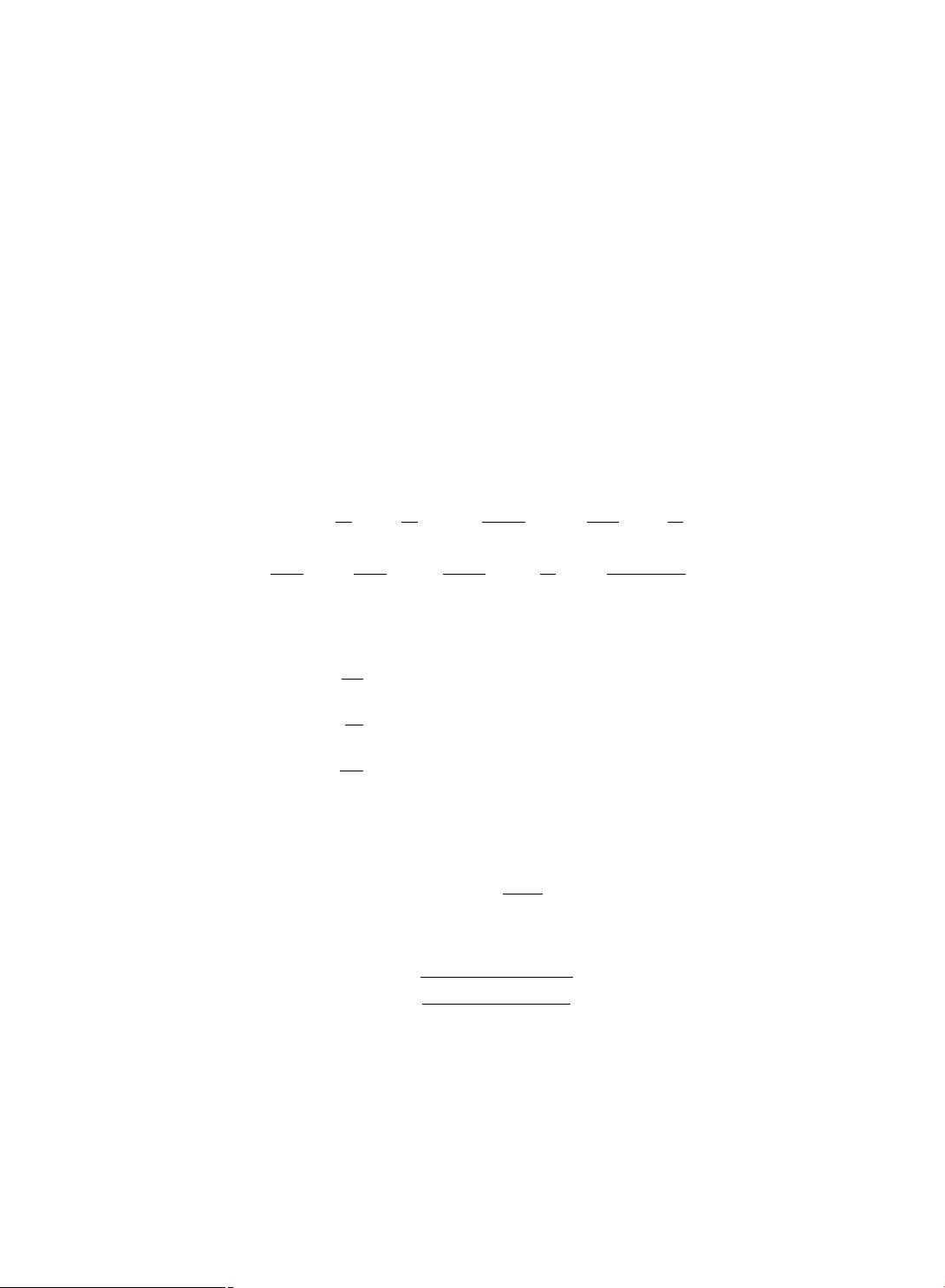
838 J. Luo et al. / J. Math. Anal. Appl. 434 (2016) 837–857

= λ − δ

T − βV T,

= βV T − δ

= NI − cV,

(1.1)

where T is the concentration of uninfected target cells, I is the concentration of infected cells, V is the

concentration of virus, λ is the production rate of new target cells, δ

and δ

are the death rates of unin-

fected

cells and infected cells respectively, β is the infection coeﬃcient, N is the burst coeﬃcient of virus

when infected cells die and c is the clearance rate of virus. Model (1.1) has made signiﬁcant contributions to

understand the dynamics of HIV infection and therapy to design speciﬁc treatment regimes [29,46]. How-

ever,

as noted in [29], since immune responses are not explicitly included, model (1.1) is suitable for the

asymptomatic phase of the disease during which immune responses are stable. Indeed, in the primary phase

of HIV infection, the cellular immune response to HIV is strong, grows with the rise in HIV in the blood

and then falls when that response reaches a peak [11,26].

Immune responses have been incorporated in the basic model of HIV infection (see, for example, [1,3,14,

20,21,28,31,32,34,35,43,45,47] and

the references cited therein) to understand how immune systems regulate

the dynamics of HIV infection. Most of these researches study eﬀects of cytotoxic T lymphocyte (CTL)

in ﬁghting HIV replication, and only a few consider the eﬀect of proliferation of CD4

T cells under the

stimulation of pathogens. Indeed, biological studies indicate that CD4

T cell activation plays a fundamental

role in guiding CTL responses [11,26]. In papers [2,33,48], an HIV model is proposed that incorporates CTL

responses to intracellular pathogens, CD4

T cell proliferation due to stimulations of pathogens, and B

cell responses to virus particles. The model admits only three state variables, which makes mathematical

analysis tractable. More importantly, the model captures the main features of the complex interactions of

HIV and immune responses. The dynamics of the variables are described by

⎧

⎪

⎨

⎪

⎩

=(λ + γ

IT)(1 − T/K) − δ

T − βV T,

= βV T − (δ

+ γ

T )I,

= NI − (c + γ

T )V −βV T.

(1.2)

Here, T is the concentration of helper T cells, I is the concentration of infected helper T cells, V is the

concentration of free virus. Parameter γ

represents the clonal ampliﬁcation of helper T cells after stimulation

by infected T cells. Further, the recruitment rate and the proliferation rate of helper T cells are limited

by the carrying capacity K. The model assumes that B cell and CTL responses are proportional to the

concentration of helper T cells. As a consequence, term γ

TI gives the removal rate of infected cells by CTL

responses and term γ

TV denotes the clearance rate of virus particles by antibodies. The last term −βV T

describes the loss rate of virus because of entry into target cells. All the other parameters in (1.2) have the

same meaning as those in (1.1).

Numerical simulations of model (1.2) in [2] found the case of asymptotic coexistence of immune system

and virus, and found the case of virus eradication. The stability of infection-free equilibrium of (1.2) was

examined in [48]. Model (1.2) was also extended by [48] to include stochastic cellular reproduction and

death, stochastic infection process, stochastic immune system activation, and stochastic viral reproduction.

In this paper, we explore the global behaviors of model (1.2) to ﬁnd possible evolutionary outcomes of

HIV infection under the control of immune responses. We establish conditions for the global stability of

infection-free equilibrium and infection equilibrium, which gives the conditions of infection eradication or

J. Luo et al. / J. Math. Anal. Appl. 434 (2016) 837–857 839

moving to the chronic infection. We show that model (1.2) exhibits the classical backward bifurcation from

the infection-free equilibrium (see, for example, [34,35]). More interestingly, we show that the activation of

CD4

T cell stimulated by pathogen can induce a new type of bifurcations at an infection equilibrium where a

backward bifurcation curve emerges and can be continued to the place where the basic reproduction number

is less than unity. This phenomenon reveals more diﬃculties to design treatment regimes. Moreover, by

numerical simulations, we ﬁnd a variety of dynamical behaviors of the model, which indicates the importance

and complexity of immune responses in ﬁghting HIV replication.

The

organization of this paper is as follows. In the next section, we study the existence of equilibria

of model (1.2). In Section 3, we present the local stability analysis of these equilibriums. Then we apply

the Li–Muldowney global-stability criterion [24] to derive suﬃcient conditions for the global stability of an

infection equilibrium. In Section 4, we study the bifurcations of model (1.2). Brief summary and discussions

are shown in the last section.

2. Existence of equilibria

Before going into details, we make the following scaling:

t = δ

T =

I =

V =

Kβγ

λ =

Kδ

,δ=

β =

Kγ

,γ=

Kγ

N =

NβK

,C=

,α=

K(β + γ

)

Dropping the bars, model (1.2) becomes

⎧

⎪

⎨

⎪

⎩

=(λ + IT)(1 − T ) − δT −βV T,

= VT − I − γTI,

= NI − CV −αV T,

(2.1)

where all the parameters are positive. Now, we analyze the equilibria of (2.1) and study the bifurcations on

the basis of distributions of these equilibria. The infection-free equilibrium (IFE) is

=(T

)



λ + δ

, 0, 0). (2.2)

By [40,48], the basic reproduction number of virus in (2.1) is



(1 + γT

)(C + αT

)

An infection equilibrium (

V )satisﬁes

⎧

⎪

⎨

⎪

⎩

(λ +

I)(1 −

T ) − δ

T − β

V =0,

V − (1 + γ

T )

I =0,

I − C

V − α

V =0.

(2.3)

Direct computations lead to

840 J. Luo et al. / J. Math. Anal. Appl. 434 (2016) 837–857

I =

T − λ(1 −

T )

T (1 −

T ) − (β + βγ

T )

V =

C + α

T − λ(1 −

T )

T (1 −

T ) − (β + βγ

T )

, (2.4)

where

T satisﬁes

the equation:

T )=αγ

+ B

T + C =0, (2.5)

in which,

B = α + Cγ −N.

Note

V =

IN/(C + α

T ). To ensure the existence of a positive equilibrium, we let B<0, and require

I>0, which is equivalent to

(

T − T

)g(

T ) > 0, (2.6)

where T

is given in (2.2) and

T )=−

+(1− βγ)

T − β. (2.7)

Set

Δ=B

− 4αγ C, Δ

=(1− βγ)

− 4β.

The positive equilibria of (2.1) are classiﬁed by the signs of Δ

and 1 − βγ. Let us consider two cases.

(C1)

< 0, or Δ

≥ 0 and βγ ≥ 1.

Note

that

:= [(1 + γT

)(C + αT

)]

> 0,

:= −2T

(1 + γT

)(C + αT

)(α + Cγ) < 0,

:= T

(α − Cγ)

> 0. (2.8)

Note also that

:= m

− 4m

=16αγCT

> 0.

We deﬁne

−m



− 4m

, (2.9)

which is clearly positive.

Theorem 2.1. Let B<0 and (C1) hold. Then we have the following conclusions.

(1) When R

> 1, system (2.1) admits a unique chronic-infection equilibrium

(2) Suppose −B/(2αγ) <T

. Then system (2.1) has no chronic-infection equilibrium if R



, has a

unique chronic-infection equilibrium

E if R



, and has two chronic-infection equilibriums

E and

E if



< 1.

(3) Suppose −B/(2αγ) = T

. Then system (2.1) has no chronic-infection equilibrium if R

≤



, has a

unique chronic-infection equilibrium

E if



< 1.

(4) System (2.1) has no chronic-infection equilibrium if −B/(2αγ) >T

and R

< 1.

J. Luo et al. / J. Math. Anal. Appl. 434 (2016) 837–857 841

Proof. Clearly, the assumptions in (C1) imply g(

T ) < 0. It follows from (2.6) that

T must lie in (0, T

). By

direct calculations we see that

f(T

)=(1+γT

)(C + αT

)(1 − R

Thus, f(T

) < 0if R

> 1, and f(T

) > 0if R

< 1. Since f(0) > 0, it follows that (2.5) admits a unique

positive root

T ∈ (0,

)if R

> 1.

Let us consider the case where R

< 1. If −B/(2αγ) >T

, since f(T

) > 0and f(0) > 0, it follows that

(2.5) has no positive root in (0, T

). Similarly, when −B/(2αγ) <T

and Δ > 0, (2.5) admits two positive

roots in (0, T

); when −B/(2αγ) <T

and Δ =0, (2.5) has a positive root in (0, T

); when Δ < 0, (2.5) has

no positive root in (0, T

); when −B/(2αγ) = T

and Δ =0, (2.5) has no positive root in (0, T

); when

−B/(2αγ) = T

and Δ > 0, (2.5) has exactly a positive root in (0, T

To determine the signs of Δin terms of the basic reproduction number, we denote R

by R

so that

N =

(1 + γT

)(C + αT

)

. (2.10)

It is easy to obtain

Δ=m

+ m

where m

are given in (2.8). Since B<0, we get

(α + γC)T

(1 + γT

)(C + αT

)

:= R

It is easy to examine R

< 1. If h(x) = m

+ m

x + m

, we have

h(R

)=m

+ m

= −4T

αγC < 0,

h(1) = m

+ m

=(αγT

− C)

> 0.

It follows that the equation of h(x) =0admits a root R

, which is described by (2.9), in interval (R

, 1).

Clearly, we have Δ =0when R



, Δ > 0when



, and Δ < 0when

√



Consequently, conclusions (2) and (3) of the theorem follow from the discussions of the last paragraph. 2

Let us now consider the second case.

(C2)

> 0 and βγ < 1.

The conditions in Assumption (C2) imply that g(T ) =0has two positive roots:

1 − βγ −

√

1 − βγ +

√

When B<0and Δ > 0, f (T ) =0has two positive roots:

−B −

√

2αγ

−B +

√

2αγ

When B<0and Δ =0, f(T ) =0has only one positive root:

−B

2αγ

评论收藏

内容反馈

df595420469

2023-07-25

这个文件简明地描述了 Matlab 传染病模型，让我对疾病传播有了更清晰的认识。
普通网友

2023-07-25

这个文件虽然没有太多花哨的功能，但它提供了一个基本的框架，供研究者们进一步扩展和优化，是个很好的起点。
西西里的小裁缝

2023-07-25

这个文件对于初学者来说是非常友好的，简单易懂的代码和清晰的解释让我很快上手。
鸣泣的海猫

2023-07-25

文件中提供了一种实用的方法来模拟传染病的传播，让我能够更好地理解疫情的发展规律。
苗苗小姐

2023-07-25

虽然是个小文件，但其中的内容足够详细，给出了一些实际场景下的模型参数和结果分析，让人深思。

weixin_43397985

粉丝: 2
资源: 1

Matlab传染病模型

matlab传染病模型.pdf

MATLAB源码集锦-传染病的SI SIS SIR 三种数学建模模型

传染病模型

数学建模的传染病模型

SARS 模型对应的代码.rar

seir用到的matlab代码-immunity:论文“群体免疫和持续SARS-CoV-2传播”的支持代码

传统传染病建模的matlab程序

基于Matlab的传染病动力学模型仿真平台

SIR_sis_SIRmatlab_SIR模型_SI_SIS模型_

matlab传染病模型.rar

MATLAB传染病模型.zip

传染病传播模型研究

seijr传染病模型

手足口病传播的数学模型建立与分析

改进SEIR模型的matlab代码.zip

数模_改进SEIR模型的matlab代码.zip

MATLAB求解SEIR模型.zip

SEIR 2（Matlab源码）

复杂网络BA模型（matlab编写）

具有阶段结构的SIRS传染病模型

构造复杂网络模型的MATLAB程序

MATLAB源码集锦-复杂网络研究中的一个病毒传播模型代码

复杂网络中基本网络模型的matlab实现

复杂网络上的新SIRS传播模型研究

SIS_1_0.3_传染病模型_传染病_sis_sis传染_SIS模型_

流行病传染模拟_matlab

MATLAB系统仿真之传染病案例

SEIR_SEIRMATLAB_SEIR模型_SIR模型_SEIR_

最新资源