DFBlaser_DFBlaser_DFB速率方程_速率方程_DFB_求解速率方程_半导体激光器的仿真代码资源-CSDN文库

共3个文件

pdf：1个

docx：1个

m：1个

版权申诉

速率方程

5星 · 超过95%的资源 168 浏览量 2021-09-11 14:08:09 上传评论 5 收藏 748KB ZIP 举报

DFB激光器，全称为分布式反馈（Distributed Feedback）激光器，是半导体激光器的一种，因其内部具有周期性光栅结构而得名。这种激光器的设计使得它在特定的波长上具有高度的选择性和稳定性，因此在通信、光谱分析、光纤传感等领域有广泛应用。DFB激光器的工作原理和性能很大程度上取决于其速率方程，这些方程描述了激光器内部的物理过程。速率方程是描述DFB激光器动态行为的关键数学模型，它们涵盖了载流子注入、增益饱和、辐射损耗以及光反馈等多个重要因素。主要的速率方程包括载流子密度方程、增益方程和光场方程。载流子密度方程反映了载流子的注入、复合和积累；增益方程则考虑了载流子对光增益的影响；光场方程则描述了光在激光器内的传播和反馈过程。龙格-库塔（Runge-Kutta）算法是一种数值求解微分方程的常用方法，尤其适合于非线性系统。在处理DFB激光器的速率方程时，由于这些方程通常是非线性的，并且涉及到多个相互耦合的过程，因此采用龙格-库塔算法可以有效地逼近真实解，特别是在模拟激光器动态响应时非常有效。 4阶龙格-库塔方法是最常见的版本，它通过四步迭代计算近似解，每一步都利用前几步的结果进行改进。这种方法既简单又高效，能提供较好的精度，是求解DFB激光器速率方程的常用工具。在实际应用中，可能还需要结合其他技术，如线性化处理、稳定性分析等，来优化求解过程并确保结果的可靠性。理解并掌握DFB激光器的速率方程及其求解方法对于设计和优化激光器的性能至关重要。通过调整激光器的结构参数，如光栅周期、量子阱深度和宽度，以及偏置电流等，可以改变速率方程的参数，从而实现对激光器发射波长和功率的控制。同时，了解如何用数值方法求解这些方程，可以帮助工程师预测和控制激光器的行为，避免潜在的不稳定现象，提高器件的稳定性和效率。在实际操作中，我们可能会使用编程语言，如Python或MATLAB，结合适当的物理模型和数值求解器来实现DFB激光器速率方程的求解。通过编写代码，可以自动化模拟过程，快速评估不同参数组合下的激光器性能，这对于科研和工程实践具有极大的价值。 DFB激光器的速率方程是其核心理论基础，而采用龙格-库塔算法进行求解则是理解和优化其性能的关键步骤。通过对速率方程的深入研究和数值模拟，我们可以更好地设计和调控DFB激光器，以满足各种应用需求。

资源推荐

资源详情

资源评论

收起资源包目录

DFB laser_DFBlaser_DFB速率方程_速率方程_DFB_求解速率方程_源码.zip （3个子文件）

DFB laser

~$文档1.docx 162B

Supelec518.pdf 905KB

DFBfankui.m 3KB

IEEE JOURNAL OF QUANTUM ELECTRONICS, VOL. 45, NO. 7, JULY 2009 879

Time-Delay Identiﬁcation in a Chaotic Semiconductor

Laser With Optical Feedback: A Dynamical

Point of View

Damien Rontani, Alexandre Locquet, Marc Sciamanna, David S. Citrin, and Silvia Ortin

Abstract—A critical issue in optical chaos-based communica-

tions is the possibility to identify the parameters of the chaotic

emitter and, hence, to break the security. In this paper, we study

theoretically the identiﬁcation of a chaotic emitter that consists of

a semiconductor laser with an optical feedback. The identiﬁcation

of a critical security parameter, the external-cavity round-trip

time (the time delay in the laser dynamics), is performed using

both the auto-correlation function and delayed mutual informa-

tion methods applied to the chaotic time-series. The inﬂuence

on the time-delay identiﬁcation of the experimentally tunable

parameters, i.e., the feedback rate, the pumping current, and

the time-delay value, is carefully studied. We show that difﬁ-

cult time-delay-identiﬁcation scenarios strongly depend on the

time-scales of the system dynamics as it undergoes a route to

chaos, in particular on how close the relaxation oscillation period

is from the external-cavity round-trip time.

Index Terms—Nonlinear dynamics, optical feedback, semicon-

ductor laser, time-delay identiﬁcation.

I. INTRODUCTION

EMICONDUCTOR lasers with an external cavity (ECSLs)

have been considered as rich sources of optical chaos, with

well-known chaotic regimes such as the so-called coherence

collapse [1] and low-frequency ﬂuctuation (LFF) regimes [2].

In these regimes, ECSLs have received considerable attention

Manuscript received July 24, 2008; revised October 29, 2008. Current version

published June 10, 2009. The work of D. S. Citrin was supported in part by the

National Science Foundation under Grant ECCS 0523923.

D. Rontani is with the Ecole Supérieure d’Electricité (Supélec), Laboratoire

Matériaux Optiques, Photonique et Systèmes (LMOPS), Centre National de

la Recherche Scientiﬁque (CNRS), F-57070 Metz, France and with the Unité

Mixte Internationale UMI 2958 GeorgiaTech-CNRS, F-57070 Metz, France.

He is also with the School of Electrical and Computer Engineering, Georgia

Institute of Technology, Atlanta, GA 30332 USA (e-mail: damien.rontani@su-

pelec.fr).

A. Locquet is with the Unité Mixte Internationale UMI 2958 GeorgiaTech-

CNRS, F-57070 Metz, France (e-mail: alocquet@georgiatech-metz.fr).

M. Sciamanna is with the Ecole Supérieure d’Electricité (Supélec), Labora-

toire Matériaux Optiques, Photonique et Systèmes (LMOPS), Centre National

de la Recherche Scientiﬁque (CNRS), F-57070 Metz, France and with the

Unité Mixte Internationale UMI 2958 GeorgiaTech-CNRS, F-57070 Metz,

France (e-mail: marc.sciamanna@supelec.fr).

D. S. Citrin is with the School of Electrical and Computer Engineering,

Georgia Institute of Technology, Atlanta, GA 30332 USA and with the Unité

Mixte Internationale UMI 2958 GeorgiaTech-CNRS, F-57070 Metz (e-mail:

david.citrin@ece.gatech.edu).

S. Ortin is with the Instituto de Física de Cantabria, Consejo Superior de In-

vestigaciones Cientíﬁcas (CSIC)-Universidad de Cantabria, Santander E-39005,

Spain (e-mail: ortin@ifca.unican.es).

Digital Object Identiﬁer 10.1109/JQE.2009.2013116

since they constitute key elements of optical secure chaotic com-

munications [3]. Indeed, the addition of an external cavity in-

troduces an inﬁnite number of degrees of freedom through the

time delay in the dynamical representation of the semiconductor

laser, leading to the generation of high-dimensional chaos [4].

The unpredictable and noisy appearance of the signals emitted

by hyper-chaotic optical systems has been used to enhance se-

curity and privacy in communications. A chaotic carrier con-

ceals and transmits an information bearing message, which is

decrypted by synchronization at the receiver end (a physical

copy of the chaotic emitter) [3], [5]–[8]. The security of such

a chaos-based communication scheme relies mainly on the dif-

ﬁculty to identify the emitter parameters and on the sensitivity

of synchronization to parameter mismatch [9]. Therefore, it is

of key importance to study the feasibility of an eavesdropper to

retrieve information on the parameters of a given chaotic gener-

ator from its transmitted time series.

In delayed hyper-chaotic systems, the security assump-

tion relies on the computational complexity to reconstruct

a high-dimensional attractor from the time series. However,

the knowledge of the time delay allows for the projection

of the high-dimensional attractor onto a reduced-dimen-

sional phase space, which makes the system vulnerable to

low-computational-complexity identiﬁcation techniques [10].

It is thus crucial that the delay not be easily identiﬁable in

a cryptosystem. Until recently, ECSLs with a single optical

feedback were considered as weakly secure systems in terms

of time-delay identiﬁcation, such that the use of several ex-

ternal cavities has been suggested [12]. However, we have

shown recently that a simple ECSL with a single optical

feedback could, with a careful choice of parameters, hide its

time-delay signature when standard estimators are employed

[13]. Interestingly, the region of laser and feedback parameters

where such a difﬁcult time-delay identiﬁcation occurs does

not necessarily correspond to the situation where the chaos

complexity (dimension and entropy) is higher [4]. Indeed, in

laser diodes with optical feedback, high-dimensional chaos is

typically found where the optical feedback strength is large, but

then the time-delay value is easily retrieved from the analysis

of the chaotic output using straightforward techniques [13].

Time-delay identiﬁcation should therefore be considered as

an additional argument to appreciate the level of security of

chaos-based communications, besides chaos complexity and

robustness of chaos synchronization.

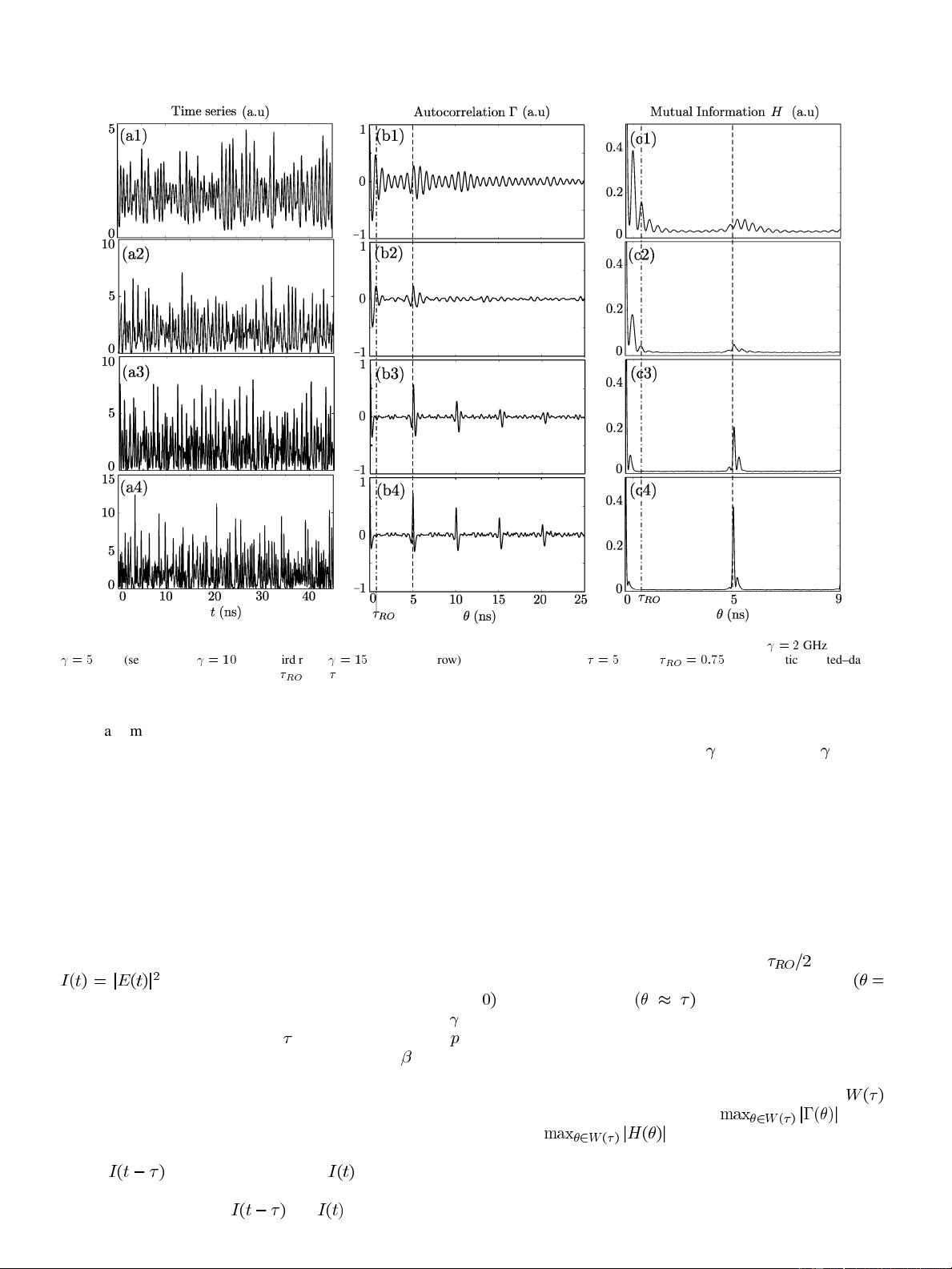
In this paper, we extend our earlier work on time-delay iden-

tiﬁcation in ECSL. Conventional techniques such as autocorre-

Authorized licensed use limited to: Marc Sciamanna. Downloaded on June 18, 2009 at 10:37 from IEEE Xplore. Restrictions apply.

880 IEEE JOURNAL OF QUANTUM ELECTRONICS, VOL. 45, NO. 7, JULY 2009

lation function (ACF) and delayed mutual information (DMI)

are used to process the identiﬁcation. We analyze further the

range of laser and feedback parameters that lead to difﬁcult

time-delay identiﬁcation and link these bad identiﬁcation sce-

narios to the laser dynamics as it undergoes a bifurcation cas-

cade to optical chaos. More speciﬁcally, we show that the time-

scales of the laser dynamics in its route to chaos inﬂuence the

time-delay identiﬁcation and therefore also the security of such

a chaotic emitter. A careful tuning of the external cavity round-

trip time with respect to the intrinsic laser relaxation oscillation

frequency leads to situations where the time-delay signature is

lost in the laser chaotic output. Finally, we discuss the robustness

of our results using other signal processing techniques applied

to the laser chaotic time-series.

This paper is organized as follows. Section II presents the

model and speciﬁcities of the various identiﬁcation techniques

used to retrieve the time-delay value. Section III details the inﬂu-

ence of the different operational parameters (the feedback rate,

the time delay and the pumping current) on the identiﬁcation.

Section IV gives a dynamical interpretation of the identiﬁca-

tion scenarios observed, based on the studies of the frequencies

that appear in the ECSL dynamics as it undergoes a route to

chaos. Section V discusses the inﬂuence of key internal param-

eters that modify the ECSL model and the possible application

of our results to chaos-based communications. Finally, we sum-

marize our main conclusions in Section VI.

II. T

HEORETICAL FRAMEWORK

A. Rate Equation Model

In this study, we consider a single-mode semiconductor laser

with optical feedback modelled by the Lang–Kobayashi rate

equations [14]. This model has been successful in reproducing

dynamical behaviors experimentally observed in ECSLs. The

rate equations are

(1)

(2)

where

is the slowly varying complex elec-

tric ﬁeld,

is the average carrier density in the active region,

is the linewidth-enhancement factor that describes the ampli-

tude phase coupling,

the optical gain where

is the saturation coefﬁcient, is the

carrier density at transparency,

is the angular frequency of

the solitary laser,

is the feedback rate, is the photon life-

time,

is the carrier lifetime, is the threshold current,

is the pumping factor, and is the delay corresponding to the

round-trip time of light in the external cavity. The Langevin

force

models the spontaneous-emission noise. Its polar de-

composition in amplitude and phase is given by the two terms

and

, where is the spontaneous-emis-

sion rate. The variables

and represent uncorre-

lated white Gaussian noises that satisfy the relations

and

and [15]. The relaxation-oscillation period

is an intrinsic damping time of the free-running laser and is

deﬁned by

with

. We consider the following pa-

rameter values:

, rad, ps, ns,

m s , m ,

m s , m , and s .

B. Time-Delay Estimator and Time-Delay Signature

The time delay is a key parameter for the security of non-

linear time-delay systems. Indeed, its estimation is of particular

importance with regard to computationally efﬁcient identiﬁca-

tion of other parameters. Standard techniques, such as the ACF

and the DMI, can be used to identify the time delay [16].

1) Autocorrelation Function (ACF): If a random process

is ergodic and wide-sense-stationary, then the ACF is de-

ﬁned by

(3)

where

and are sampled from the random process

, , and , where de-

notes time average. The ACF measures for a given value

the

tendency of the cloud of points

to be aligned

along a straight line and thus measures a linear relationship be-

tween

and .

2) Delayed Mutual Information (DMI): The mutual infor-

mation

is a quantity originally used in information theory

[17]. Given two continuous variables

and with joint proba-

bility density function

and marginal probability den-

sity functions

and , the mutual information of

and is deﬁned as

(4)

where

is the expectancy operator, the two variables and

are obtained by sampling the random process at two

times

and , and such a process is assumed to be stationary

and ergodic. The probability density functions

, , and

will be estimated by their respective histogram , , and

computed from the time series and leading to the approximate

mutual information estimator, also called delayed mutual infor-

mation (DMI)

(5)

The mutual information corresponds intuitively to the quan-

tity of information that the two random variables

and

are sharing. In time-delay systems, the presence of a

delayed feedback term induces a nonlocal time dependence in

the time evolution of its state variables. The integral deﬁnition

of the estimators under consideration allows for the detection of

Authorized licensed use limited to: Marc Sciamanna. Downloaded on June 18, 2009 at 10:37 from IEEE Xplore. Restrictions apply.

RONTANI et al.: TIME-DELAY IDENTIFICATION IN A CHAOTIC SEMICONDUCTOR LASER WITH OPTICAL FEEDBACK: A DYNAMICAL POINT OF VIEW 881

Fig. 1. ECSL intensity time series (ﬁrst column), ACF (second column), and DMI (third column) for increasing value of the feedback rate



GHz (ﬁrst row),



GHz (second row),



=10

GHz (third row),



=15

GHz (fourth row) with a time-delay value



ns and



ns. The vertical dotted–dashed

and dashed lines give the time location of



and



, respectively.

nonlocal time dependencies, linear for the ACF and nonlinear

for the DMI. Therefore, if a particular time scale, such as a

time delay, is present in a given time series, it should manifest

itself in the estimator through a local extremum. This signature

of the time scale, depending on its sign, will be referred to

as peak or valley later on in this article. The time location of

a peak (respectively, valley) will be considered as a possible

estimation of the time delay.

III. I

DENTIFICATION OF TIME DELAY IN

LANG–KOBAYASHI EQUATIONS

Here, the security of a chaotic ECSL is investigated. A time-

delay identiﬁcation is performed on the intensity time-series

using ACF and DMI. The evolution of the re-

sulting time-delay signatures are analyzed with the variation

of the following operational parameters: the feedback rate

the external-cavity round-trip time

, and the pumping factor .

Throughout this study, the spontaneous-emission rate

is taken

equal to zero.

A. Inﬂuence of the Feedback Rate

The feedback rate controls the optical power reinjected in the

laser cavity and hence drives the contribution of the delayed in-

tensity

in the time-evolution of . We thus expect

that the stronger the feedback rate, the more important the in-

formation shared between

and will be.

Fig. 1 analyzes a ﬁrst case of time-delay identiﬁcation for in-

creasing values of the feedback rate

. Large values of produce

chaotic time series with a clear time-delay signature: a signiﬁ-

cant peak is present in both the ACF [Fig. 1(b4)] and the DMI

[Fig. 1(c4)], at a time corresponding to the time delay. Sim-

ilar results have been obtained experimentally, from the anal-

ysis of the ACF [11]. The progressive decrease in feedback

rate produces the following: ﬁrst, a decrease of the peak am-

plitude close to the time-delay location and its multiples, in

both the ACF and DMI [Fig. 1(b3) and c(3)] until it reaches

a minimum value [Fig. 1(b2) and (c2)]; second, a qualitative

change of behavior appears. For relatively weak feedback rates,

numerous peaks and valleys, separated by

from each

other, appear and are ampliﬁed in the vicinity of the origin

and the time delay [Fig. 1(b1) and (c1)]. They

complicate the time-delay identiﬁcation. A quantitative descrip-

tion of the feedback-rate inﬂuence in terms of time location

and amplitude of the time-delay signature is given in Fig. 2

for both the ACF and the DMI. Fig. 2 is obtained by consid-

ering the most signiﬁcant peak (or valley) in a vicinity

of the time delay which is deﬁned by for the

ACF and

for the DMI. In Fig. 2, the curves

with triangle markers are drawn for the same parameters as the

ones of Fig. 1. The curves conﬁrm the tendency already ob-

served in Fig. 1: as the feedback rate is decreased, the ampli-

tude starts to diminish until a global minimum value is reached,

Authorized licensed use limited to: Marc Sciamanna. Downloaded on June 18, 2009 at 10:37 from IEEE Xplore. Restrictions apply.

882 IEEE JOURNAL OF QUANTUM ELECTRONICS, VOL. 45, NO. 7, JULY 2009

Fig. 2. Impact of the feedback variation and pumping factor on the amplitude and time location of the most signiﬁcant peak in the vicinity

(



)=[4

;

]

of the time delay



ns. (a1)–(b1) gives, respectively, the amplitude and time location of

max



)

(a2)–(b2) gives respectively the amplitude and

time location of

max

(



)

. The solid lines with triangle

(

)

, round

(



)

, and square

( )

markers stands for

and

respectively. These three different values of

, correspond to the following relaxation oscillation periods:



ns,



ns and



ns,

respectively. In (b1)–(b2), the dotted–dashed lines give the time location of the time delay



and then it increases [Fig. 2(a1)–(a2)]. The decreasing region

of the curve corresponds to a weak chaotic regime where the

laser’s intrinsic nonlinearity and feedback terms have equiva-

lent driving actions. In these conditions, the ACF and DMI may

still ﬁnd structural relationships within the intensity time se-

ries

. However, larger values of lead to well established

chaotic regimes. In such case, most of the time-scale signatures

are weakened except for the time delay which is favored by the

linear driving action of the feedback term

the rate equations. The decrease in

also inﬂuences the time

location of the largest peak at a time close to the time delay;

see Figs. 2(b1)–(b2). For small feedback rates, the largest au-

tocorrelation or mutual information is obtained for a time close

to a high order multiple of

, when analyzing a small time

window in the vicinity of the time delay

. Then, the iden-

tiﬁcation gives a value of time which is quite shifted from the

expected value

. However, as the feedback rate increases,

the greatest autocorrelation and mutual information values are

achieved for a time closer to the time delay. This explains the

monotonic decreasing behavior of the time shift identiﬁcation

curve in Fig. 2(b1)–(b2).

In summary, this ﬁrst analysis shows that, when the feed-

back rate is relatively weak, the chaotic output of the laser diode

shows only small evidence of the time-delay signature, both in

the ACF or the DMI. The largest contribution to the ACF or

DMI, in a time window around the time delay, is in fact closer

to a high multiple of the relaxation oscillation period

, and

this blurs the good identiﬁcation of the expected value

Fig. 2 also shows the inﬂuence of the pumping factor

, which

controls the injection current

as well as the value

of the relaxation oscillation period

. When increasing ,

chaotic dynamics are observed for larger values of the feedback

rate

but similar conclusions hold for the identiﬁcation of the

time delay. The amplitude of the highest peak (or valley) in the

ACF and DMI in the vicinity of

exhibits a minimum value

when the feedback rate increases, and the feedback rate cor-

responding to this minimum increases with the increase of

Moreover, the time value obtained from the ACF or DMI iden-

tiﬁcation techniques is shifted from the expected value

when

the feedback rate is small but gets closer to

as the feedback rate

increases. However, the maximum time shift is smaller when

increases, hence progressively leading to a better identiﬁcation

B. Inﬂuence of the Time Delay Relatively to the

Relaxation-Oscillation Period

Here, we study how the value

affects the time-delay iden-

tiﬁcation based on the ACF and DMI. This inﬂuence is ana-

lyzed relatively to the value of

, the other signiﬁcant ECSL

time scale in terms of identiﬁcation. This line of reasoning is

Authorized licensed use limited to: Marc Sciamanna. Downloaded on June 18, 2009 at 10:37 from IEEE Xplore. Restrictions apply.

评论收藏

内容反馈

版权申诉

yixiangzhe1

2023-02-24

资源有一定的参考价值，与资源描述一致，很实用，能够借鉴的部分挺多的，值得下载。
wox_iuyehxjs9

2024-07-29

支持这个资源，内容详细，主要是能解决当下的问题，感谢大佬分享~
fdf@-@

2021-11-24

用户下载后在一定时间内未进行评价，系统默认好评。
weixin_45120100

2023-03-12

资源内容详实，描述详尽，解决了我的问题，受益匪浅，学到了。
彩虹小马285

2024-01-19

资源有很好的参考价值，总算找到了自己需要的资源啦。

前往

页

心梓

粉丝: 849
资源: 8042

DFB laser_DFBlaser_DFB速率方程_速率方程_DFB_求解速率方程

DFB laser_DFBlaser_DFB速率方程_速率方程_DFB_求解速率方程_源码.rar

DFB laser_DFBlaser_DFB速率方程_速率方程_DFB_求解速率方程.zip

matlab_DFB_

dbf.rar_DFB_DFB激光器_dfb激光器数值模拟程序_激光_激光器 动力学

10GHzDFB.rar_DFB激光器_diode laser_semiconductor diode_semiconducto

激光器速率方程matlab程序

DFBlaser窄线宽激光器

UnityPro--第08章__DFB和诊断_DFB

冰河的渗透实战笔记-冰河.pdf

stm32f103 adc采样+dma传输+fft处理 频率计_fft处理_stm32_ADCFFT_频率计_ADC采样_

ISO21434.pdf

Web安全漏洞扫描工具-AWVS14

J-LINK V10 V11固件.rar

CTF 竞赛入门指南（ctf-all-in-one）.pdf

Web中间件常见漏洞总结.pdf

jts-1.14.zip

DEAP2.1.zip_DEA2.1软件下载_dea 2.1软件下载_deap2.1_deap2.1基础模型_dea模型

CobaltStrike4.4.zip

RK3568硬件设计资料.zip_C#

数据结构与算法分析--C语言描述_数据结构与算法_

cisp-pte渗透测试资源下载 （考试环境+题库）

QT帮助文档_中文版_QT中文版帮助文档_

goby2021红队专版，1.8.255

pconline1478255959502.rar

海康威视配置文件解码专用工具器.rar

内网渗透攻击线路图.pdf

AWD之赛前培训.pptx

苹果越狱解ID博客中提到的所有工具集.zip

APPinvent，蓝牙软件源文件

最新资源

dbf.rar_DFB_DFB激光器_dfb激光器数值模拟程序_激光_激光器动力学

stm32f103 adc采样+dma传输+fft处理频率计_fft处理_stm32_ADCFFT_频率计_ADC采样_

cisp-pte渗透测试资源下载（考试环境+题库）