CMB对α吸引子充气中充气联轴器和再热温度的约束资源-CSDN文库

Open

Access

168 浏览量 2020-03-24 02:39:38 上传评论收藏 1.83MB PDF 举报

资源推荐

资源详情

资源评论

JHEP11(2017)072

Published for SISSA by Springer

Received: August 11, 2017

Accepted: October 28, 2017

Published: November 14, 2017

CMB constraints on the inﬂaton couplings and

reheating temperature in α-attractor inﬂation

Marco Drewes,

a,d

Jin U Kang

b,c

and Ui Ri Mun

Physik Department T70, Technische Universit¨at M¨unchen,

James Franck Straße 1, D-85748 Garching, Germany

Abdus Salam International Centre for Theoretical Physics,

Strada Costiera 11, Trieste 34014, Italy

Department of Physics, Kim Il Sung University,

RyongNam Dong, TaeSong District, Pyongyang, D.P.R. Korea

Centre for Cosmology, Particle Physics and Phenomenology,

Universit´e catholique de Louvain,

Louvain-la-Neuve B-1348, Belgium

E-mail: marco.drewes@uclouvain.be, jkang@ictp.it, uirimun@gmail.com

Abstract: We study reheating in α-attractor models of inﬂation in which the inﬂaton

couples to other scalars or fermions. We show that the parameter space contains viable

regions in which the inﬂaton couplings to radiation can be determined from the properties

of CMB temperature ﬂuctuations, in particular the spectral index. This may be the only

way to measure these fundamental microphysical parameters, which shaped the universe

by setting the initial temperature of the hot big bang and contain important information

about the embedding of a given model of inﬂation into a more fundamental theory of

physics. The method can be applied to other models of single ﬁeld inﬂation.

Keywords: Cosmology of Theories beyond the SM, Thermal Field Theory

ArXiv ePrint: 1708.01197

Open Access,

 The Authors.

Article funded by SCOAP

https://doi.org/10.1007/JHEP11(2017)072

JHEP11(2017)072

of these temperature ﬂuctuations has conﬁrmed several predictions of cosmic inﬂation [5].

This makes the idea that the observable universe underwent a phase of accelerated cosmic

expansion during its very early history very appealing. However, it is not known what was

the driving force behind this rapid acceleration. Moreover, inﬂation dilutes matter and

radiation and leaves a cold and empty universe. In contrast to that, the good agreement of

the observed light element abundances with the predictions from big bang nucleosynthesis

(BBN) indicates that the universe was ﬁlled with a dense medium of relativistic particles

in thermal equilibrium, which we in the following refer to as “radiation”, and puts a lower

bound of roughly 10 MeV on the temperature in the early universe [6]. Thus any viable

theory for cosmic inﬂation should address at least following two questions:

I) What mechanism drove the inﬂationary growth of the scale factor?

II) How did the transition to the hot radiation dominated epoch occur?

These are fundamentally important questions not only for cosmologists, but also for particle

physicists, who would like to understand how the idea of inﬂation can be embedded into

a more general theory of nature. In the present work, we focus on the second question

above. Regarding the ﬁrst question, we adopt the viewpoint that inﬂation was caused by

a scalar inﬂaton ﬁeld φ with a ﬂat potential, which dominated the energy density of the

universe and led to a negative equation of state. There exist many models of this single

ﬁeld inﬂation, see e.g. ref. [7] for a partial overview.

The rapid expansion during inﬂation diluted all pre-inﬂationary matter and radia-

tion, leaving a cold and empty universe. The transition to the radiation dominated epoch

occurred when the inﬂaton’s energy density was transferred into relativistic particles via

dissipative eﬀects, see ref. [8] for a recent review. This process is called cosmic reheating.

The reheating process lasts for a ﬁnite amount of time, which should be regarded as a

separate era in the cosmic history, i.e. the reheating era. The most important eﬀect of

the reheating era on the CMB lies in the modiﬁed expansion rate, which is illustrated

in ﬁgure 1. This aﬀects the red-shifting of cosmological perturbations, and therefore the

relation between physical scales of the CMB mode at the present time and at Hubble cross-

ing of the modes during inﬂation.

This eﬀect can be parametrised by a single number

rad

[14, 15], which can be expressed in terms of the averaged equation of state during

reheating ¯w

and the ratio of the cosmic energy densities at the end of inﬂation ρ

end

and

reheating ρ

ln R

rad

1 − 3 ¯w

12 (1 + ¯w

)



end



, (1.1)

or in terms of N

, the number of e-folds from the end of inﬂation until the end of

reheating, as

ln R

rad

(3 ¯w

− 1) . (1.2)

We use the term reheating in the general sense described below, which includes a possible “preheating”

phase.

Other possible signatures of reheating in the CMB include non-Gaussianities [9, 10] and curvature-

perturbations [11–13].

– 2 –

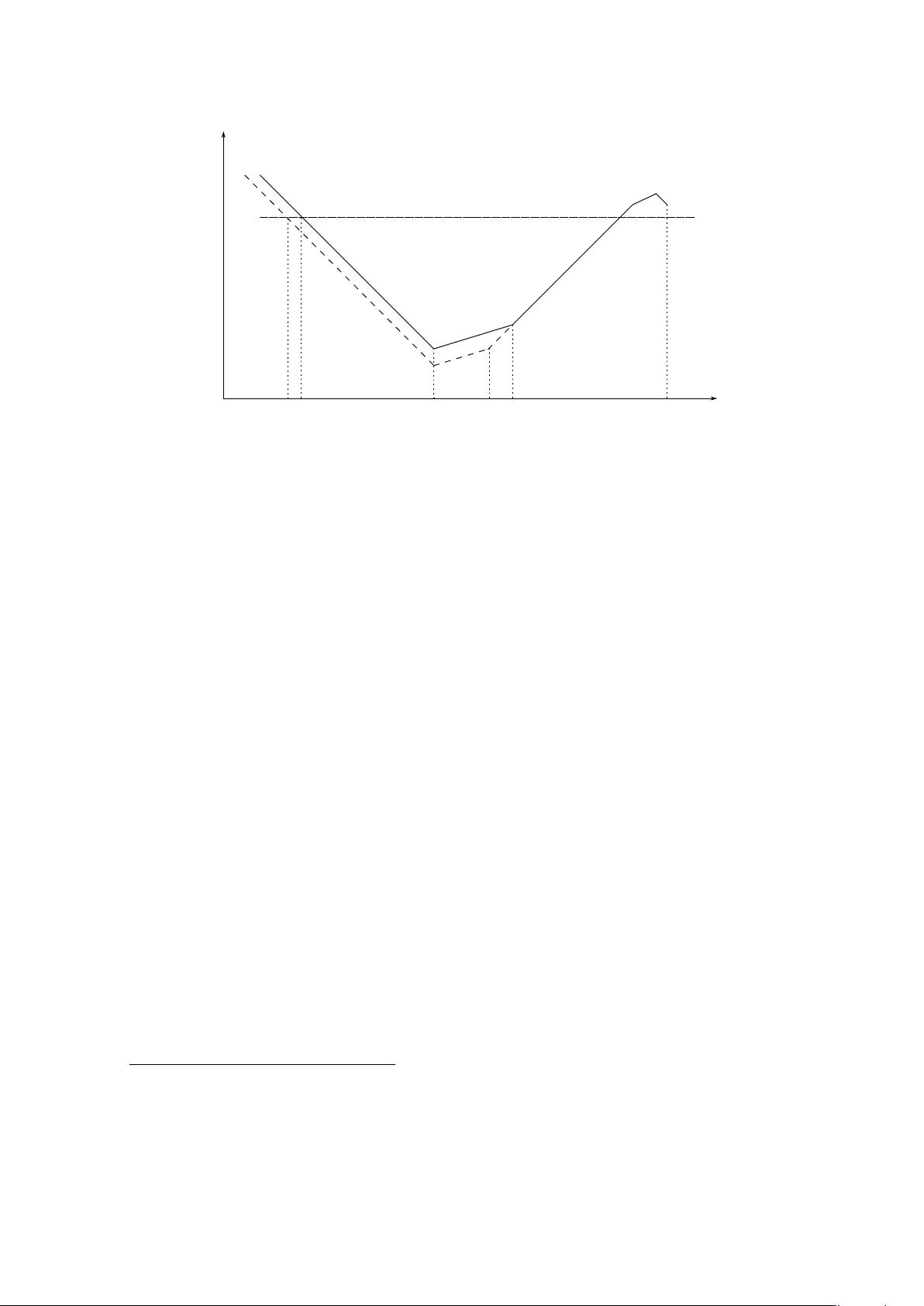
JHEP11(2017)072

Inflation

Reheating

Comoving length scale

end

ln (aH)

-1

ln a

Figure 1. Evolution of the comoving Hubble horizon in diﬀerent epochs of cosmic history. RD, MD

and Λ indicate the radiation dominated era, matter dominated era and the current era of accelerated

cosmic expansion, respectively. H is Hubble parameter and a the scale factor. a

, a

end

, a

and a

are the values of the scale factor at the horizon crossing of a reference mode with a comoving wave

number k, the end of inﬂation, the end of reheating and the present time, respectively. The diﬀerent

slopes are a result of the diﬀerent equation of state in diﬀerent epochs. In order to visualise the eﬀect

of the reheating era on the horizon crossing point, we used two line styles: solid line corresponds to

the small dissipation rate Γ, i.e. the large e-fold number of reheating N

and dashed line corresponds

to the relatively large value of Γ, i.e. small N

. In both cases we have assumed that the equation

of state parameter w

remains approximately constant during reheating; if this were not the case,

the slope of the lines would change during reheating. Our analysis, however, does not rely on this

assumption because the eﬀect on the CMB only depends on the average value ¯w

, cf. eq. (1.1).

For the larger Γ, the end of reheating lies further back in time. As a result, the inferred values for

the scale factor at that moment and at the moment of horizon crossing decrease, as can be seen by

comparing a

and a

to a

and a

. This implies that the horizon crossing happens at the larger

ﬁeld value φ

if Γ is larger, where the slow-roll parameters are more suppressed, and hence the

spectral index n

gets closer to 1. Conversely, a larger n

implies a larger Γ. Assuming that Γ is

a monotonically increasing function of the inﬂaton’s coupling constant to radiation, the coupling

constant is an increasing function of n

If ¯w

can be somehow ﬁxed, which is often the case in a given model, then N

or ρ

can be used instead of R

rad

to parametrise the eﬀect of reheating on the CMB. In ref. [16]

it has been shown that the CMB indeed contains enough information to treat R

rad

a meaningful independent ﬁt parameter when constraining models of inﬂation. In view

of various upcoming CMB observations,

this provides strong motivation to study the

potential of these measurements to say something about reheating.

The derivation of constraints on inﬂationary models from CMB observations in prin-

ciple requires knowledge of R

rad

, which depends not only on the inﬂaton potential, but

An overview of realistic sensitivities of various proposed experiments can be found in ref. [17]. The

CORE collaboration has already studied their potential to gather information about the reheating pe-

riod [18].

– 3 –

JHEP11(2017)072

also on the interactions between φ and the radiation. The lack of knowledge about these

interactions imposes a systematic uncertainty on the derived constraints [19, 20], which can

be quantiﬁed by the deviation of R

rad

from unity. One may, however, turn the tables and

use this dependency to impose constraints on the reheating epoch [15, 16, 21–36]. Most of

the previous works on CMB constraints on the reheating epoch has focused on constraints

on the macroscopic parameters such as N

, ¯w

and the reheating temperature T

at the

onset of the radiation dominated era. However, from the viewpoint of particle physics it is

interesting to derive constraints on microphysical parameters, such as inﬂaton coupling to

radiation. This is the main goal of the present paper.

In ref. [37] it has been pointed out that constraints on R

rad

can be converted into

constraints on the inﬂaton couplings to radiation, and that this relation can have a simple

analytic form if reheating is primarily driven by perturbative processes. In the present

work, we apply this idea to α-attractor models [38–43] that cover a wide class of inﬂationary

scenarios. A lower bound on the inﬂaton coupling in this class of models has previously

been studied in ref. [27], where it was assumed that the inﬂaton couples to another scalar

ﬁeld χ via the interaction of the type gφχ

. We extend this analysis to diﬀerent kinds of

interactions and include the feedback of the produced radiation on the reheating process,

which can severely limit the range of validity of the results. This is indeed a non-trivial

requirement. If the coupling constant is too large, the particle production is eﬃcient enough

to trigger a parametric resonance, and perturbative techniques cannot be applied. For very

small values, reheating is not eﬃcient enough to heat the universe to temperatures above

10 MeV, which is required for consistency with BBN. We also show that thermal corrections

to the perturbative decay do not aﬀect the CMB constraints in the perturbative regime.

This allows us to establish analytic relations between the inﬂaton couplings and observable

quantities, and to identify their range of applicability.

This article is organised as follows. In section 2 we brieﬂy review the CMB-constraints

on reheating and set up the theoretical framework and describe the methodology adopted

in this work. In section 3 we apply this to derive constraints on interactions via which

the inﬂaton φ may couple to other scalars χ or fermions ψ. We consider interactions of

the form gφχ

, hφχ

and yφ

ψψ and establish relations between the coupling constants g,

h and y and CMB observables. These relations hold in the parameter regime in which

reheating is entirely driven by perturbative processes. We show that there exists a range

of values of the coupling constants for which this is the case. We conclude in section 4. In

the appendix, we present the expressions for dissipation rates used in the main text.

2 Reheating in α-attractor E-model

2.1 General considerations

The expectation value of the inﬂaton ﬁeld ϕ ≡ hφi is often assumed to follow an equation

of motion of the form

¨ϕ + (3H + Γ) ˙ϕ + ∂

V(ϕ) = 0, (2.1)

– 4 –

剩余41页未读，继续阅读

评论收藏

内容反馈

weixin_38735182

粉丝: 5
资源: 920

CMB对α吸引子充气中充气联轴器和再热温度的约束

U（1）对称α吸引子

CMB异常和充气潜力的局部特征的影响

通过重新加热将CMB各向异性与冷暗物质现象学联系起来

当前CMB极化数据对原始引力波和极化旋转角的联合约束。

来自CMB和中微子振荡测量的无菌中微子约束的组合视图

沼泽距离猜想，通货膨胀和α吸引子

5D SUGRA产生的自然膨胀和较低的再热温度

CMB的慢速充气和BB模式角功率谱

用CMB光谱调查互相关约束电离气体的释放

美国环境保部署epa-cmb82安装包+用户手册+中文翻译.7z

SN Ia和BAO / CMB观测对重构暗能量模型的约束

暗物质模型，调和了宇宙射线e±过量与伽马射线和CMB约束之间的张力

沼泽地猜想后的再加热

α′通货膨胀：模态稳定和高阶导数的可观测张量

双引力耦合的宇宙学：来自SNIa，BAO和CMB的约束

对C波消除暗物质的强CMB约束

CFT驱动的宇宙学中的充气起因：R2-重力和非最小耦合的Inflaton模型

化学质量平衡模型EPA-CMB82

大张量模式的充气重生

多重通胀情景下的CMB频谱失真

CMB搜索中微子质量机制及其与哈勃张力的关系

受体模型EPA-CMB82

在修正的Teleparallel通胀模型中进行再加热

用CMB数据约束暗光子及其与21 cm宇宙学的联系

最新资源