没有合适的资源?快使用搜索试试~ 我知道了~
在最小d = 6 F(4)规范超重力范围内派生出一类新的精确超对称解决方案。 这些流动的全部特征是超重力多重峰中包含的2形表形电势的非平凡径向轮廓。 特别是,此类中的三个解决方案的特点是6d背景的AdS3叶片和AdS6渐近几何。 其次,考虑其中最简单的示例,我们给出了其大量的IIA隆起,描述了在两个间隔I r×Iξ上纤维化的AdS3×S 2×S 3类型的翘曲解。 我们将这种背景解释为D4-D8系统的近视水平,其结合状态D2-NS5-D6结束时会产生表面缺陷。 最后,我们根据N $$ \ mathcal {N} $$ = 2对AdS6对偶的SCFT5的N $$ \ mathcal {N} $$ =(0,4)SCFT2缺陷理论来讨论其全息双重解释。 S 4大型IIA使真空弯曲。
资源推荐
资源详情
资源评论

JHEP01(2019)193
Published for SISSA by Springer
Received: November 11, 2018
Accepted: December 20, 2018
Published: January 25, 2019
Surface defects in the D4-D8 brane system
Giuseppe Dibitetto
a
and Nicol`o Petri
b
a
Institutionen f¨or fysik och astronomi, University of Uppsala,
Box 803, SE-751 08 Uppsala, Sweden
b
Department of Mathematics, Bo˘gazi¸ci University,
34342, Bebek, Istanbul, Turkey
E-mail: giuseppe.dibitetto@physics.uu.se, nicolo.petri@boun.edu.tr
Abstract: A new class of exact supersymmetric solutions is derived within minimal d = 6
F (4) gauged supergravity. These flows are all characterized by a non-trivial radial profile
for the 2-form gauge potential included into the supergravity multiplet. In particular three
solutions within this class are featured by an AdS
3
foliation of the 6d background and by
an AdS
6
asymptotic geometry. Secondly, considering the simplest example of these, we
give its massive IIA uplift describing a warped solution of the type AdS
3
×S
2
×S
3
fibered
over two intervals I
r
× I
ξ
. We interpret this background as the near-horizon of a D4-D8
system on which a bound state D2-NS5-D6 ends producing a surface defect. Finally we
discuss its holographic dual interpretation in terms of a N = (0, 4) SCFT
2
defect theory
within the N = 2 SCFT
5
dual to the AdS
6
× S
4
massive IIA warped vacuum.
Keywords: AdS-CFT Correspondence, D-branes, Superstring Vacua
ArXiv ePrint: 1807.07768
Open Access,
c
The Authors.
Article funded by SCOAP
3
.
https://doi.org/10.1007/JHEP01(2019)193

JHEP01(2019)193
Contents
1 Introduction 1
2 The D4-D8 system and AdS
6
/CFT
5
3
2.1 Including a NUT charge 6
3 The supergravity setup 7
3.1 Minimal N = (1, 1) gauged supergravity in d = 6 8
3.2 AdS
6
vacuum and domain walls 10
3.3 The massive IIA origin of F (4) supergravity 11
4 BPS flows with the 2-form gauge potential 12
4.1 The general ansatz 13
4.2 Background with M
3
= R
1,2
and Σ
2
= R
2
14
4.3 Background with M
3
= AdS
3
and Σ
2
= R
2
16
4.4 Background with M
3
= R
1,2
and Σ
2
= S
2
18
4.5 Background with M
3
= AdS
3
and Σ
2
= S
2
19
5 Surface defects within the N = 2 SCFT
5
22
5.1 Charged domain wall and massive IIA uplift 22
5.2 Defect SCFT
2
and the AdS
3
× S
2
× S
3
× I
2
solution 23
5.3 One-point correlation functions 26
A Massive IIA supergravity 28
B Symplectic-Majorana-Weyl spinors in d = 1 + 5 29
C Gauged N = (1, 1) supergravities in six dimensions 30
1 Introduction
The most peculiar feature of the quantum string theory spectrum is the presence of ex-
tended objects of non-perturbative nature, which are referred to as branes. Therefore,
branes as such are the key to the non-perturbative aspects of string theory. Even if a lot of
progress has been made in this respect, all main insights in this direction are still coming
from the low-energy description of brane systems. For this reason, the search for new su-
persymmetric solutions within supergravity theories, as well as engineering novel examples
of SCFTs emerging from branes should be considered as the most practical, concrete and
predictive playgrounds for producing quantitative results concerning the physics of strings
propagating within ten dimensional spacetime.
– 1 –

JHEP01(2019)193
The aim of this paper is to take some further steps in this direction by considering
the holographic realization of defect conformal field theories arising from brane systems.
Generally speaking, these are CFTs defined on a defect hypersurface within the background
of a higher-dimensional bulk CFT [1–6]. From the point of view of this “mother” theory, the
presence of the defect is realized through a deformation associated to a position-dependent
coupling. This deformation turns out to partially break conformal invariance in the bulk,
while only preserving the conformal transformations leaving the defect CFT intact. As
an immediate consequence, the one-point correlation functions are no longer vanishing,
and a non-trivial displacement operator appears. This a sign of the fact that the energy-
momentum tensor needs not be conserved in the presence of the defect.
The first realizations of defect CFTs in string theory were constructed in [7]. Then
many other examples and applications followed (for a non-exhaustive list of references on
conformal defects in string theory and holography see [8–36]). The key idea is to let defect
CFTs emerge from some particular supersymmetric brane configurations in which some
“defect branes” end on a given brane system, which is known to give rise to an AdS vacuum
in the near-horizon limit. The main effect of these intersections is to break partially the
isometry group of the AdS vacuum of the original brane system and to produce a lower-
dimensional warped AdS solution. The defect CFT describes the boundary conditions
defining the intersection with the defect branes and the warping of the corresponding
background describes the backreaction of the defect onto the bulk geometry. This may be
viewed as the supergravity picture associated to the position-dependent deformation of the
“mother” SCFT, dual to the original higher-dimensional AdS vacuum.
More concretely, let us consider a SUSY AdS
d
closed string vacuum associated with the
near-horizon of some brane system, where we furthermore assume the existence of a con-
sistent truncation linking the 10d (or 11d) picture to a solution in a d-dimensional gauged
supergravity describing the excitations around the AdS
d
vacuum. If some defect branes
end on this system, then we have a bound state with a (p + 1)-dimensional worldvolume
whose physics is captured by a d-dimensional Janus-type background
ds
2
d
= e
2U(r)
ds
2
AdS
p+2
+ e
2V (r)
dr
2
+ e
2W (r)
ds
2
d−p−3
. (1.1)
The d-dimensional background is thus characterized by a AdS
p+2
slicing and an asymp-
totic region locally described by the AdS
d
vacuum.
1
The solutions like (1.1) can be then
consistently uplifted producing warped geometries of the type AdS
p+2
×M
d−p−2
×Σ
D−d
,
where M
d−p−2
is realized as a fibration of the (d −p −3)-dimensional transverse manifold
over the interval I
r
and Σ
D−d
is the internal manifold of the truncation with D = 10 or 11.
1
We point out that the main difference between this case and the one of RG flows across dimensions
can be observed by considering the “radial” coordinates giving rise to the AdS vacua respectively in the
UV and IR. In a conformal defect, the radii of the AdS
p+2
and AdS
d
are different, while in a supergravity
solution describing an RG flow across dimensions, the AdS backrounds arising in the UV and in the IR are
described by the same radial coordinate. Conformal defects and RG flows across dimensions are somehow
two complementary descriptions. For example one may guess the existence of more general flows involving
r as well as the radial coordinate of AdS
p+2
describing a geometry where the metric (1.1) is replaced by an
R
1,p
slicing of the d-dimensional background.
– 2 –

JHEP01(2019)193
From the point of view of the dual field theories, this is exactly the supergravity realization
of a defect SCFT
p+1
within the “mother” SCFT
d−1
.
In this paper we consider D4-D8 systems in massive IIA string theory and its intersec-
tion with D2-NS5-D6 defect branes. It is well-known that stacks of coincident D4 branes
localized on D8 branes and in the presence of O8 planes are described at the horizon by a
warped vacuum AdS
6
× S
4
[37]. The dual picture of this vacuum is realized by a matter-
coupled N = 2 SCFT
5
arising as a fixed point of the 5d quantum field theory living on
the worldvolume of the D4s [37–40]. For a non-exhaustive list of references on AdS
6
vacua
in string theory and AdS
6
/CFT
5
correspondence we will refer to [41–59], while in [60, 61]
some holographic RG flows across dimensions are studied which arise from spontaneous
wrapping of D4 branes.
Massive IIA string theory can be consistently truncated around the AdS
6
× S
4
vac-
uum [62] and the theory produced by this truncation is d = 6, N = (1, 1) gauged super-
gravity, also known as F (4) gauged supergravity [63]. The minimal incarnation of this
theory will be the main tool of this paper and, within this context, we will be able to de-
rive a new class of analytic BPS solutions characterized by a running profile for the 2-form
gauge potential included into the supergravity multiplet. This new class of flows will be
presented by starting from the simplest 6d background compatible with the presence of
the 2-form, to subsequently move to more complicated 6d geometries. The main results
are thus represented by three backgrounds of the type (1.1), namely, warped solutions of
the type AdS
3
×M
3
admitting a locally AdS
6
asymptotic geometry with a 2-form charge.
In particular one of these backgrounds is non-singular in the IR and, in this limit, the
geometry is locally given by AdS
3
× T
3
.
Among these warped AdS
3
× M
3
solutions, we then consider the simplest one, given
by a “charged” domain wall with a running profile for the 2-form and we interpret the
singular behavior appearing in the IR regime as a brane singularity associated to D2-NS5-
D6 defect branes ending on the D4-D8 system. The key point of this interpretation is
based on the presence of the 2-form that turns out to be related to the F
(4)
, F
(2)
and H
(3)
fluxes in the 10d picture. Thanks to the uplift formula, we then obtain the corresponding
10d backround written as AdS
3
× S
2
× S
3
fibered over two intervals I
r
× I
ξ
, where M
3
is
realized by an S
2
fibration over I
r
and the 4d squashed sphere defining the truncation is
written as an S
3
fibration over I
ξ
. Then we discuss the relations of the 10d background
AdS
3
× S
2
× S
3
× I
r
× I
ξ
with the near-horizon geometry of the brane intersection D2-
D4-NS5-D6-D8 found in [34] and we formulate the holographic interpretation of the 6d
charged domain wall as a defect N = (0, 4) SCFT
2
within the N = 2 SCFT
5
. Finally
we test this interpretation by deriving the one-point functions of the defect both from
holographic arguments and conformal perturbation expansion, and we find agreement in
the position-dependence for the coupling driving the deformation produced by the defect.
2 The D4-D8 system and AdS
6
/CFT
5
Let us consider the brane system discussed in [37, 39, 40]. The construction starts from a
probe five-brane brane in type I string theory on R
9
×S
1
whose worldvolume is wrapping
– 3 –
JHEP01(2019)193
branes t y
1
y
2
y
3
y
4
z ρ θ
1
θ
2
θ
3
D8 × × × × × − × × × ×
D4 × × × × × − − − − −
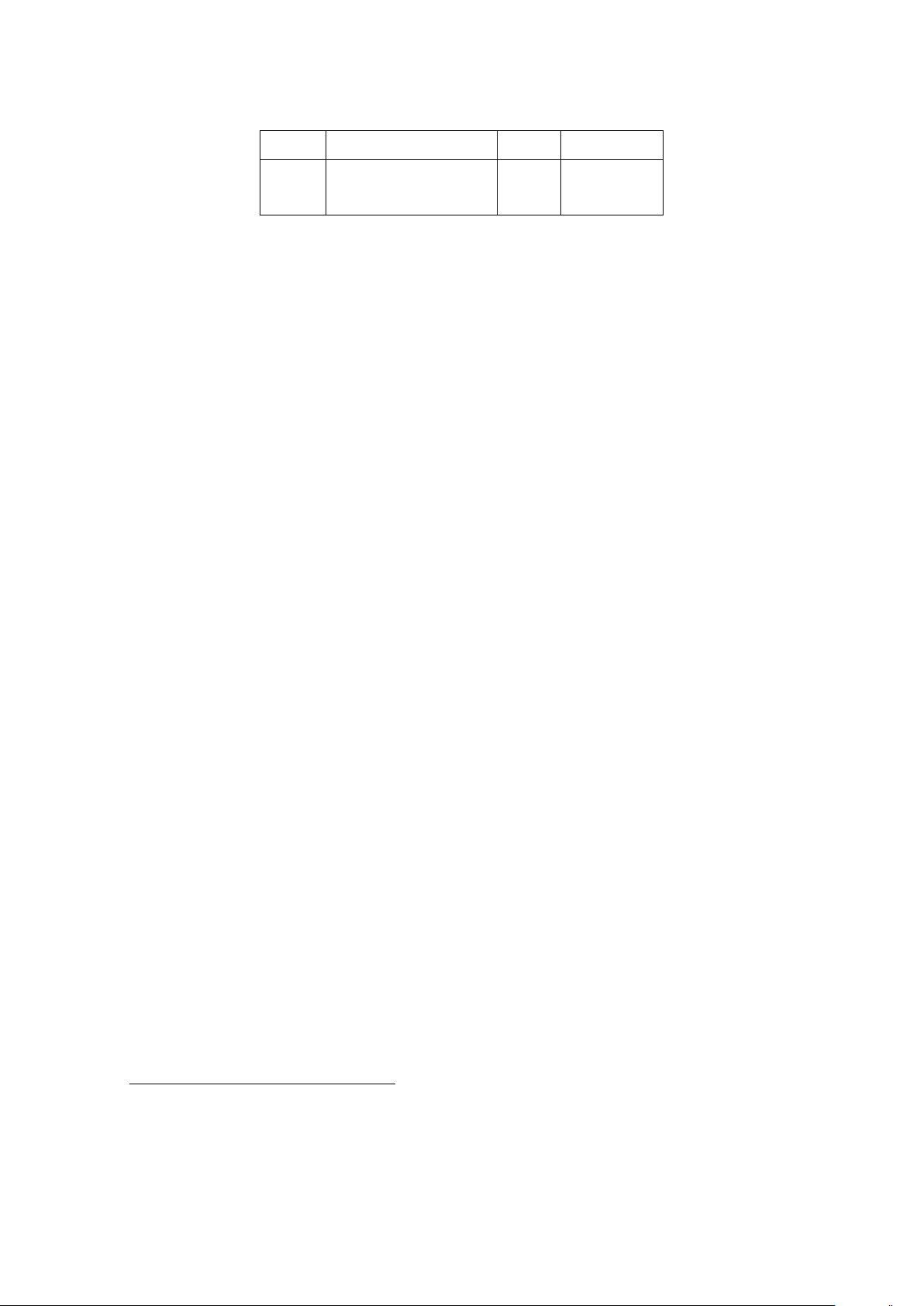
Table 1. The brane picture underlying the 5d N = 2 SCFT defined by the D4-D8 system. The
system is BPS/4 and the AdS
6
× S
4
vacuum is realized by a combination of ρ and z.
the circle. Performing a T-duality along the circle we obtain a four-brane in type I’ on
the interval S
1
/Z
2
with two O8 planes in the fixed points. Then the four-brane can be
interpreted as a D4 brane in massive IIA string theory located at a point of the interval. In
order to cancel the −16 charge units carried by the O8 planes, one has to include at least
16 D8 branes whose position is described by the moduli appeared after dualizing. Then a
slightly more general construction involving two D8 stacks can be considered, one of each
consisting of N
f
and 16 − N
f
D8 branes, respectively.
Let us now move to discussing the worldvolume theory of this construction. Within
the interval S
1
/Z
2
, the gauge group of the theory on the D4 brane is broken to U(1), but at
the two endpoints a larger gauge symmetry is restored. In particular, if the D4 and N
f
D8
branes are located at one orientifold and the other 16 −N
f
at the other O8, then we have a
d = 5 N = 2 Yang-Mills theory with gauge group SU(2). The 5d vector multiplet includes a
gauge field and a real scalar describing the locus of the D4 along S
1
/Z
2
. The matter content
is given by N
f
hypermultiplets in the fundamental, arising from open strings streched be-
tween the D4 and the D8 branes, and by an antisymmetric massless hypermultiplet coming
from the D4 brane. The supercharges and the scalars coming from the antisymmetric hy-
permultiplet transform as a doublet under the R-symmetry group, that is given by SU(2)
R
.
The global symmetry of the theory is SU(2) ×SO(2N
f
) ×U(1)
I
, where the SU(2) factor is
associated to the antisymmetric hyper, the SO(2N
f
) is related to the N
f
hypers in the fun-
damental and finally the extra U(1)
I
corresponds to the instanton number conservation.
2
The above construction can be extended to a stack of N coinciding D4 branes entirely
localized on the N
f
D8 branes at a 9-dimensional orientifold and other 16 −N
f
D8 branes
at the other O8 plane. In this case we have a N = 2 SYM theory with gauge group USp(N)
coupled to N
f
“quark” hypers and to an antisymmetric hyper.
If the number of flavors is such that N
f
< 8, the theory introduced above has a
non-trivial fixed point at the origin of the Coulomb branch, given by R
+
and the global
symmetry associated to the Higgs branch is then enhanced to SU(2) × E
N
f
+1
[39]. This
fixed point is described by a N = 2 SCFT
5
with USp(N ) gauge group and couplings to
matter given by N
f
fundamental and one antysimmetric hypermultiplets.
The low-energy description of the above brane system is naturally realized in massive
IIA supergravity.
3
It turns out that this construction includes an AdS
6
vacuum in its near-
horizon limit and this corresponds to a fixed point in the RG flow of the 5d worldvolume
theory of the D4 branes [37, 40]. Let us now consider the supergravity solution describing
2
It is related to the 5d conserved current ?
5
(F ∧ F ) [39, 40].
3
See appendix A for a brief review on massive IIA supergravity.
– 4 –
剩余36页未读,继续阅读
资源评论

weixin_38693753
- 粉丝: 9
- 资源: 993
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功