搜索在s=8TeV的质子-质子碰撞中衰变成四轻子最终态的疏疏Z'玻色子资源-CSDN文库

Open

Access

141 浏览量 2020-05-05 20:04:48 上传评论收藏 737KB PDF 举报

资源推荐

资源详情

资源评论

Physics Letters B 773 (2017) 563–584

Contents lists available at ScienceDirect

Physics Letters B

www.elsevier.com/locate/physletb

Search for leptophobic Z



bosons decaying into four-lepton ﬁnal states

in proton–proton collisions at

√

s = 8 TeV

.The CMS Collaboration



CERN, Switzerland

a r t i c l e i n f o a b s t r a c t

Article history:

Received

5 January 2017

Received

in revised form 30 April 2017

Accepted

24 August 2017

Available

online 6 September 2017

Editor:

M. Doser

Keywords:

LHC

CMS

Physics

Exotica



Four leptons

A search for heavy narrow resonances decaying into four-lepton ﬁnal states has been performed using

proton–proton collision data at

√

s = 8 TeV collected by the CMS experiment, corresponding to an

integrated luminosity of 19.7 fb

−1

. No excess of events over the standard model background expectation

is observed. Upper limits for a benchmark model on the product of cross section and branching fraction

for the production of these heavy narrow resonances are presented. The limit excludes leptophobic Z



bosons with masses below 2.5 TeV within the benchmark model. This is the ﬁrst result to constrain a

leptophobic Z



resonance in the four-lepton channel.

(http://creativecommons.org/licenses/by/4.0/). Funded by SCOAP

1. Introduction

Extensions of the standard model (SM) that incorporate one or

more extra Abelian gauge groups predict the existence of one or

more neutral gauge bosons [1,2]. These occur naturally in most

grand uniﬁed theories. Heavy neutral bosons are also predicted in

models with extra spatial dimensions [3,4], e.g. Randall–Sundrum

models [5,6], where these resonances may arise from Kaluza–

Klein

excitations of a graviton. Searches for heavy neutral reso-

nances

at hadron colliders, and most recently at the CERN LHC,

are typically performed using the dijet [7–10], dilepton [11–14],

diphoton [15–17], diboson [18–24], and tt [25–28] ﬁnal states.

The dilepton channel provides a clean signal compared with the

dijet and tt channels. However, in leptophobic Z



models, where

the Z



does not couple to SM leptons, the dilepton limits are not

applicable. Although searches based on the dijet ﬁnal state re-

main

applicable, they suffer from large dijet background produced

by quantum chromodynamics (QCD) subprocesses. We extend the

search for heavy neutral vector bosons by considering possible Z



decays into new particles predicted by various theoretical exten-

sions

of the SM.

In this Letter, we report on a search for a leptophobic Z



reso-

nance

that decays into four leptons via cascade decays as described



E-mail address: cms-publication-committee-chair@cern.ch.

Fig. 1. Leading order Feynman diagram for the production and cascade decay of a Z



resonance to a four-lepton ﬁnal state.

in Ref. [29]. In this model, the Z



is coupled to quark pairs but not

to lepton pairs, and can be produced with a large cross section at

the LHC. These non-standard Z



resonances also decay to pairs of

new scalar bosons (ϕ) each of which subsequently decays to pairs

of leptons (ϕ → 



, where  and 



= eor μ). Fig. 1 shows the

leading-order Feynman diagram for the production of four-lepton

ﬁnal states via a Z



resonance at a hadron collider. The reconstruc-

tion

of the ϕ bosons in the dilepton channel is ineﬃcient if the

difference between Z



and ϕ masses is large and the two daughter

leptons are consequently highly collimated. In the following sec-

tions

we describe a technique to increase the selection eﬃciency.

http://dx.doi.org/10.1016/j.physletb.2017.08.069

0370-2693/

SCOAP

564 The CMS Collaboration / Physics Letters B 773 (2017) 563–584

The analysis is a search for heavy narrow resonances decaying

into four isolated ﬁnal state leptons. The benchmark model [29]

assumes

(/M < 1%), corresponding to a natural width of the Z



resonance that is much smaller than the detector resolution. The

following ﬁnal states are considered: μμμμ, μμμe, μμee, μeee,

and eeee. The μμee, μμμe and μeee channels are included to al-

low

for the possibility of lepton ﬂavor violation (LFV) [30–32] in

the decays of the new scalar bosons. In this Letter, we set limits

on the product of the cross section and branching fraction for pro-

duction

and decay to four leptons, and interpret the results in the

context of the benchmark model described above [29].

2. The CMS detector

The central feature of the CMS apparatus is a superconduct-

ing

solenoid of 6 m internal diameter, providing a magnetic ﬁeld

of 3.8 T. Within the solenoid volume are a silicon pixel and strip

tracker, a lead tungstate crystal electromagnetic calorimeter (ECAL),

and a brass and scintillator hadron calorimeter (HCAL). Each de-

tector

is composed of a barrel and two endcap sections. Muons

are measured in gas-ionization detectors embedded in the steel

ﬂux-return yoke outside the solenoid. Extensive forward calorime-

try

complements the coverage provided by the barrel and endcap

detectors.

Muons

are measured in the range |η| < 2.4with detection

planes made using three technologies: drift tubes, cathode strip

chambers, and resistive-plate chambers. Matching muons to tracks

measured in the silicon tracker results in a relative p

resolution

for muons with 20 < p

< 100 GeV of 1.3–2.0% in the barrel and

better than 6% in the endcaps. The p

resolution in the barrel is

better than 10% for muons with p

up to 1TeV [33].

The

ECAL consists of 75 848 crystals that provide coverage in

pseudorapidity |η| < 1.48 in a barrel region (EB) and 1.48 < |η| <

3.00 in two endcap regions (EE). The electron momentum is es-

timated

by combining the energy measurement in the ECAL with

the momentum measurement in the tracker. The momentum reso-

lution

for electrons with transverse momentum p

≈ 45 GeV from

Z → e

−

decays ranges from 1.7% for nonshowering electrons

(approximately 30%) in the barrel region to 4.5% for showering

electrons (approximately 60%) in the endcaps [34].

more detailed description of the CMS detector, together with

a deﬁnition of the coordinate system used and the relevant kine-

matic

variables, can be found in Ref. [35].

3. The simulated event samples

The Monte Carlo (MC) generator program used to produce the

simulated event samples for the benchmark model is CalcHEP

3.4.1 [36] interfaced

with pythia 6.4.24 [37]. These samples are di-

vided

into ﬁve decay channels (μμμμ, μμμe, μμee, μeee, eeee)

for different Z



boson masses (m



) ranging from 250 to 3000 GeV

increments of 250 GeV. The benchmark model assumes that

new particles other than Z



and ϕ are heavy enough not to af-

fect

the production and decay of the Z



boson. Signal MC samples

are produced with six different values of the ϕ mass (m

), with

= 50 GeV used as the reference mass value in the interpre-

tation

of the results. An important feature of this analysis is the

presence of a “boosted signature” associated with the collimation

of the two leptons coming from the same parent particle and re-

sulting

from the large difference between m



and m

. In addition,

samples are generated with m

masses of 5, 10, 20, 30 and 40%

of m



, for which, in most cases, the contribution from the boosted

signature is less important. The product of the leading order (LO)

signal cross section and branching fraction in each channel varies

with m



(from 250 to 3000 GeV) as follows: μμμμ and eeee

from

0.8pb to 3.0 ×10

−6

pb, μμee from 12.3pb to 4.7 ×10

−5

pb,

and μμμe and μeee from 3.1pb to 1.2 × 10

−5

pb. The branching

fraction of ϕ → 



is set to 1 and therefore only the leptonic de-

cay

channels are considered. These signal MC samples are used to

optimize event selection, evaluate signal eﬃciencies and calculate

exclusion limits.

The dominant SM background is the production of ZZ decay-

ing

into four leptons. The qq-induced ZZ production is generated

using the pythia event generator and the gg-induced production

using the gg2zz program [38]. Additional backgrounds from di-

boson

production (WW and WZ) are generated with pythia, and

from top quark production (tt, tW, and tW) are generated with

powheg 1.0 [39].

Other processes, such as ttZ and triboson produc-

tion

(WWγ , WWZ, WZZ, and ZZZ), are generated with MadGraph

5.1.3.30 [40].

Simulated event samples are normalized using the

integrated luminosity and higher order theoretical cross sections:

next-to-next-to-leading order for tt [41] and next-to-leading order

for ZZ [42] and the other backgrounds.

The MC samples are generated using the CTEQ6L [43] set of par-

ton

distribution functions (PDFs) and the pythia Z2* tune [44,45]

order to model the proton structure and the underlying event.

The samples are then processed with the full CMS detector sim-

ulation

software, based on Geant4 [46,47], which includes trigger

simulation and event reconstruction.

4. Event selection

The 2012 data set of proton–proton collisions at

√

s = 8 TeV,

corresponding to an integrated luminosity of 19.7fb

−1

, is used for

the analysis. Data are collected with lepton triggers with various

thresholds. The trigger used for the muon-enriched channels

(μμμμ, μμμe) requires the presence of at least one muon can-

didate

with p

> 40 GeV and |η| < 2.1. The trigger used for the

electron-enriched channels (μeee, eeee) requires two clusters of

energy deposits in the ECAL with transverse energy E

> 33 GeV

each.

For the μμee channel, the trigger requires both an electron

and a muon with p

> 22 GeV.

In the subsequent analysis, events are required to contain a

reconstructed primary vertex (PV) with at least four associated

tracks, and its r (z) coordinates are required to be within 2 (24) cm

the nominal interaction point. The PV is deﬁned as the vertex

with the highest sum of p

for the associated tracks. We select the

events with four leptons in the ﬁnal state, where the leptons are

identiﬁed by the selection criteria described below. The two lead-

ing

leptons are required to have p

> 45 GeV to ensure that the

trigger is fully eﬃcient for the selected events. This requirement

has a negligible effect on the signal acceptance. The two sublead-

ing

leptons are required to have p

> 30 GeV. This choice balances

loss of eﬃciency against increased misidentiﬁcation probability. All

four leptons must satisfy |η| < 2.4. No charge requirement is ap-

plied

to the lepton selection.

Muon

candidates are reconstructed by a combined ﬁt including

hits in both tracking and muon detectors (“global muons”) [33].

The tracks associated with global muons are required to have the

following properties: at least one pixel detector hit, at least six

strip tracker layers with hits, at least one muon chamber hit, at

least two muon detector planes with muon segments, a transverse

impact parameter of the tracker track |d

| < 0.2cm with respect

to the PV, a longitudinal distance of the tracker track |d

| < 0.5cm

with

respect to the PV, and δp

< 0.3 where δp

is the uncer-

tainty

in the measured p

of the track. All muon candidates are

required to be isolated. A muon is considered isolated if the scalar

sum of all tracks within a cone of R < 0.3around the muon,

excluding the muon candidate itself, does not exceed 10% of the

The CMS Collaboration / Physics Letters B 773 (2017) 563–584 565

muon p

, where R =



(φ)

+(η)

. If there is a second lep-

ton

candidate within a cone R < 0.3, we remove its contribution.

An electron candidate is identiﬁed by matching a cluster in the

ECAL to a track in the silicon tracker [34]. Identiﬁcation criteria

are applied to suppress jets misidentiﬁed as electrons. Electrons

are required to pass the following criteria: the proﬁle of energy

deposition in the ECAL should be consistent with an electron, the

sum of HCAL energy deposits behind the ECAL cluster should be

less than 10% of the associated ECAL deposit, the track associated

with the cluster should have no more than one hit missing in the

pixel detector layers and |d

| should be less than 0.02 cm with

respect to the selected PV. All electron candidates are required to

be isolated using the following deﬁnition: within a cone R < 0.3

around

the track of the electron candidate, the p

sum of all other

tracks is required to be less than 5 GeV and the E

sum of the en-

ergies

of the calorimeter deposits that are not associated with the

candidate is required to be less than 5% of the candidate’s E

. This

differs from the isolation requirement of 3% in Ref. [13], because

of the ineﬃciency (of approximately 6% at electron E

= 1 TeV)

caused by overlapping electrons due to the high Lorentz boost of

the ϕ boson (m

= 50 GeV). In addition, if the direction of the sec-

ond

lepton candidate falls within the isolation cone of the ﬁrst

(R < 0.3), the contributions it makes to both p

and E

are sub-

tracted

when imposing the isolation requirements.

The

kinematic distributions of the ﬁnal-state particles are sim-

ilar

for all ﬁve channels. The ﬁnal state consists of two leading

leptons with high p

and two subleading leptons with relatively

low p

. The two leptons from the same parent ϕ boson can be

highly Lorentz boosted if m

is signiﬁcantly smaller than m



. This

feature is generally found for high-mass (m



> 1 TeV) samples in

the case of m

= 50 GeV. This boosted signature introduces a sig-

niﬁcant

ineﬃciency for the event selection except for the LFV case

(ϕ decaying into eμ). To take into account the boosted signature

for ϕ decaying into μμ, one of the muon candidates selected by

the above criteria is allowed to be reconstructed only as a tracker

muon, a track in the tracker matched to track segments in the

muon system (“tracker muons”) [33], if the two muons are as close

as R < 0.4. In such exceptional cases, the requirements of at least

one muon chamber hit and at least two muon detector planes with

muon segments are not applied to the tracker muon.

The

boosted signature for a ϕ decaying into ee is much more

complicated since the electrons can easily merge into a single

cluster in the ECAL. In this case, only one electron candidate is

reconstructed from the two original electrons. The probability for

having a merged candidate is about 50% with m



= 3 TeV and

= 50 GeV. These events would be rejected by the four-lepton

requirement, introducing a large signal ineﬃciency. To select such

events, an electron candidate having a ratio of ECAL cluster en-

ergy

to track momentum larger than 1.5 and a second track with

> 30 GeV within a cone of R(electron, track) < 0.25, is consid-

ered

as a “merged electron”. Events are accepted with three (two)

leptons if they contain one (two) merged electron(s), since each

merged electron is considered to contribute two electrons to the

total. In order to avoid signiﬁcant misidentiﬁcation, merged elec-

trons

are only considered if the ECAL cluster energy is bigger than

500 GeV.

The

dominant background in this analysis arises from ZZ events

decaying into four leptons. To suppress this background, events

with two oppositely charged same-ﬂavor lepton pairs are rejected

if the mass of the lepton pair, m



, is in the range 89–93 GeV. The

Zmass window is made as narrow as possible in order to min-

imise

degradation of the signal eﬃciency in the case of m

≈ m

This requirement results in negligible signal eﬃciency loss for



> 500 GeV. At lower masses, the eﬃciency loss increases and

is approximately 20 (7)% at m



= 250 GeV for the eeee (μμμμ)

channel. More than 70% (30%) of the ZZ background is rejected by

the mass window veto requirement in the muon (electron) chan-

nel.

This requirement is not applied to the merged electron case,

thus accounting for the difference in rejection eﬃciency for the

two channels.

The

event selection eﬃciency is 50–70% (μμμμ), 55–65%

(μμμe and μμee) and 45–65% (μeee and eeee) throughout the

range m



> 1 TeV for m

= 50 GeV. Below m



= 1 TeV, the eﬃ-

ciency

decreases rapidly because of the effect on the acceptance

of the kinematic requirements. Heavier m

values correspond to a

less boosted signature and therefore are selected with a higher ef-

ﬁciency.

For m



> 2TeV, the eﬃciency for the other m

samples is

approximately 10–15% (1–5%) higher in the electron (muon) chan-

nels

than for the m

= 50 GeV scenario, where the range of values

reﬂects the variation with m



. For m



< 1 TeV, the contribution

from boosted events is not signiﬁcant and the eﬃciency is similar

for all values of m

considered.

5. Background estimation

Most of the SM backgrounds are suppressed by requiring four

isolated high-quality lepton candidates. As discussed above, the

dominant background is from ZZ events decaying into four leptons.

Other backgrounds originate from top quark events with two gen-

uine

leptons and two lepton candidates arising from misidentiﬁed

jets, and from WW (WZ) events that contain two (one) misidenti-

ﬁed

or nonprompt leptons from jets. In the case of triboson pro-

duction,

there may be four genuine leptons in the event. These

backgrounds are estimated using MC simulation.

The

contribution from events with more than two leptons aris-

ing

from misidentiﬁed jets is expected to be small because this

analysis requires four isolated leptons in the ﬁnal state. This back-

ground

is estimated using the “misidentiﬁcation rate” method de-

scribed

in Ref. [13]. The misidentiﬁcation rate measured as a func-

tion

of electron E

in the barrel and endcap is applied to events

with electron candidates passing the trigger but failing the full se-

lection.

The contribution from jet backgrounds estimated using this

procedure is found to be negligible.

Fig. 2 sho

ws the four-lepton invariant mass (m

4

) distribution

for selected events. The number of observed events and estimated

backgrounds are summarized in Table 1. As shown in the ﬁgure

and table, the distribution of observed events is in agreement with

the expected backgrounds. The table shows two different mass

ranges. In the region m

4

> 1 TeV, the backgrounds from SM pro-

cesses

are very small, typically less than one event.

6. Results

No excess of events is observed in the data sample compared

to the SM expectations and exclusion limits at 95% conﬁdence

level (CL) are calculated in the context of the benchmark model.

The signal region consists of events with four leptons (e or μ)

with |η| < 2.4: the two leading (subleading) leptons are required

to have p

> 45 (30) GeV. A Bayesian approach is adopted with a

likelihood function deﬁned with a signal strength modiﬁer, a prior

probability, and a set of nuisance parameters. The prior probabil-

ity

distribution for the signal cross section is positive and uniform,

since this is known to result in good frequentist coverage proper-

ties.

The systematic uncertainties associated with the backgrounds,

selection eﬃciency and luminosity are treated as nuisance param-

eters

with log-normal prior distributions [48]. A limit on the signal

contribution is derived by interpreting the likelihood function as a

probability distribution and integrating over this. The coverage of

the 95% CL assigned to the limit has been checked using a Markov

chain Monte Carlo method.

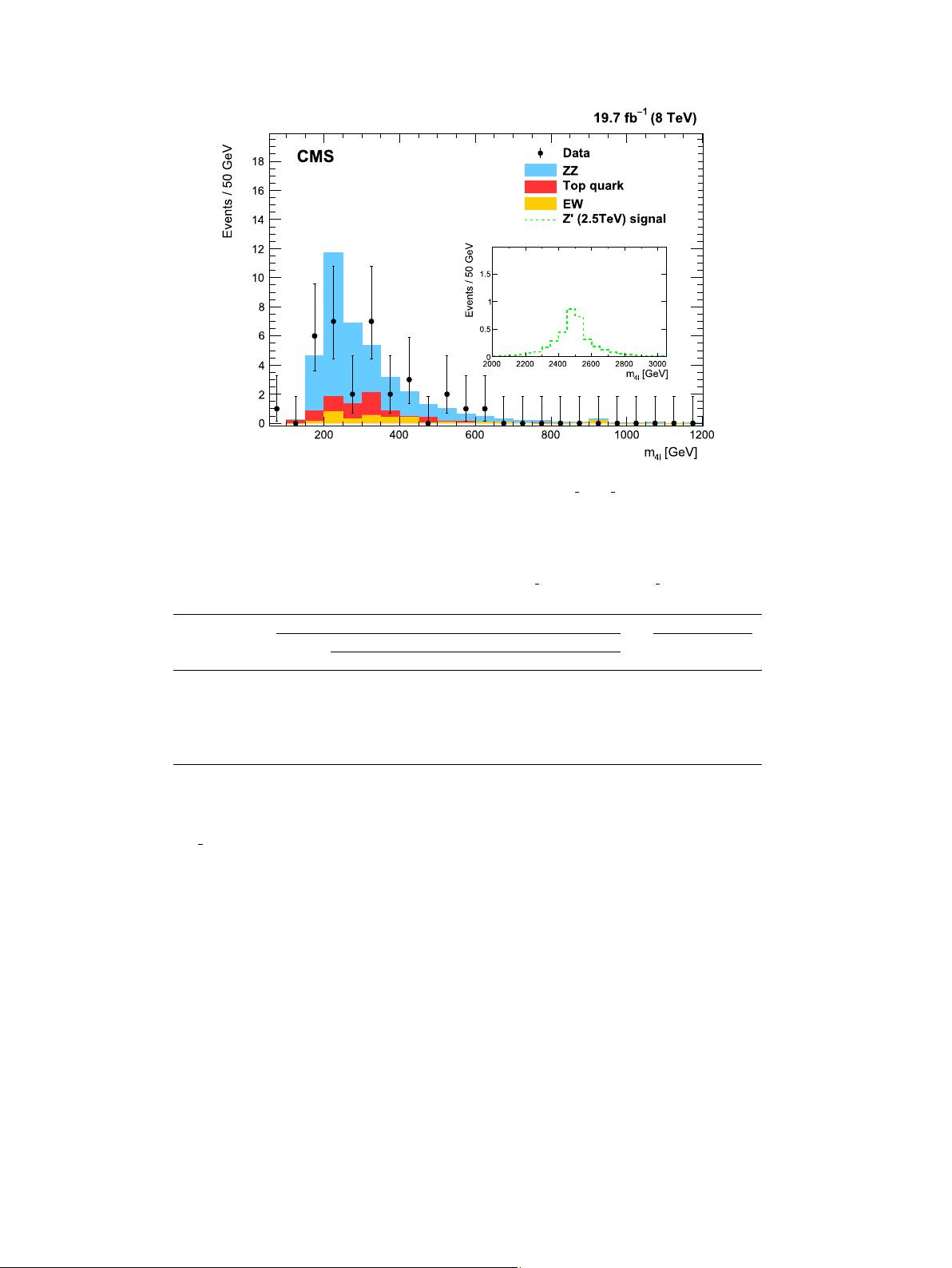
566 The CMS Collaboration / Physics Letters B 773 (2017) 563–584

Fig. 2. The m

4

spectrum for the combination of the ﬁve studied channels. The points with vertical bars represent the data and the associated statistical uncertainties; the

histograms represent the expectations from SM processes; “Top quark” denotes the sum of the events for tt, tW, ttZ processes; “EW” denotes the sum of the events from

WW, WZ, WWγ , WWZ, WZZ, and ZZZ processes. The inset shows the expectation from the benchmark model for a signal at m



= 2.5 TeV with m

= 50 GeV.

Table 1

Summary

of the observed yield and expected backgrounds for all channels, where N

obs

is the number of observed events

in data. The total background (N

tot

) is the sum of three different backgrounds that are estimated using MC simulations;

refers to the background from ZZ events; N

is the background from tt, single top quark, and ttZ production; N

the background from WW and WZ, and triple gauge boson production. The quoted uncertainties are statistical only.

Channel 0.1 < m

4

< 1.0TeV m

4

> 1.0TeV

obs

SM backgrounds N

obs

tot



→ μμμμ 34.9± 0.3 0.9 ± 0.5 – 5.9 ± 0.6 0 –



→ μμμe6 0.4± 0.1 1.3 ± 0.6 1.2 ± 0.3 2.9 ± 0.7 0 –



→ μμee 12 9.3 ± 0.4 3.0 ± 1.5 1.2 ± 0.3 13.5 ± 1.6 0 0.1 ± 0.1



→ μeee 2 0.2 ± 0.1 0.4 ± 0.1 0.6 ± 0.2 1.2 ± 0.2 0 0.1 ± 0.1



→ eeee 9 15.0 ± 0.5 0.2 ± 0.1 0.2 ± 0.1 15.4 ± 0.5 0 0.2 ± 0.1

Combined 32 29.9

± 0.7 5.7 ± 1.9 3.3 ± 0.5 38.9 ± 2.1 0 0.4 ± 0.2

The systematic uncertainties are dominated by the uncertainties

in the background estimates and in the lepton selection eﬃcien-

cies.

The uncertainty in the MC estimation of the main background

cross section (ZZ and tt) arising from higher-order QCD correc-

tions

and choice of PDFs is 15%. In order to be conservative, we

choose to double this ﬁgure and assign an uncertainty of 30%

from this source. The systematic uncertainty in the muon selection

eﬃciency including reconstruction, identiﬁcation, and isolation is

0.5% [33]. The uncertainties in the electron selection eﬃciency

are 0.7% (0.6%) for electrons below 100 GeV in EB (EE) and 1.4%

(0.4%) for electrons above 100 GeV in EB (EE) [13]. The uncertain-

ties

due to the lepton eﬃciency in both signal and background

yields vary between 2.2% and 2.7% as a function of m

4

. Includ-

ing

the effect of the merged lepton signature, a total uncertainty

of 10% in the event selection eﬃciency is assigned for each chan-

nel.

The impact of the uncertainty in the electron energy scale on

signal (background) yield is 1% (0.5%) [13]. Uncertainties in the

muon momentum scale and mass resolutions are below 0.1% [33].

The uncertainty in the integrated luminosity is assigned to be 2.6%

[49]. In this analysis, the statistical uncertainties are dominant and

the systematic uncertainties have a small impact on the results.

We tested the robustness of the limits by doubling the values as-

sumed

for the systematic uncertainties. We observed a negligible

change in the calculated limits, and conclude that the limits are

insensitive to any underestimation of the systematic uncertainties.

Limits on the product of cross section and branching fraction

are set in the context of the benchmark model as a function of

4

. The natural width of the Z



resonance is assumed to be

smaller than the mass resolution of the detector in all channels.

The detector resolution in the μμμμ channel varies from 1.1%

at m

4

= 250 GeV to 7.5% at m

4

= 3 TeV, and it has a constant

value of about 0.6% over this range in the eeee channel. In the

limit calculation, we set the mass window to be six times the

mass resolution centred around the signal mass point considered.

A counting experiment is performed for the limit calculation. Fig. 3

shows

the upper limits on the product of the cross section and

branching fraction, for the combination of all ﬁve channels, for the

mass range considered in the benchmark model of Ref. [29]. In the

framework of this model, we translate these cross section upper

limits into lower limits on the Z



boson mass. For the combina-

tion

of the ﬁve channels, the value obtained for this lower mass

limit is 2.5TeV. The black solid (dashed) line indicates the observed

(expected) 95% CL upper limits, the inner (outer) band indicates

the ±1 (2) standard deviation uncertainty in the expected limits,

and the blue dashed line shows the theoretical Z



cross section

for m

= 50 GeV. This theoretical cross section is calculated un-

der

the benchmark model assumption that the branching fraction

剩余21页未读，继续阅读

评论收藏

内容反馈

weixin_38674675

粉丝: 3
资源: 920

搜索在s = 8TeV的质子-质子碰撞中衰变成四轻子最终态的疏疏Z'玻色子

最新资源

搜索在s = 8TeV的质子-质子碰撞中衰变成四轻子最终态的疏疏Z'玻色子

在质子-质子碰撞中，在四个b夸克最终状态下寻找衰变成一对希格斯玻色子的共振。

搜索质子-质子碰撞中像矢量一样的T夸克衰变成Z玻色子和顶夸克的单项生成

搜索在s = 8 $$ \ sqrt {s} = 8 $$ TeV的质子-质子碰撞中衰变成魅力和底部夸克的带电希格斯玻色子

在s = 8TeV的质子-质子碰撞中搜索通过WZ衰减到轻子的新共振

用ATLAS探测器在s = 13 TeV的质子-质子碰撞中希格斯玻色子衰减成一对τ轻子的截面测量

矢量玻色子散射和约束对s = 13 TeV的质子-质子碰撞中具有四个轻子和两个射流的事件具有异常四次耦合的约束

在s = 8 TeV的质子-质子碰撞中搜索X（5568）衰变为Bs0π±的状态

搜索质子-质子碰撞中质子-质子碰撞的矢量样夸克衰变到顶夸克和$$ \ mathrm {W} $$ W玻色子的单次生成= 13 \，\ text {TeV} $ $ s = 13TeV

在s = 13 $$ \ sqrt {s} = 13 $$ TeV的质子-质子碰撞中的H±→τ±ντ衰变通道中搜索带电的希格斯玻色子

在s = 8TeV的质子-质子碰撞中搜索带有右手耦合的重中微子和W玻色子

使用ATLAS探测器在s = 13 TeV的质子-质子碰撞中使用两个或三个带电轻子在最终状态下使用递归曲线重构在最终状态下搜索中性中子的产生

在s = 13 TeV的质子-质子碰撞中与Z玻色子相关的单顶夸克产生的观察

在s $$ \ sqrt {s} $$ = 13 TeV的质子-质子碰撞中，在轻子和质子-质子碰撞的WW和WZ生产中搜索异常的三重量规耦合

在s = 13 TeV的质子-质子碰撞中，在带有三个荷电轻子的事件中搜索重中性轻子

在s = 13TeV的pp碰撞中测量单个顶夸克和Z玻色子的相关产量

使用ATLAS探测器在s = 13 TeV的质子-质子碰撞中测量ttZ和ttW截面

在s = 13 $$ \ sqrt {s} = 13 $$ TeV的质子-质子碰撞中搜索衰落到tau轻子对的重共振

用ATLAS探测器在s = 13 TeV的pp碰撞中搜索与Z玻色子相关的无形衰变的希格斯玻色子或暗物质候选物

在s = 8 TeV的pp碰撞中，结合W或Z玻色子测量顶夸克-反夸克对的产生

使用ATLAS探测器在s = 13 TeV时使用36 fb-1质子-质子碰撞数据搜索重共振衰减为玻色子和轻子最终状态的搜索组合

在s = 8 $$ \ sqrt {s} = 8 $$ TeV的质子-质子碰撞中搜索衰减到tW的激发底夸克的产生

在$ \ sqrt {s} = 13 \，\ text {TeV} $$ s = 13TeV的质子-质子碰撞中，在Dilepton最终态的顶夸克生成中搜索新的物理学

最新资源