Engineeringtheemissionoflaserarraystonullifythejammingfrompassiveobstacles资源-CSDN文库

80 浏览量 2021-01-27 05:11:35 上传评论收藏 1MB PDF 举报

资源推荐

资源详情

资源评论

Engineering the emission of laser arrays

to nullify the jamming from passive obstacles

CONSTANTINOS VALAGIANNOPOULOS* AND VASSILIOS KOVANIS

Department of Physics, School of Science and Technology, Nazarbayev University, 53 Qabanbay Batyr Ave, Astana KZ-010000, Kazakhstan

*Corresponding author: konstantinos.valagiannopoulos@nu.edu.kz

Received 30 October 2017; revised 26 April 2018; accepted 29 May 2018; posted 1 June 2018 (Doc. ID 312268); published 10 July 2018

Non-Hermitian characteristics accompany any photonic device incorporating spatial domains of gain and loss.

In this work, a one-dimensional beam-forming array playing the role of the active part is disturbed from the

scattering losses produced by an obstacle in its vicinity. It is found that the placement of the radiating elements

leading to perfect beam shaping is practically not affected by the presence of that jammer. A trial-and-error inverse

technique of identifying the features of the obstacle is presented based on the difference between the beam target

pattern and the actual one. Such a difference is an analytic function of the position, size, and texture of the object,

empowering the designer to find the feeding fields for the lasers giving a perfect beam forming. In this way, an

optimal beam-shaping equilibrium is re-established by effectively cloaking the object and nullifying its jamming

effect.

OCIS codes: (140.3300) Laser beam shaping; (160.3918) Metamaterials; (230.3205) Invisibility cloaks.

https://doi.org/10.1364/PRJ.6.000A43

1. INTRODUCTION

Collective operation of laser waveguides in arrays and networks

is the backbone of several state-of-the-art applications and

recent advances in photonics and lightwave technologies.

One-dimensional laser phased arrays characterized by strong

nonlinearity and non-Hermiticity have been experimentally in-

vestigated in Ref. [1], where the effect of various symmetries on

multimode emission and edge-mode lasing has been identified

(free-space wavelength λ

≅ 1.59 μm). In two dimensions, net-

works of optical nanoantennas have been found able to support

functionalities beyond conventional focusing and steering use-

ful in three-dimensional holography and biomedical testing [2]

(λ

≅ 1.55 μm). Of course, the major application of such

structures remains efficient beam shaping, which can be elec-

tronically controlled based on hybrid prototypes of dielectric

waveguides and metallic nanoemitters [3](λ

≅ 1.57 μm)

and provides grating lobe-free steering for light detection

and ranging [4](λ

≅ 1.55 μm).

Regarding beam forming in similar THz applications, an

inverse problem for the excitations of an array of emitters

has been lately formulated [5]. Inspired by long established

level-set methods for computing moving fronts [6], new limits

for the radiation of emitters [7] and the recent inverse-design

paradigm shift in photonic design [8], the optimal arrangement

of the cavity lasers is considered. It has been reported [5] that

the distance between two consecutive radiating elements

should fall within an approximate value range, so that the

aggregate far-field response mimics perfectly a specific target

pattern. In particular, it is found that the waveguides should

not be placed too close to each other, or they will act as one

source unable to create a directive collective pattern.

Additionally, they cannot be very distant from each other

because each emitter should talk with the neighboring ones to

give a combined response instead of a sum of isolated and

uncorrelated radiation patterns.

Forward and inverse problems such as the aforementioned

ones have appeared for various bands of operational frequen-

cies. In radio engineering, e.g., clusters of radiators have been

traditionally used for optimal beam forming and, most impor-

tantly, adaptive techniques are employed to avoid the jamming

of the collective radiation pattern due to several causes.

Indicatively, signal processing methods that allow the system

to fully adapt to a complex spatio-temporal environment con-

taining jammers are presented in Ref. [9]. Furthermore, filter-

ing techniques that suppress the perturbation of the

information signal from interference sources by selecting the

suitable transmitting array [10] or alleviate the harming effects

of array imperfections [11] are also known and available.

Alternatives to these historical signal cancellation [12]

approaches are the modern cloaking techniques that allow an

object to interact minimally with the background field.

Similarly, the jamming effect of an obstacle can be mitigated

with use of passive dielectric coats [13,14] or periodic metallic

flanges that guide the incident field around it [15]. More easily,

an object that jams the signal from the source can vanish by

neutralizing its scattering field with active components

Research Article

Vol. 6, No. 8 / August 2018 / Photonics Research A43

such as electric/magnetic currents [16] or non-foster meta-

surfaces [17].

In this paper, we pair the structure of a beam-shaping laser

array with a near-field obstacle that jams the formed radiation

pattern. The cluster of emitters is identical to that of Ref. [5],

where their optimal excitations for the best beam forming are

computed. In the presence of the obstacle, the new effective

current feeds leading to a perfect result are determined by

solving the corresponding boundary value problem. The per-

missible range for the distance between the laser waveguides

remains the same as in the obstacle-free analysis [5], since

the object is passive and acts as a secondar y source. The con-

sidered system combines gain (lasers) with loss (obstacle) and

clearly constitutes a non-Hermitian photonic configuration

[18–23]. Note that losses are not referring only to that part

of energy that is converted into thermal form due to the

passivity of the obstacle; we can define effective scattering losses

describing the jamming created by the object that destroys an

already established equilibrium. More specifically, in a working

beam-forming device, an object appears and harms the proper

response, behaving as an effective lossy part; to remedy that

situation, we re-adjust the active part and cloak the obstacle

by producing an aggregate response identical to the desired

target pattern.

This paper is organized as follows. In Section 2, we present

the configuration and state the assumption for the two-

dimensional variation. To this end, we rigorously impose

the boundar y conditions to obtain a linear system whose sol-

ution is the local output fields of the lasers giving an optimal

beam forming in the presence of the obstacle. In Section 3,we

define the value ranges for the input parameters and our basic

observable metric, which is the error of the obstacle-free solu-

tion. Furthermore, we present the possibility of finding some

(or even all) objects’ features from the variations of that metric

and demonstrate the effectiveness of our method if one has

exact knowledge of the size, the texture, and the position of

the obstacle. Finally, in Section 4, we summarize the proposed

methodology and briefly mention our future plans on

non-Hermitian engineering for structures of the same class.

2. PROBLEM STATEMENT

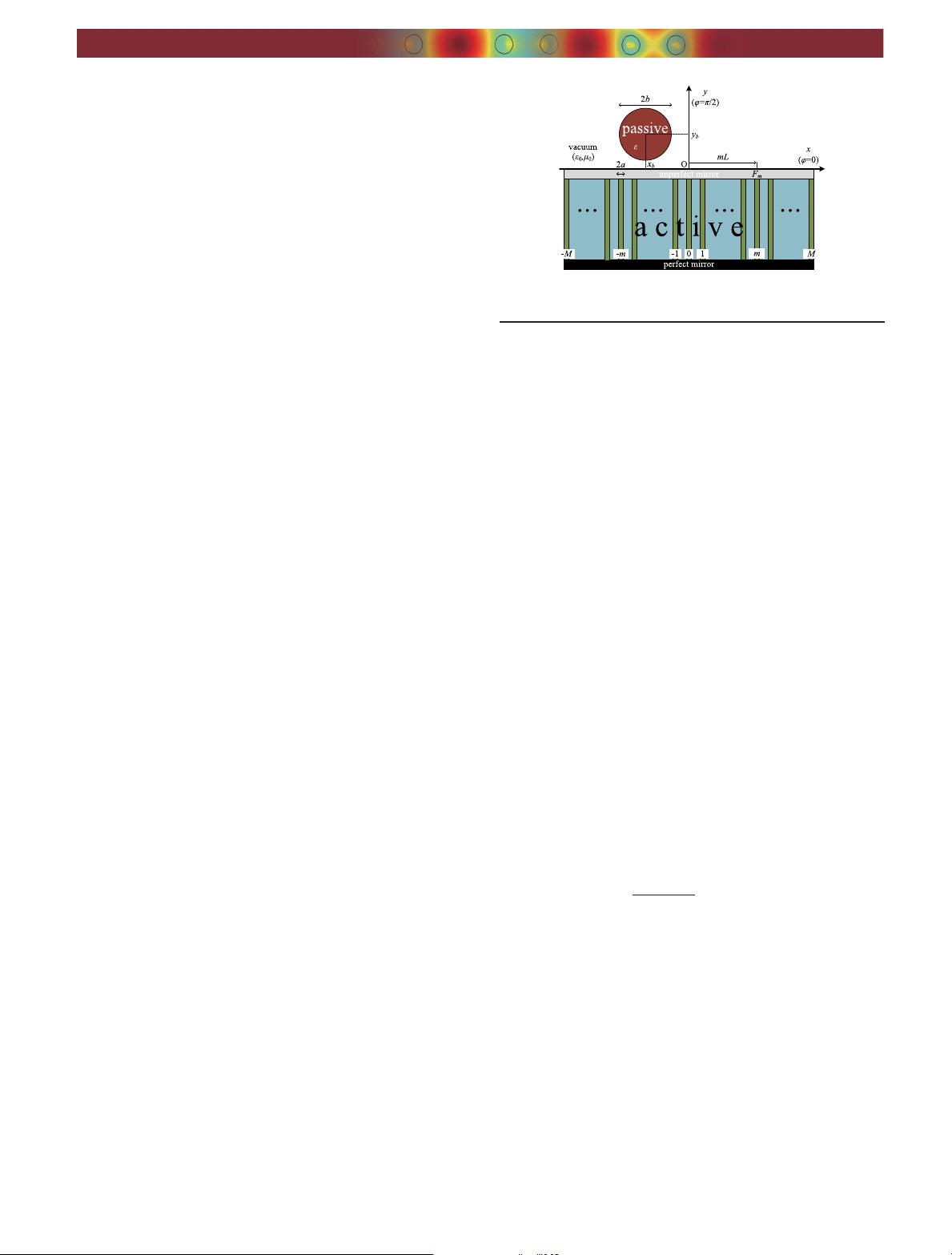
Let us consider an array of multiple laser emitters radiating into

free space ε

, μ

 as that depicted in Fig. 1, where the used

Cartesian x, y, z and cylindrical r, φ, z coordinate systems

are also defined. Referring to the z  0 plane, we regard

2M  1 laser waveguides of common finite length along

y axis defined by a perfect and an imperfect mirror (at

y  0) and equispaced along x axis. These cavities are properly

fed to develop a z-polarized electric field at their ends, with

complex phasors denoted by F

for m  −M, …, M, which

gets diffused into vacuum half space y>0 [24]. A cylindrical

obstacle of radius b and filled with material of relative complex

permittivity ε, is positioned along axis x, yx

, y

 and

jams the collectively produced field of the waveguides [15].

We assume that the output fields F

do not significantly alter

in the presence of the obstacle, despite the formed external

cavity, which may influence the intrinsic behavior of the lasers.

Indeed, the size of the cylinder 2b is usually chosen much

smaller than the length 2ML of the radiating aperture and thus

may affect only a minute number of elements. Furthermore,

according to feedback literature [25,26], there are ways to mit-

igate the effect of outer mirrors on the characteristics (intensity

threshold) of the formed external cavity laser. The distance

between two consecutive cavities equals L, and the transversal

size of each of them equals 2a. The suppressed time depend-

ence is of harmonic form: e

jωt

A major assumption of this study is that the phasor of

the electric field remains constant across the entire zone

fjx − mLj <a, y  0g equal to F

; it simplifies substantially

the considered problem by making it two dimensional (field

distributions independent from z). In other words, the struc-

ture and excitation are taken unaltered along z axis and, thus,

the system’s response is the same regardless of the observation

plane, as long as it is parallel to the xy one. Such a reduction is

not unrealistic, since one may consider identical (with respect

to structure, texture, and feed) sets of waveguides as those

existing on z  0 plane to be positioned along parallel planes

covering a distance along z axis equal to W . In this way, an

illusion of z independence is created, which gets more success-

ful for increasing W . In particular, our analysis would be

exactly valid for the entire space if W → ∞ and would describe

qualitatively the spatial distributions only for xy plane

for W → 0.

The background (z-directed) electric field, in the absence of

the obstacle, is written as an aggregation of the outputs of the

2M  1 emitters [5]

back

x, y

m−M

2

k

a

2



ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

x − mL

 y



(1)

where k

 ω

ﬃﬃﬃﬃﬃﬃﬃﬃﬃ

 2π∕λ

is the free-space wavenumber,

and H

2

is the Hankel function of u-th order and second type.

The quantity λ

is the free-space wavelength. The quantity

2

k

a is used for normalization purposes, since the outputs

of the lasers are considered as constant throughout the cross

section of the ends of the waveguides. Therefore, Eq. (1) is suit-

able only for points external to the laser cavities, since there is

no actual field singularity in the interior of them. In other

words, we assume that the waves are the outcome of point

sources only outside of the lasers that produce them. This field

is scattered by the obstacle, and the signal that perturbs the

background distribution can be expressed as

Fig. 1. Schematic of the regarded configuration. The aggregate field

of an active laser array is perturbed by a passive cylindrical obstacle.

A44 Vol. 6, No. 8 / August 2018 / Photonics Research

Research Article

剩余7页未读，继续阅读

评论收藏

内容反馈

weixin_38670433

粉丝: 9
资源: 899

Engineering the emission of laser arrays to nullify the jamming ...

最新资源

Engineering the emission of laser arrays to nullify the jamming ...

The substrate temperature dependence of the field emission properties of Si tip arrays with N-doped SrTiO3 thin films

Scattering, Absorption, and Emission of Light by Small Particles

Laser Physics:From Principles to Practical Work in the Lab-Springer (2014).pdf

Frequency spectrum characterization of terahertz emission from laser-induced air plasma

The Spectral Emission Characteristics of Laser Induced Plasma on Tea Samples

Effect of nitrogen addition on the band gap, core level shift, surface energy, and the threshold field of electron emission

Amplified spontaneous emission contrast of CPA laser

What can we exactly learn from the XPS measurement of the orientation-resolved surface core level shift?

Characteristics of optical emission during laser-induced damage events of fused silica

A novel approach to improve the field emission characteristics of printed CNT films

Highly efficient continuous-wave laser operation of laser diode-pumped Nd,Y:CaF2 crystals

计算机网络第六版答案

Transient process in the triplet states of phosphorescent Ir(ppy)3 material doped in organic materials: peak shift of the emission band in polycarbonate

Supersymmetric microring laser arrays

Automotive Emission Control

Improvement Field Emission of Carbon Nanotube/Polyurethane Foam Composites by Cellular Wall Field Enhancement Structure

【船级社】 RS Guidelines on the Application of Provisions on the Tech

Anisotropy of laser emission in monoclinic Nd:ScYSiO5 crystals cut along the optical indicatrix axes

【正版】ISO 9613-2-1996.pdf

2018年美赛E题M奖论文（一等奖）

Broadband fluorescence emission in Bi-doped silica glass prepared by laser additive manufacturing technology

Effect of charge compensation on emission spectrum of Ca2SiO4:Dy3+ phosphor

Handbook of Research on Soft Computing and Nature-Inspired Algorithms

Optimal distance from high power laser diode to cylindrical microlens in a coupling system

最新资源