没有合适的资源?快使用搜索试试~ 我知道了~
我们考虑对彩色玻璃冷凝物中颗粒产生的非本征校正,这是由于获得有限纵向尺寸的目标的冲击波近似值松弛所致。 我们导出了Lipatov顶点的修改表达式,该表达式考虑了此有限的目标宽度。 该表达式用于计算格拉斯玛图极限中单,双和三重胶子的生成,该数量对两个稀薄对象的散射有效,并且扩展了颜色数量的所有阶数。 我们证明并归纳了先前的结果,并讨论了这些非本征校正的两个粒子相关性的可能含义,这些校正会引起远侧和近侧峰之间的差异。
资源推荐
资源详情
资源评论

Eur. Phys. J. C (2019) 79:600
https://doi.org/10.1140/epjc/s10052-019-7097-5
Regular Article - Theoretical Physics
Non-eikonal corrections to multi-particle production in the color
glass condensate
Pedro Agostini
1
, Tolga Altinoluk
2
, Néstor Armesto
1,a
1
Instituto Galego de Física de Altas Enerxías IGFAE, Universidade de Santiago de Compostela, 15782 Santiago de Compostela, Galicia, Spain
2
National Centre for Nuclear Research, 00-681 Warsaw, Poland
Received: 19 February 2019 / Accepted: 1 July 2019 / Published online: 16 July 2019
© The Author(s) 2019
Abstract We consider the non-eikonal corrections to parti-
cle production in the color glass condensate stemming from
the relaxation of the shockwave approximation for the tar-
get that acquires a finite longitudinal dimension. We derive
a modified expression of the Lipatov vertex which takes into
account this finite target width. This expression is employed
to compute single, double and triple gluon production in
the Glasma graph limit valid for the scattering of two dilute
objects, at all orders in the expansion in the number of col-
ors. We justify and generalize previous results, and discuss
the possible implications on two particle correlations of these
non-eikonal corrections that induce differences between the
away- and near-side peaks.
1 Introduction
Particle production at high energies in the soft and semi-
hard regimes is usually computed resourcing to high energy
approximations [1], namely the eikonal approximation. This
is the case in the color glass condensate (CGC) [2–4]. In this
framework, the process of propagation of an energetic parton
from the projectile through the target, considered as a back-
ground field, is computed in the light cone gauge neglecting
its transverse components and considering it as infinitely time
dilated and Lorentz contracted (thus treated as a shockwave),
see for example the discussion in [5]. Also terms subleading
in energy (among them, spin flip ones) are neglected. On the
other hand, in the calculation of elastic and radiative energy
loss of energetic partons traversing a medium composed of
coloured scattering centers – jet quenching – the shockwave
approximation is relaxed and the target is considered to have
a
e-mail: nestor.armesto@usc.es
a finite length, see e.g. the reviews [6,7].
1
In this context, a
systematic expansion of the gluon propagator in non-eikonal
terms was done in [5,11] and applied to particle production
in the CGC in [12]. Non-eikonal corrections at high energies
have also been treated recently in the context of Transverse
Momentum Distributions and spin physics [13–19], and soft
gluon exponentiation [20–22].
In the CGC, particle production and correlations have been
computed within several approximation schemes, providing
an alternative explanation to final state interactions for the
ridge phenomenon observed in small systems, proton–proton
and proton–nucleus, at the Large Hadron Collider (LHC)
at CERN [23–36] and the Relativistic Heavy Ion Collider
(RHIC) at BNL [37–41]. The “Glasma graph” approximation
[42,43], suitable for collisions between two dilute objects
like proton–proton and containing both Bose enhancement
and Hanbury–Brown–Twiss effects [44–47], has been used
to describe experimental data [48–51], and to compute three
and four gluon correlations [52,53]. Quark correlations have
also been calculated in this framework [54,55]. It was later
extended to dilute–dense (proton–nucleus) collisions both
numerically [56] and analytically [57–59], and used to cal-
culate three gluon correlations [58]. A description of data has
been obtained [60,61]. Density gradients [62] have also been
considered to explain the observed azimuthal structure.
Beyond the analytical extension to dense–dense colli-
sions, the remaining key theoretical problem for the descrip-
tion of azimuthal structure in small systems in the CGC lies
in odd harmonics that are absent in usual calculations. For
this, density corrections in the projectile [63–65], quark cor-
relations [60,66,67] and a more involved description of the
target [68,69] than the one provided by the commonly used
McLerran–Venugopalan (MV) model [
70,71], have been
1
The relation between jet quenching and CGC calculations, using the
formalism in [8,9], was established in [10] where the validity of the
eikonal approximation for this type of computations was also addressed.
123

600 Page 2 of 27 Eur. Phys. J. C (2019) 79 :600
Fig. 1 Diagrams that contribute to the computation of the Lipatov vertex. The black dot represents the Lipatov vertex which is the sum of all real
diagrams for gluon production shown on the right hand side of the equation
proposed. Using the former, a description of data is possi-
ble [72–74].
In this manuscript we deal with non-eikonal corrections
to particle production in the CGC that stem from relaxing
the shockwave approximation for the target, which becomes
of finite length. These are the corrections included in jet
quenching calculations and systematically expanded up to
next-to-next-to-leading order in [5,11]. In Sect. 2 we derive
an expression for the Lipatov vertex – one central building
block for particle production calculations in the CGC – that
takes into account the finite longitudinal extent of the target
field. While by itself this result is not new and similar cal-
culations and expressions can be found in the literature, see
e.g. Refs. [75,76] or more recently in Ref. [77], its identi-
fication for use to include non-eikonal corrections in CGC
calculations is done here for the first time. Then, in Sect. 3 we
apply our corrections to gluon production in the dilute-dilute
(Glasma graph) limit, following the notations in [58]. First, in
Sect. 3.1 we consider single gluon production, matching the
results in [12] and justifying the educated guess done there
on the basis of the expansion up to next-to-next-to-leading
order. Then we consider double gluon production in Sect. 3.2,
where we generalize the results in [12]. Third, in Sect. 3.3
we compute three gluon production. Finally, in Sect. 4 we
discuss our results. We focus on providing analytical expres-
sions and show a few numerical results; a more complete
study of the impact of non-eikonal corrections on particle
correlations is left for a forthcoming study [78].
2 Derivation of the non-eikonal Lipatov vertex
As usually done in the CGC, we describe a high energy p-A
collision by a right moving dilute projectile which interacts
with a left moving dense target described by a random and
intense (O(1/g)) classical gluon field A
μ
(x). The simplest
setup to derive the non-eikonal Lipatov vertex is considering
the emission of a gluon from a projectile massless quark in
the process of a single scattering with the target (an analo-
gous calculation leading to the same conclusions on the non-
eikonal corrections holds for a projectile gluon). In light cone
coordinates a
±
= (a
0
±a
3
)/
√
2 and in the light cone gauge
(n · A = A
+
= 0, n = (0, 1, 0
⊥
) in (+, −, ⊥) coordinates),
this field can be written as
A
μ
(x) ≈ δ
μ−
δ(x
+
)A
−
(x
⊥
), (1)
since the transverse component of the gluon field is not
altered by the large Lorentz γ factor, the x
−
dependence dis-
appears due to the time dilatation and the target is shrinked
to x
+
= 0 forming a shock-wave. However, in some applica-
tions these suppressed terms may be sizeable. For this reason,
in this note we will relax the infinite boost approximation, in
order to calculate the corresponding non-eikonal corrections
to the usual Lipatov vertex computed at O(g
2
).
To proceed, we analyze gluon production in p-A collisions
in the quark initiated channel and compute the Lipatov vertex,
which is an effective vertex that takes into account all the real
contributions to gluon production. For that one needs to sum
the amplitudes where the gluon is emitted before, during and
after the interaction with the field as shown in Fig. 1.
Our setup is such that the right moving quark with momen-
tum p +k −q is generated by some function J (p +k −q) =
J (p
+
+k
+
−q
+
) at x
+
0
=−∞and (x
−
0
, x
0⊥
) = 0, and then
interacts with the classical gluon field A
μ
(x) generated by
one scattering source located at x
1
, picking up a momentum
q. However, since we are interested in non-eikonal correc-
tions, we consider A
μ
(x) with an x
+
dependence which has a
finite support instead of treating it as a shockwave at x
+
= 0,
but we still assume that there is no dependence on x
−
. That
is, the new form of Eq. (1)is
A
μ
(x) ≈ δ
μ−
A
μ
(x
+
, x
⊥
), (2)
or, in momentum space,
A
μ
(q) ≈ δ
μ−
2πδ(q
+
)A
−
(q
−
, q
⊥
). (3)
Furthermore, we assume that the outgoing quark has a large
momentum p
+
compared to all other momenta in the process.
The general strategy in this case is to keep the leading terms
in +-momenta in the numerator algebra, while taking the
full phase corrections coming from the integration of the
denominators, see below, as done in the Furry approximation
and its non-abelian generalization [79].
We start by computing diagram A where the gluon is emit-
ted with momentum k before the quark interaction with the
target field as shown in Fig. 2. Using the Feynman rules,
we find that the amplitude for fixed gluon and final quark
momenta is
123
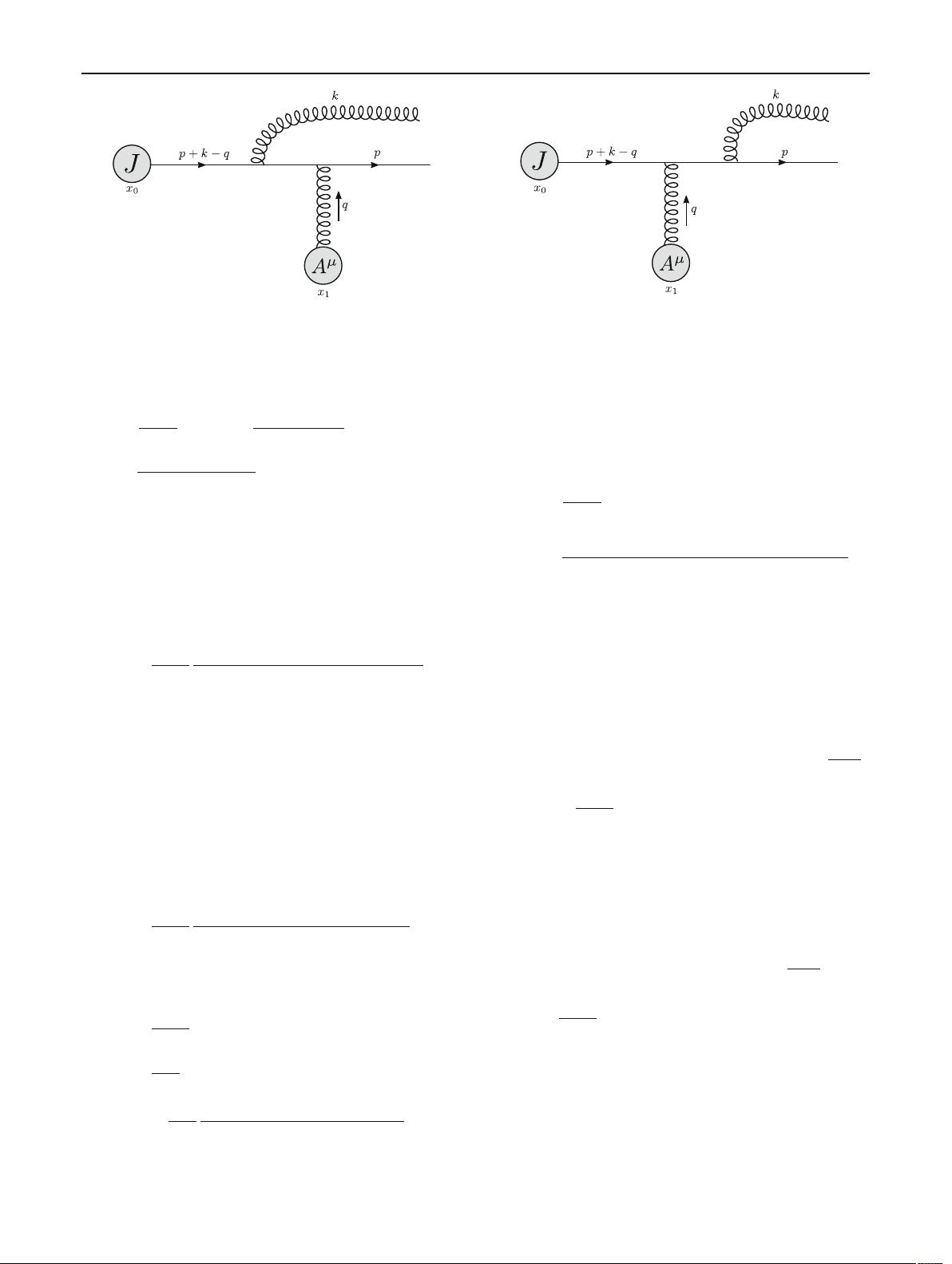
Eur. Phys. J. C (2019) 79 :600 Page 3 of 27 600
Fig. 2 Diagram A where the gluon is emitted before the interaction of
the quark with the target field
iM
A
=¯u( p)(−igγ
μ
t
a
)
d
4
q
(2π)
4
A
a
μ
(q)e
iqx
1
i(
/
p −
/
q)
( p − q)
2
+ i
(−igγ
ν
t
b
)
b∗
ν
(k)
×
i(
/
p +
/
k −
/
q)
( p + k − q)
2
+ i
e
i( p+k−q)x
0
J (p + k − q), (4)
with t
a
the SU(N
c
) generators in the fundamental represen-
tation.
Since p
+
is the largest momentum in our problem, we
approximate
/
p −
/
q ≈
/
p and
/
p +
/
k −
/
q ≈
/
p and write
iM
A
≈¯u( p)e
i( p+k)x
0
g
2
t
a
t
b
d
4
q
(2π)
4
/
A
a
(q)
/
p
/
b∗
(k)
/
p
[( p − q)
2
+ i][( p + k − q)
2
+ i]
e
iq(x
1
−x
0
)
J (p
+
+ k
+
− q
+
). (5)
Using again the eikonal approximation ( p
+
much larger
than all other momenta), we can approximate ( p − q)
2
≈
−2 p
+
q
−
and ( p + k − q)
2
≈ 2 p
+
(k
−
− q
−
). Employing
/
a
/
b = 2a ·b−
/
b
/
a and the massless Dirac equation ¯u( p)
/
p = 0,
we get ¯u( p)
/
A
a
(q)
/
p
/
b∗
(k)
/
p =¯u( p)4( p ·A
a
(q))( p ·
b∗
(k)).
Therefore, the amplitude for diagram A can be written as
iM
A
≈−¯u( p)e
i( p+k)x
0
g
2
t
a
t
b
d
4
q
(2π)
4
( p · A
a
(q))( p ·
b∗
(k))
[p
+
q
−
− i][p
+
(k
−
− q
−
) + i ]
e
iq(x
1
−x
0
)
J (p
+
+ k
+
− q
+
)
=−¯u( p)e
i( p+k)
−
x
+
0
g
2
t
a
t
b
d
2
q
⊥
(2π)
2
e
−iq
⊥
x
1⊥
( p ·
b∗
(k))
dq
+
2π
e
iq
+
x
−
1
J (p
+
+ k
+
− q
+
)(2π)δ(q
+
)
×
dq
−
2π
e
iq
−
(x
+
1
−x
+
0
)
p
+
A
−a
(q
−
, q
⊥
)
( p
+
)
2
[q
−
− i][k
−
− q
−
+ i]
, (6)
Fig. 3 Diagram B where the gluon is emitted after the interaction of
the quark with the target field
where in the last line we used Eq. (3) and we have set x
0⊥
=
x
−
0
= 0. Performing the q
+
and q
−
integrals we obtain
iM
A
≈−¯u( p)e
ipx
0
J (p
+
+ k
+
)g
2
t
a
t
b
d
2
q
⊥
(2π)
2
e
−iq
⊥
x
1⊥
p ·
b∗
(k)
×
i
e
ik
−
x
+
0
A
−a
(0, q
⊥
) − e
ik
−
x
+
1
A
−a
(k
−
, q
⊥
)
p
+
k
−
(x
+
1
− x
+
0
). (7)
Since the outgoing gluon is on-shell, k
−
= k
2
⊥
/2k
+
and,
furthermore, in the light cone gauge we have
∗−
(k) =
k
i
i
/k
+
. Therefore, making use of p
μ
∗
μ
≈ p
+
∗−
,we
obtain
iM
A
≈ 2i ¯u( p)e
ipx
0
J (p
+
+ k
+
)g
2
t
a
t
b
(x
+
1
− x
+
0
)
k
i
bi
k
2
⊥
×
d
2
q
⊥
(2π)
2
e
−iq
⊥
x
1⊥
e
ik
−
x
+
1
A
−a
(k
−
, q
⊥
) − e
ik
−
x
+
0
A
−a
(0, q
⊥
)
. (8)
Now, sending x
+
0
→−∞we can finally write
iM
A
≈ 2i ¯u( p)e
ipx
0
J (p
+
+ k
+
)g
2
t
a
t
b
e
ik
−
x
+
1
k
i
bi
k
2
⊥
d
2
q
⊥
(2π)
2
e
−iq
⊥
x
1⊥
A
−a
(k
−
, q
⊥
). (9)
Now we proceed to calculate diagram B where the gluon
is emitted with momentum k after the interaction of the quark
with the target field, as shown in Fig. 3. Following the pre-
vious procedure we find
123
600 Page 4 of 27 Eur. Phys. J. C (2019) 79 :600
Fig. 4 Diagram C where the emitted gluon interacts with the target
field
iM
B
≈−2i ¯u( p)e
ipx
0
J (p
+
+ k
+
)g
2
t
b
t
a
e
ik
−
x
+
1
k
i
bi
k
2
⊥
d
2
q
⊥
(2π)
2
e
−iq
⊥
x
1⊥
A
−a
(k
−
, q
⊥
). (10)
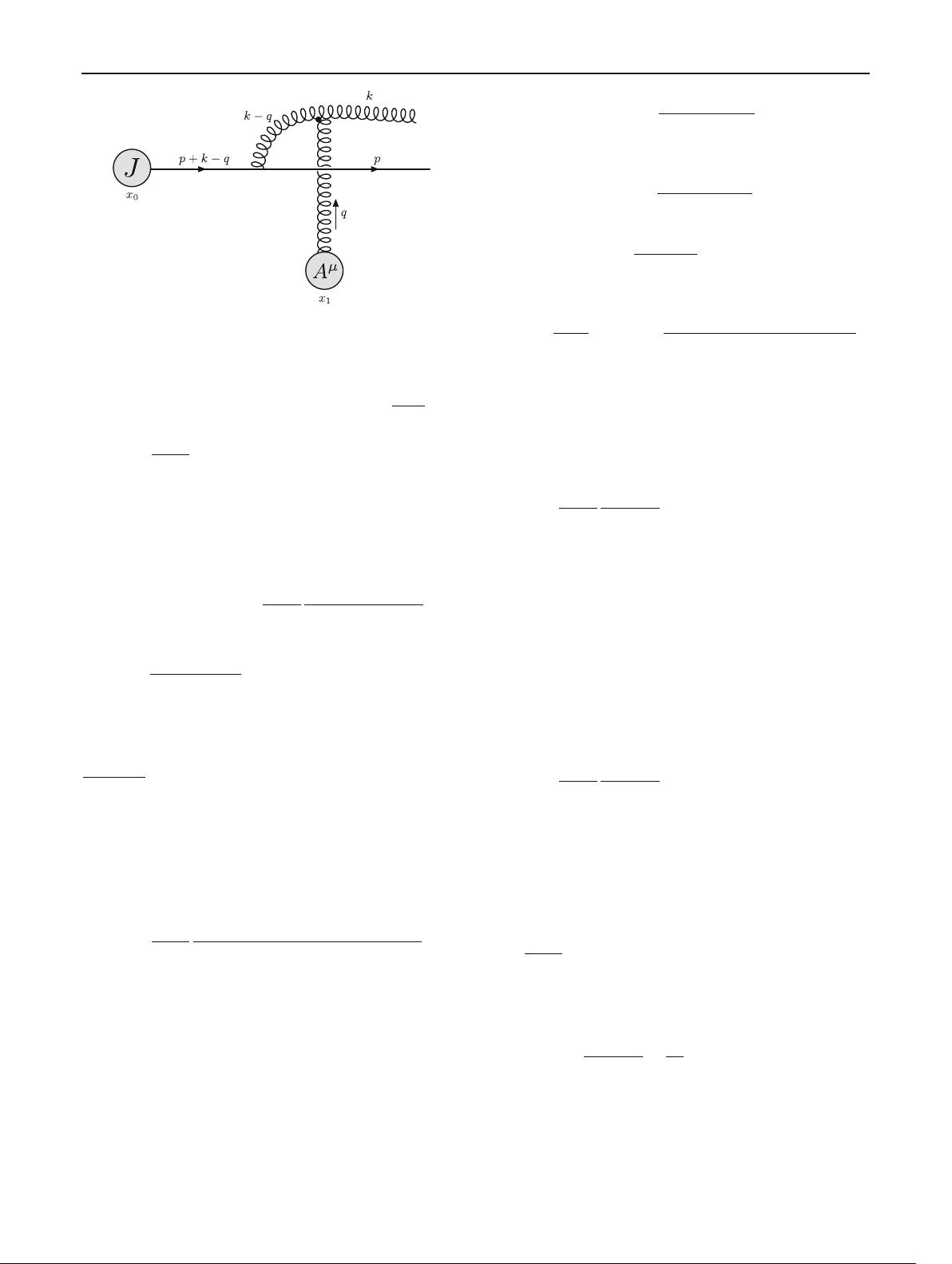
Diagram C, shown in Fig. 4, where the emitted gluon inter-
acts with the target field, requires dealing with the three-gluon
vertex. Applying the Feynman rules we have
iM
C
=¯u( p)(−igγ
μ
t
a
)
d
4
q
(2π)
4
i(
/
p +
/
k −
/
q)
( p + k − q)
2
+ i
e
i( p+k−q)x
0
J (p + k − q)
×
−id
μα
(k − q)
(k − q)
2
+ i
V
ανβ
abc
A
c
β
(q)
b∗
ν
(k)e
iqx
1
, (11)
where V
ανβ
abc
= gf
abc
g
αν
(q − 2k)
β
+ g
νβ
(k + q)
α
+ g
βα
(k − 2q)
ν
]
is the three-gluon vertex and d
μα
(k) = g
μα
−
k
μ
n
α
+k
α
n
μ
k·n
the gluon propagator in the light cone gauge.
Considering Eq. (3), we only need the V
αν+
abc
component
of the vertex. Furthermore, using the Dirac equation and
the gamma matrices anti-commutation relation we have that
¯u( p)γ
μ
/
p =¯u( p)2 p
μ
. Thus,
iM
C
=−2i ¯u( p)e
i( p+k)x
0
g
2
t
a
d
4
q
(2π)
4
p
μ
d
μα
(k − q)V
αν+
abc
b∗
ν
(k)
[( p + k − q)
2
+ i][(k − q)
2
+ i]
A
−c
(q)e
iq(x
1
−x
0
)
J (p
+
+ k
+
− q
+
). (12)
After some algebra we find, in the eikonal approximation,
p
μ
d
μα
(k − q)V
αν+
abc
b∗
ν
(k) ≈−2gf
abc
p
+
(k − q)
i
·
bi
,
(13)
and
(k − q)
2
=−2k
+
q
−
−
k
2
⊥
− (k − q)
2
⊥
2k
+
+ 2q
+
q
−
− 2k
−
q
+
≈
− 2k
+
q
−
−
k
2
⊥
− (k − q)
2
⊥
2k
+
. (14)
Thus, defining
˜
k =
k
2
⊥
−(k−q)
2
⊥
2k
+
, we get
iM
C
≈−i ¯u( p)g
2
t
a
f
abc
e
i( p+k)x
0
d
4
q
(2π)
4
(k − q)
i
bi
e
iq(x
1
−x
0
)
k
+
[k
−
− q
−
+ i][q
−
−
˜
k − i ]
A
−c
(q)J ( p
+
+ k
+
− q
+
). (15)
Using Eq. (3) and performing the q
+
and q
−
integrals we
obtain
iM
C
≈ 2 ¯u( p)J ( p
+
+ k
+
)e
ipx
0
g
2
t
a
f
abc
d
2
q
⊥
(2π)
2
(k − q)
i
(k − q)
2
⊥
bi
e
−iq
⊥
x
1⊥
× e
i
˜
kx
+
1
e
i(k
−
−
˜
k)x
+
0
A
−c
(
˜
k, q
⊥
)
−e
i(k
−
−
˜
k)x
+
1
A
−c
(k
−
, q
⊥
)
(x
+
1
− x
+
0
). (16)
Finally, making use of it
a
f
abc
=[t
b
, t
c
] and sending
x
+
0
→−∞, we obtain
iM
C
≈−2i ¯u( p)J ( p
+
+ k
+
)e
ipx
0
g
2
[t
a
, t
b
]
d
2
q
⊥
(2π)
2
(k − q)
i
(k − q)
2
⊥
bi
e
ik
−
x
+
1
A
−a
(k
−
, q
⊥
)e
−iq
⊥
x
1⊥
.
(17)
Summing up the three diagrams we get
i(M
A
+ M
B
+ M
C
)
≈−2i ¯u( p)J ( p
+
+ k
+
)e
ipx
0
g
2
[t
a
, t
b
]
d
2
q
⊥
(2π)
2
L
i
(k
⊥
, q
⊥
)
bi
e
ik
−
x
+
1
A
−a
(k
−
, q
⊥
)e
−iq
⊥
x
1⊥
,
(18)
where
L
i
(k
⊥
, q
⊥
) =
(k − q)
i
(k − q)
2
⊥
−
k
i
k
2
⊥
(19)
is the eikonal Lipatov vertex. We see that in our calculation,
as announced, the non-eikonal corrections result in the sum
of the amplitudes simply picking up a phase (important for
123

Eur. Phys. J. C (2019) 79 :600 Page 5 of 27 600
k
−
x
+
1
∼ 1 with k
−
∝ k
2
⊥
e
−η
and negligible for k
2
⊥
x
+
1
/k
+
1 where we recover the eikonal result) that can be absorbed
in a redefinition of the Lipatov vertex. Therefore, we define
a non-eikonal Lipatov vertex
L
i
NE
(k, q
⊥
;x
+
1
) =
(k − q)
i
(k − q)
2
⊥
−
k
i
k
2
⊥
e
i
k
2
⊥
2k
+
x
+
1
, (20)
with k
≡ (k
−
, k
⊥
).
As stated in the Introduction, this result is not new by
itself and similar calculations and expressions can be found
in the literature, e.g. in Refs. [75,76]orlaterinRef.[77]. But
the identification of this building block for its use to include
non-eikonal corrections in CGC calculations is done here for
the first time. Note that using the non-eikonal expression of
the gluon propagator from [5,11], the two first terms of the
expansion of the exponential were obtained in [12] and the
exponential form guessed.
3 Multi-particle production
In the previous section, we have presented the derivation
of the non-eikonal Lipatov vertex. Now, we would like to
use this expression in order to calculate multi-gluon produc-
tion cross section at mid rapidity within the Glasma graph
approach in order to study the effects of finite target width
corrections to those observables.
The double and triple inclusive gluon production cross
sections in p-A collisions have been recently studied in
[52,53] in the Glasma graph approximation, and in [58]
going beyond it, i.e. taking into account multiple scattering
effects of the dense target. For each observable, the contri-
butions to Bose enhancement of the projectile gluons and
HBT contributions of the final state gluons are identified.
However, the studies in [52,53,58] are performed within
the eikonal approximation without taking into account the
corrections due to the finite longitudinal width of the tar-
get.
In the rest of this section, we take this extra step.
Namely, we first expand the single, double and triple
inclusive gluon production cross section in powers of the
background field of the target which actually corresponds
to the original Glasma graph approach. Then, we intro-
duce the non-eikonal Lipatov vertex (20) in the expanded
cross sections and get the explicit expressions of the Bose
enhancement and HBT contributions beyond the strict
eikonal limit for the double and triple inclusive gluon pro-
duction. Hereafter, in order to alleviate the notation we
will drop the ⊥ for denoting transverse coordinates and
momenta.
3.1 Single inclusive gluon production beyond the eikonal
approximation
Within the CGC framework, the production cross section of
a gluon with transverse momenta k and rapidity η can be
written
dσ
d
2
kdη
= 4πα
s
z ¯z
e
ik(z−¯z)
xy
A
i
(x − z) A
i
(¯z − y)
ρ
a
(x)ρ
b
(y)
P
U
z
−U
x
ac
U
†
¯z
−U
†
y
cb
T
,
(21)
where ρ
a
(x) ≡ ρ
a
x
is the colour charge density of the pro-
jectile, ···
P(T )
denote the average over the projectile (tar-
get) colour configurations and A
i
is the standard Weizäcker-
Williams field that is defined as
A
i
(x − y) =−
1
2π
(x − y)
i
(x − y)
2
=
d
2
p
(2π)
2
e
−ip·(x−y)
p
i
p
2
.
(22)
Moreover, we have introduced a short hand notation for the
transverse coordinate integrals
z
=
d
2
z. Here, U
ab
x
is the
adjoint Wilson line in the colour field of the target represent-
ing the scattering matrix of a gluon at transverse position x,
whose explicit expression reads
U
ab
x
= P e
ig
dx
+
T
c
ab
A
−
c
(x
+
,x )
, (23)
with T
c
ab
being the SU(N
c
) generator in the adjoint represen-
tation and A
−
c
(x
+
, x) the colour field of the target. The Wil-
son line operator accounts for the multiple scattering effects
of the gluon in its interaction with the target. However, as
mentioned previously, the Glasma graph approach for dou-
ble (or multiple) gluon production corresponds to the dilute
limit of the target. Therefore, we expand the Wilson lines to
first order in the colour field of the target:
U
ab
(x) ≈ 1 + igT
c
ab
dx
+
A
−
c
(x
+
, x)
= 1 +igT
c
ab
dx
+
d
2
q
(2π)
2
e
iqx
A
−
c
(x
+
, q).
(24)
Using Eq. (24) we can write the single inclusive gluon pro-
duction cross section in the dilute limit as
dσ
d
2
kdη
dilute
= 4πα
s
z ¯z
e
ik(z−¯z)
xy
A
i
(x − z) A
i
(¯z − y)
ρ
a
(x)ρ
b
(y)
P
×g
2
dx
+
1
dx
+
2
d
2
q
1
(2π)
2
d
2
q
2
(2π)
2
123
剩余26页未读,继续阅读
资源评论

weixin_38616120
- 粉丝: 7
- 资源: 944
上传资源 快速赚钱
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
资源上传下载、课程学习等过程中有任何疑问或建议,欢迎提出宝贵意见哦~我们会及时处理!
点击此处反馈



安全验证
文档复制为VIP权益,开通VIP直接复制
 信息提交成功
信息提交成功