March 10, 2009 / Vol. 7, No. 3 / CHINESE OPTICS LETTERS 259
Unification of the Kirchhoff approximation and the method
of moment for optical wave scattering from the lossy
dielectric Gaussian random rough surface
Jun Ma (
æææ
)
∗
, Lixin Guo (
HHH
ááá
###
), and Xiangzhe Cheng (
§§§
óóó
)
School of Science, Xidian University, Xi’an 710071
∗
E-mail: clack2006@163.com
Received July 3, 2008
The optical wave scattering from one-dimensional (1D) lossy dielectric Gaussian random rough surface is
studied. The tapered incident wave is introduced into the classical Kirchhoff approximation (KA ), and th e
shadowing effect is also taken into account to make the KA results have a high accuracy. The definition
of the bistatic scattering coefficient of the modified KA and the method of moment (MOM) are unified.
The characteristics of the optical wave scattering from the lossy dielectric Gaussian random rough surface
of d ifferent parameters are analyzed by implementing MOM.
OCIS codes: 290.5880, 290.0290, 240.0240.
doi: 10.3788/COL20090703.0259.
The study on optical wave scattering from random rough
surface has been the subject of intensive investigation
over the past several decades for the application in a
number of important research areas such as characteri-
zation of films and optical interface, and the design of
optical scanning instrument for use in the semiconductor
industry
[1−6]
. The characteristic of optical wave scatter-
ing by one-dimensional (1D) conducting rough surface is
studied by the angular correlation functions of the po-
larized scattered intensities
[7]
. Among the many rough
surface theories for calculating scattering, the Kirch-
hoff approximation (KA)
[8]
and the method of moment
(MOM)
[9]
are widely used. KA is an analytical method
and MOM is a numerical method. In this letter, the
characteristic of optical wave scattering from the lossy di-
electric Gaussian random rough surface is studied by the
bistatic scattering coefficient. The tapered incidence,
which is commonly used in the MOM for calculating
rough surface scattering, is introduced into the classical
KA. The emphasis is put on studying the unification
of the definition of the bistatic scattering coefficient by
KA and MOM. The numerical results of the optical wave
scattering from the lossy dielectric rough surface are also
compared with these methods, and the influence of the
shadowing effect is taken into account in KA so as to
make the results have a high accuracy.
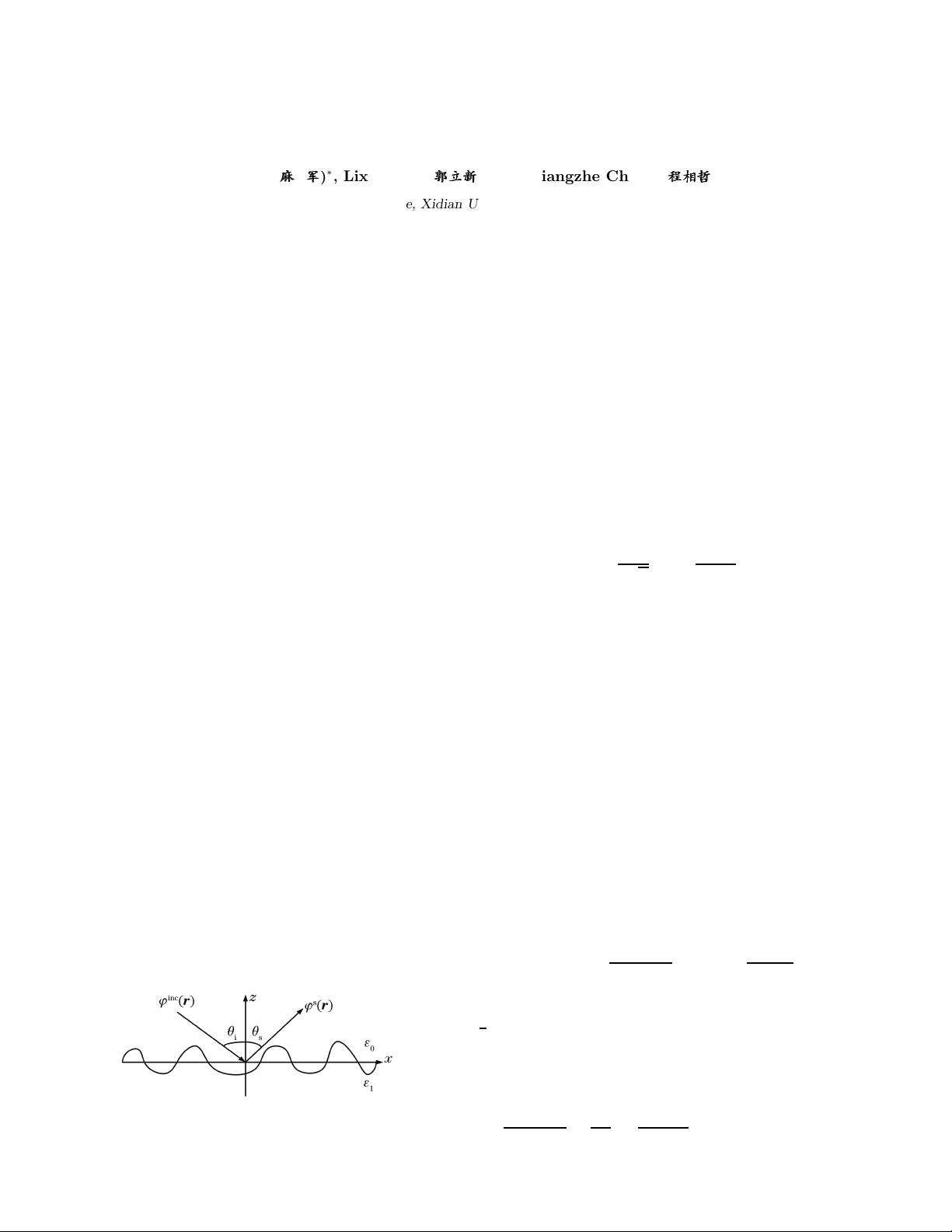
Considering an incident wave ϕ
inc
(~r) impinging upon
a random rough surface with the height profile z = f(x),
as shown in Fig. 1. ε
0
and ε
1
are the relative dielectric
constants of the free space and the lower medium space,
Fig. 1. Geometry of optical wave scattering from the Gaus-
sian rough surface.
and ε
0
= 1. f (x) is a Gaussian distributed rough surface
with the correlation length l and the root mean square
(RMS) height h. The spectral density of 1D Gaussian
random rough surface is
[8]
W (k) =
h
2
l
2
√
π
exp(−
−k
2
l
2
4
). (1)
In this letter, the optical wave scattering by 1D lossy
dielectric Gaussian rough surface is studied by the total
electric field for the horizontal polarization and the total
magnetic field for the vertical polarization, respectively.
For the horizontal polarization, the total electric field of
the optical wave is
~
E(~r) = ϕ(~r)ˆy, and for the vertical
polarization, the total magnetic field of the optical wave
is
~
H(~r) = ϕ(~r)ˆy. The time dependence is e
−iωt
.
From the spectral density, the Gaussian rough surface
f(x) can be simulated by Monte Carlo method
[9]
. In the
two-dimensional (2D) scattering problem ~r = xˆx + zˆz,
the total wave function ϕ(~r) is
ϕ(~r) = ϕ
inc
(~r) + ϕ
s
(~r), (2)
where ϕ
s
(~r) is the scattered wave, which can be written
as
[10]
ϕ
s
(~r) = ϕ(~r) − ϕ
inc
(~r)
=
Z
s
ϕ(~r
′
)
∂G(~r,~r
′
)
∂n
′
− G(~r, ~r
′
)
∂ϕ(~r
′
)
∂n
′
ds
′
, (3)
G(~r,~r
′
) is the 2D Green’s function and G(~r,~r
′
) =
i
4
H
(1)
0
(k
0
|~r −~r
′
|), where k
0
is the wave number of the
free space and H
(1)
0
(·) is the zeroth order Hankel function
of the first kind. The normal derivative of the Green’s
function is given by
[11]
∂G(~r,~r
′
)
∂n
′
=
ik
0
4
ˆn
′
·
~r −~r
′
|~r −~r
′
|
H
(1)
1
(k
0
|~r −~r
′
|), (4)
1671-7694/2009/030259-04
c
2009 Chinese Optics Letters
资源评论
