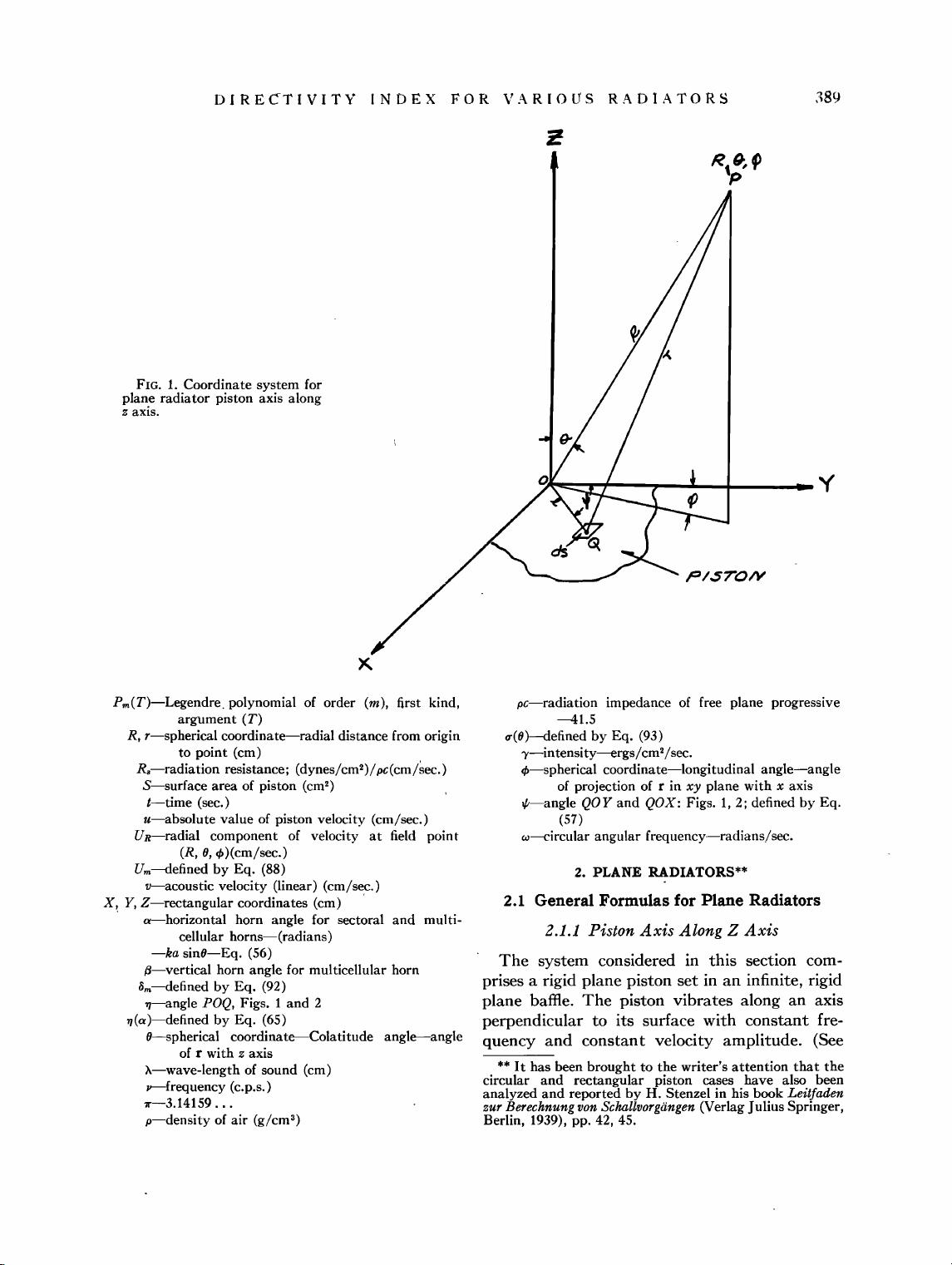
CalculationoftheDirectivityIndexforVariousTypesofRadiators资源-CSDN文库

需积分: 9 98 浏览量 2014-03-26 20:03:24 上传评论收藏 3.46MB PDF 举报

本文是一篇关于换能器指向性指数计算的研究性文章，该文由C.T.Molloy撰写，并发表于1948年的《美国声学学会杂志》。文章的主旨是为多种类型的声波辐射器提供指向性指数的计算公式及其推导过程，并给出实际案例下的相关图表。指向性指数（Directivity Index，缩写为DI）被定义为换能器在轴上某点产生的声压时，相对于产生同样声压的点声源的声功率输出的比率。这一概念的效用在于，它允许人们像计算点声源那样计算所有辐射器的功率。文章首先介绍了关于平面辐射器的指向性指数计算。对于平面辐射器，文章给出了两种情况下的通用分布积分：一种是活塞轴沿z轴方向，另一种是活塞轴沿y轴方向。这是因为总功率积分的评估便利性取决于坐标系的选择，并且对于矩形活塞和圆形活塞案例，分别采用了不同的坐标系统。接着，文章论述了曲面辐射器的指向性指数，包括扇形喇叭、多细胞喇叭和球形中的活塞式辐射器等类型。对于这些辐射器类型，文章也给出了计算指向性指数的通用公式，并配合图表详细说明了它们在实际应用中的表现。具体到内容细节上，指向性指数的定义和计算是基于这样一个事实：不同类型的辐射器在轴线上的指向性是不同的，而指向性指数是量度这一指向性差异的一个重要参数。对于平面活塞辐射器在无限障板中的情况，文中提供了几种不同情况的计算公式，这些公式考虑了活塞的几何形状、位置和工作频率等因素。文章还讨论了辐射阻抗（radiation resistance）与指向性指数之间的关系。辐射阻抗是辐射器在空气中产生声波时所遇到的阻抗，它会影响声波的发射效率。文中详细分析了辐射阻抗对平面活塞、圆形活塞和矩形活塞辐射器指向性指数的影响。此外，除了直接给出的指向性指数的理论计算公式，文中还提供了相关的曲线图，这些曲线图可以帮助设计工程师在实际设计时对不同类型的辐射器的指向性进行评估和选择。文章最后总结了所提出的指向性指数公式和曲线，并通过一系列的图表和数据，为音响工作中的换能器提供了详细的指向性分析工具。从上述内容中，我们可以得出如下几点关于换能器指向性计算的知识点： 1. 指向性指数（Directivity Index, DI）是描述辐射器指向性的参数，它表明了辐射器相对于点源声源的指向性效益。 2. 指向性指数的概念使我们能够以一种统一的方式，像处理点声源那样处理各种辐射器的功率计算。 3. 文章提供了包括平面、圆形和矩形活塞辐射器以及扇形喇叭、多细胞喇叭和球形中活塞式辐射器在内的多种辐射器类型的指向性指数计算公式。 4. 指向性指数的计算需要考虑辐射器的具体几何形状、位置、方向以及工作环境中的频率等因素。 5. 辐射阻抗是影响辐射器指向性的重要因素之一，文中对此进行了详细的分析。 6. 文章给出了计算公式的同时，还提供了实际应用中相关辐射器指向性指数的图表，以便于工程师在设计和评估扬声器系统时能够直观地理解和应用这些概念。以上这些知识点对于声学设计、扬声器工程以及声波传播等领域的研究和工程实践具有重要意义。通过这些详细的理论推导和实证分析，工程师可以更加精确地对不同辐射器的指向性进行评估和设计，以满足实际应用中的声学性能要求。

资源推荐

资源详情

资源评论

THE JOURNAL OF THE ACOUSTICAL SOCIETY OF AMERICA VOLUME 20, NUMBER 4 JULY, 194S

Calculation of the Directivity Index for Various Types of Radiators

C. T. MOLLOY

Bell Telephone Laboratories, Inc., Murray Hill, New Jersey

This paper gives the derivations of the "directivity index" formulas for several types of

sound radiators. The "directivity index" is defined as "the ratio of the total acoustic power

output of the radiator to the acoustic power output of a point source producing the same

pressure at the same point on the axis." The utility of the directivity index concept is that it

permits power calculations to be made for all radiators in the same manner as for point sources.

Directivity index formulas, together with graphs covering practical cases, are given for the

following types of radiators:

1. General plane piston in infinite baffle,

2. Circular plane pistion in infinite baffle,

3. Rectangular plane piston in infinite baffle,

4. Sectoral horn,

5. Multicellular horn,

6. Piston set in sphere.

TABLE OF CONTENTS

1. Introduction

1.1 Symbols

2. Plane radiation

2.1 General formula for D.I. for plane radiators

2.1.1. Piston axis along z axis

2.1.2 Pistori axis along y axis

2.2 Relation between radiation resistance (R•) and

directivity index (D.I.) for a rigid plane piston

2.3 Special cases of plane radiators

2.3.1 Circular piston

2.3.2 Rectangular piston

3. Curved surface radiators

3.1 Sectoral horn

3.2 Multicellular horn

3.3 Piston set in sphere

4. Summary

List of D.I. formulas and curves

1. INTRODUCTION

T is the purpose of this paper to present for-

mulas, together with their derivations and

graphs, which will permit the computation of the

"directivity index" (D.I.) for a variety of

radiators encountered in loudspeaker work.

The intention is first to treat plane radiators

and then curved surface radiators. Under the

former heading some general distribution inte-

grals are developed. These are given for two

cases, the first where the piston axis lies along

lhe z axis and the second where it lies along the

y axis. The reason for this is that the ease with

which the total power integrals are evaluated

depends upon the coordinates and it was found

convenient to use one system for the rectangular

lfiston and the other system for the circular

piston case. Next, general formulas valid for

plane radiators are given for the D.I. and the

radiation resistance. Finally the general plane

radiator formulas are applied to the cases of

circular piston and rectangular piston, each

mounted in an infinite baffle. The resulting D.I.

functions were graphed and the values for many

practical cases can be read from the graphs.

Under the classification of curved surface radi-

ators three cases were treated, namely, sectoral

horn, multicellular horn, piston set in sphere. In

the first two cases, what seemed to be reasonable

pressure distributions were assumed and the

resulting power output calculated by integration

and the D.I. finally computed. In these cases no

calculation of the radiation resistance was made

because this calculation requires a knowledge of

the relation between air velocity in the mouth

of the horn and the corresponding axial pressure

at an arbitrary distance from the mouth and the

assumptions made here did not provide this

information. The case of a piston set in a sphere

is based on analysis which is given by Morse. •

For all the curved surface radiators graphs are

given which permit the easy determination of the

D.I. for many practical cases.

In the derivation of the various D.I. formulas

several approximations and assumptions were

• P.M. Morse, Vibration and Sound (McGraw-Hill Book

Company, Inc., New York, 1936), p. 249.

387

Redistribution subject to ASA license or copyright; see http://acousticalsociety.org/content/terms. Download to IP: 221.212.116.28 On: Wed, 19 Mar 2014

10:56:58

388 C. T. MOLLOY

made and an effort has been made clearly to

indicate them whenever they occur. In the cases of

the sectoral and multicellular horns some sort of

assumptions were necessary because the theory

is not at present sufficiently developed to permit

a rigorous analytical solution. Consequently

pressure distribution functions were employed

which admittedly represent only first approxi-

mations to those which actually exist. In spite

of this, limited data indicate that the agreement

is not too bad.

The D.I. of a radiator may be defined as:*

The ratio of the total acoustic power output of

the radiator to the acoustic power output of a

point source producing the same pressure at the

same point on the axis.

The utility of this quantity arises from the

fact that so far as total power is concerned any

radiator of sound may be treated as a point

source having an appropriate correction factor,

i.e.D.I. (For an illustration showing the prac-

tical application of the D.I. concept see the

recent paper of Hopkins and Stryker. 2) In practice

it is sometimes desirable to convert pressure

measurements made with a microphone to total

power output. For the purpose of such calcula-

tions in this paper the assumption is made that

the pressure measurements are made sufficiently

far from the radiator so that the pressure and

velocity may be considered in phase at the point

of measurement. Specifically it is assumed that if

the instantaneous pressure function at a point is

p(t) = poei•t(dynes/cm •), (1)

then the instantaneous velocity function at the

same point is

v(t) =voe •t= (po/pc)ei•t(c•n/sec.). (2)

Hence the intensity at the point averaged over

one cycle is given by

2r/o•

•= (•/2r)f0 (PoS/pc) cos•'o•tdt

= (pos/2pc)(ergs/cm2/sec.). (3)

* It ma3 also be defined as minus ten times the logarithm

{ff the above ratio. The former definition and the results of

this paper are given in terms of the ratio although a db

scale is included on the curves for those who prefer this

system.

• H. F. Hopkins and N. Stryker, Proc. I.R.E. 36, 315

(1948).

Defining'

P ...... = p0/v•.

Then

•/=po•'/2pc = (p•'r.m.s./pc)(ergs/cm•'/sec.). (4)

For a point source the total power output is

Po = 4rr2•/ (ergs/sec.). (5)

For any other source the total power output (P)

is given by'

P = (D.I.)P0. (6)

An example illustrating the use of the Direc-

tivity Index is'

Given a radiator whose D.I. is 0.3 and which

produces an r.m.s. pressure of 10 dynes/cm • at

a distance of 10 feet from the radiator. Calculate

the total power output of the radiator.

Po=4rr2(p2r.,..•./pc) X 10 -*

=4,r(10 X 12 X 2.54)'" X (102/41.5) X 10 -•

= 0.281 watt;

P = 0.3 >< 0.281 = 0.0843 watt

=power output of radiator.

1.1 SYMBOLS

a--circular piston radius (cm)

« the (x) dimensions of rectangular piston (cm)

« the height of sectoral horn mouth (cm)

radius of sphere in "Piston Set in Sphere"

problem (cm)

b--« the Z dimension of rectangular piston (cm)

c--speed of sound 3.44 X 104 cm/sec.

D--circular piston diameter (inches)

D.I.--Directivity. Index (pure number)

Din--defined by Eq. (89)

e--base of natural logarithms 2.71828

h--height of sectoral horn mouth (inches)

I•, L--distribution integrals defined by Eqs. (20), (21)

and (31), (32)

j,•(Z)--spherical Bessel function of first kind defined by

Eq. (90)

Jv(Z)--Bessel function of first kind and order (P)

k--co/c= 2;rv/c= 2;r/X (cm -•)

/--radial distance on plane piston surface from

origin to element of area dS

L(0)--defined by Eq. (87)

M(a)--defined by Eq. (73)

nm(Z)--spherical Bessel function of second kind--defined

by Eq. (91)

p--acoustic pressure (dynes/cm :)

P--total power output from source (watts) or (ergs/

sec. )

Redistribution subject to ASA license or copyright; see http://acousticalsociety.org/content/terms. Download to IP: 221.212.116.28 On: Wed, 19 Mar 2014

10:56:58

DIRECTIV1TY INDEX FOR VARIOUS RADIATORS 389

Fio. 1. Coordinate system for

plane radiator piston axis along

z axis.

,01• 7'0

Pm(T)--Legendre. polynomial of order (m), first kind,

argument (T)

R, r--spherical coordinate--radial distance from origin

to point (cm)

R•--radiation resistance; (dynes/cm2)/pc(cm/sec.)

S--surface area of piston (cm 2) ,

t--time (sec.)

u--absolute value of piston velocity (cm/sec.)

UR--radial component of velocity at field point

(R, O, •)(cm/sec.)

Urn--defined by Eq. (88)

v--acoustic velocity (linear) (cm/sec.)

X, Y, Z--rectangular coordinates (cm)

a--horizontal horn angle for sectoral and multi-

cellular horns--(radians)

.ka sin0--Eq. (56)

•--vertical horn angle for multicellular horn

a,•--defined by Eq. (92)

ß t--angle POQ, Figs. 1 and 2

• (a )--defined by Eq. (65)

0--spherical coordinate--Colatitude angle--angle

of r with z axis

X--wave-length of sound (cm)

v--frequency (c.p.s.)

•r--3.14159...

p--density of air (g/cm a)

pc--radiation impedance of free plane progressive

---41.5

a(0)--defined by Eq. (93)

•,--intensity--ergs/cm •/sec.

•--spherical coordinate--longitudinal angle--angle

of projection of r in xy plane with x axis

•--angle QOY and QOX: Figs. 1, 2; defined by Eq.

(57)

o•--circular angular frequency--radians/sec.

2. PLANE RADIATORS**

2.1 General Formulas for Plane Radiators

2.1.1 Piston Axis Along Z Axis

The system considered in this section com-

prises a rigid plane piston set in an infinite, rigid

plane baffle. The piston vibrates along an axis

perpendicular to its surface with constant fre-

quency and constant velocity amplitude. (See

** It has been brought to the writer's attention that the

circular and rectangular piston cases have also been

analyzed and reported by H. Stenzel in his book Leitfaden

zur Berechnung yon Schallvorgt•ngen (Verlag Julius Springer,

Berlin, 1939), pp. 42, 45.

Redistribution subject to ASA license or copyright; see http://acousticalsociety.org/content/terms. Download to IP: 221.212.116.28 On: Wed, 19 Mar 2014

10:56:58

剩余18页未读，继续阅读

评论收藏

内容反馈