Propeller performance characteristics 103
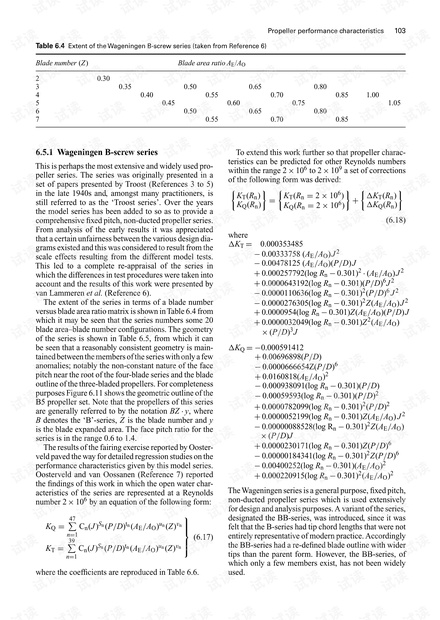
Table 6.4 Extent of the Wageningen B-screw series (taken from Reference 6)
Blade number (Z) Blade area ratio A
E
/A
O
2 0.30
3 0.35 0.50 0.65 0.80
4 0.40 0.55 0.70 0.85 1.00
5 0.45 0.60 0.75 1.05
6 0.50 0.65 0.80
7 0.55 0.70 0.85
6.5.1 Wageningen B-screw series
This is perhaps the most extensive and widely used pro-
peller series. The series was originally presented in a
set of papers presented by Troost (References 3 to 5)
in the late 1940s and, amongst many practitioners, is
still referred to as the ‘Troost series’. Over the years
the model series has been added to so as to provide a
comprehensive fixed pitch, non-ducted propeller series.
From analysis of the early results it was appreciated
that a certain unfairness between the various design dia-
grams existed and this was considered to result from the
scale effects resulting from the different model tests.
This led to a complete re-appraisal of the series in
which the differences in test procedures were taken into
account and the results of this work were presented by
van Lammeren et al. (Reference 6).
The extent of the series in terms of a blade number
versus blade area ratio matrix is shown inTable 6.4 from
which it may be seen that the series numbers some 20
blade area–blade number configurations. The geometry
of the series is shown in Table 6.5, from which it can
be seen that a reasonably consistent geometry is main-
tained between the members of the series with only a few
anomalies; notably the non-constant nature of the face
pitch near the root of the four-blade series and the blade
outline of the three-bladed propellers. For completeness
purposes Figure 6.11 shows the geometric outline of the
B5 propeller set. Note that the propellers of this series
are generally referred to by the notation BZ ·y, where
B denotes the ‘B’-series, Z is the blade number and y
is the blade expanded area. The face pitch ratio for the
series is in the range 0.6 to 1.4.
The results of the fairing exercise reported by Ooster-
veld paved the way for detailed regression studies on the
performance characteristics given by this model series.
Oosterveld and van Oossanen (Reference 7) reported
the findings of this work in which the open water char-
acteristics of the series are represented at a Reynolds
number 2 ×10
6
by an equation of the following form:
K
Q
=
47
n=1
C
n
(J )
S
n
(P/D)
t
n
(A
E
/A
O
)
u
n
(Z)
v
n
K
T
=
39
n=1
C
n
(J )
S
n
(P/D)
t
n
(A
E
/A
O
)
u
n
(Z)
v
n
⎫
⎪
⎪
⎬
⎪
⎪
⎭
(6.17)
where the coefficients are reproduced in Table 6.6.
To extend this work further so that propeller charac-
teristics can be predicted for other Reynolds numbers
within the range 2 ×10
6
to 2 ×10
9
a set of corrections
of the following form was derived:
K
T
(R
n
)
K
Q
(R
n
)
=
K
T
(R
n
= 2 × 10
6
)
K
Q
(R
n
= 2 × 10
6
)
+
K
T
(R
n
)
K
Q
(R
n
)
(6.18)
where
K
T
= 0.000353485
−0.00333758 (A
E
/A
O
)J
2
−0.00478125 (A
E
/A
O
)(P/D)J
+0.000257792(log R
n
−0.301)
2
·(A
E
/A
O
)J
2
+0.0000643192(log R
n
−0.301)(P/D)
6
J
2
−0.0000110636(log R
n
−0.301)
2
(P/D)
6
J
2
−0.0000276305(log R
n
−0.301)
2
Z(A
E
/A
O
)J
2
+0.0000954(log R
n
−0.301)Z(A
E
/A
O
)(P/D)J
+0.0000032049(log R
n
−0.301)Z
2
(A
E
/A
O
)
×(P/D)
3
J
K
Q
=−0.000591412
+0.00696898(P/D)
−0.0000666654Z(P/D)
6
+0.0160818(A
E
/A
O
)
2
−0.000938091(log R
n
−0.301)(P/D)
−0.00059593(log R
n
−0.301)(P/D)
2
+0.0000782099(log R
n
−0.301)
2
(P/D)
2
+0.0000052199(log R
n
−0.301)Z(A
E
/A
O
) J
2
−0.00000088528(log R
n
−0.301)
2
Z(A
E
/A
O
)
×(P/D)J
+0.0000230171(log R
n
−0.301)Z(P/D)
6
−0.00000184341(log R
n
−0.301)
2
Z(P/D)
6
−0.00400252(log R
n
−0.301)(A
E
/A
O
)
2
+0.000220915(log R
n
−0.301)
2
(A
E
/A
O
)
2
The Wageningen series is a general purpose, fixed pitch,
non-ducted propeller series which is used extensively
for design and analysis purposes. A variant of the series,
designated the BB-series, was introduced, since it was
felt that the B-series had tip chord lengths that were not
entirely representative of modern practice. Accordingly
the BB-series had a re-defined blade outline with wider
tips than the parent form. However, the BB-series, of
which only a few members exist, has not been widely
used.
资源评论
qq_42533957
- 粉丝: 0
- 资源: 2
最新资源
- 使用 Java EE 7 的 Java Petstore.zip
- (源码)基于Qt和SQL Server的实验室设备管理系统.zip
- 使用 HTML、CSS 和 JAVASCRIPT 在 100 天内构建 100 多个项目.zip
- (源码)基于Python和Thingsboard框架的温湿度数据模拟与导出系统.zip
- 使用 HTML CSS 和 JavaScript 制作的项目.zip
- (源码)基于Python和Postgresql的图书管理系统.zip
- VID_20241125022451.mp4
- (源码)基于SSM框架的顶铮快递管理系统.zip
- 从 Java 到 Kotlin - 从 Java 到 Kotlin 的速查表.zip
- (源码)基于Spring Boot框架的项目管理系统.zip
资源上传下载、课程学习等过程中有任何疑问或建议，欢迎提出宝贵意见哦~我们会及时处理！
点击此处反馈
