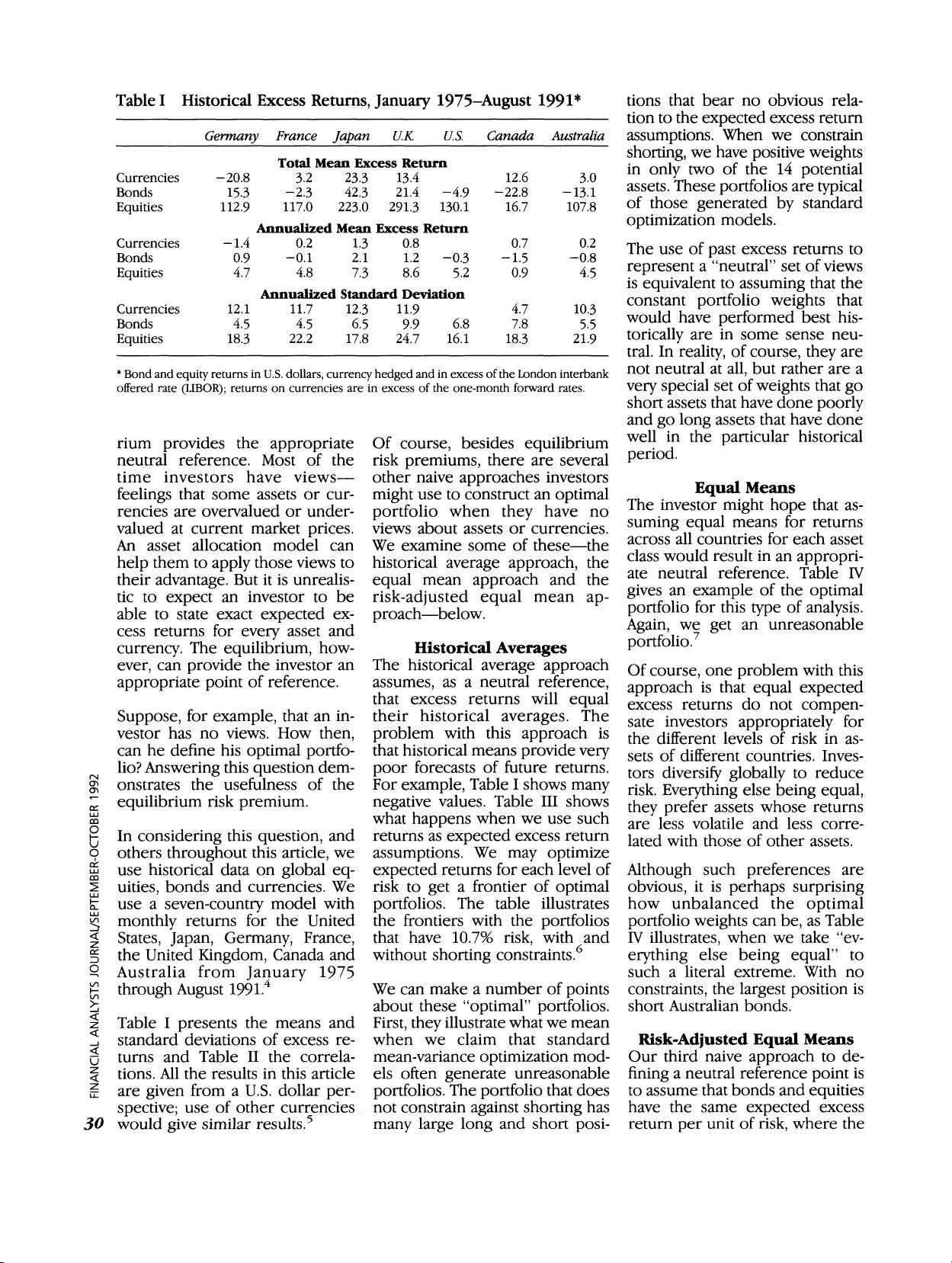
Table
I
Historical Excess Returns, January 1975-August 1991*
Germany France Japan UK
US.
Canada Australia
Total Mean Excess Return
Currencies
-20.8
3.2 23.3 13.4
12.6
3.0
Bonds 15.3 -2.3 42.3 21.4 -4.9 -22.8 -13.1
Equities 112.9 117.0 223.0 291.3 130.1 16.7 107.8
Annualized Mean Excess Return
Currencies
-1.4
0.2
1.3
0.8
0.7
0.2
Bonds
0.9
-0.1
2.1
1.2
-0.3 -1.5 -0.8
Equities 4.7
4.8
7.3
8.6
5.2 0.9 4.5
Annualized Standard Deviation
Currencies
12.1
11.7 12.3 11.9 4.7 10.3
Bonds
4.5 4.5 6.5 9.9
6.8 7.8
5.5
Equities 18.3
22.2
17.8 24.7 16.1 18.3 21.9
*
Bond and equity returns
in
U.S. dollars, currency hedged and
in
excess
of the London
interbank
offered rate (LIBOR); returns on currencies are
in
excess of
the
one-month forward rates.
on
cn
0
U
LL
cca
z
30
rium provides
the appropriate
neutral reference.
Most of
the
time
investors have
views-
feelings
that some assets or cur-
rencies are
overvalued
or under-
valued at
current market
prices.
An asset allocation model
can
help them
to apply those
views to
their
advantage. But it
is unrealis-
tic
to
expect
an investor
to be
able
to state exact expected
ex-
cess returns for every
asset
and
currency.
The
equilibrium,
how-
ever,
can provide the
investor
an
appropriate
point
of
reference.
Suppose,
for
example,
that an
in-
vestor has
no views.
How then,
can
he
define
his
optimal
portfo-
lio? Answering
this question
dem-
onstrates
the usefulness of
the
equilibrium
risk
premium.
In
considering
this question,
and
others
throughout
this
article,
we
use historical
data on
global eq-
uities,
bonds
and currencies.
We
use
a
seven-country
model
with
monthly
returns
for the United
States, Japan,
Germany, France,
the
United
Kingdom,
Canada
and
Australia
from January
1975
through
August 1991.4
Table
I
presents
the
means and
standard
deviations of excess
re-
turns and Table
II
the correla-
tions.
All
the results
in
this article
are
given
from
a U.S. dollar
per-
spective;
use of other currencies
would
give
similar results.5
Of
course,
besides
equilibrium
risk
premiums, there are several
other
naive approaches investors
might use
to
construct an
optimal
portfolio
when they have no
views
about assets or currencies.
We
examine some
of
these-the
historical
average approach, the
equal
mean
approach
and the
risk-adjusted equal mean ap-
proach-below.
Historical Averages
The
historical average approach
assumes,
as a neutral
reference,
that excess returns
will
equal
their
historical averages. The
problem
with this approach is
that historical means
provide very
poor
forecasts of future returns.
For
example,
Table
I
shows
many
negative
values. Table
III
shows
what happens when
we
use
such
returns as
expected
excess return
assumptions.
We
may optimize
expected returns
for each level of
risk to
get
a frontier
of
optimal
portfolios.
The table illustrates
the frontiers with the
portfolios
that have
10.7%
risk,
with and
without
shorting
constraints.6
We can make a number
of
points
about
these
"optimal" portfolios.
First, they
illustrate what we
mean
when
we
claim
that
standard
mean-variance
optimization mod-
els often
generate
unreasonable
portfolios.
The
portfolio
that does
not
constrain
against shorting
has
many large
long
and short
posi-
tions that
bear no
obvious rela-
tion to the
expected
excess return
assumptions.
When we constrain
shorting,
we have
positive weights
in only two of the 14 potential
assets.
These
portfolios
are
typical
of
those generated by standard
optimization
models.
The use of past excess returns to
represent a "neutral" set of views
is equivalent to assuming that the
constant portfolio weights that
would have performed best his-
torically are
in
some sense
neu-
tral.
In
reality, of course, they are
not neutral at all, but rather are a
very special set of weights that go
short assets that have done
poorly
and go long assets that
have
done
well
in
the particular historical
period.
Equal
Means
The investor might hope that as-
suming equal
means for
returns
across
all
countries for each
asset
class would result
in
an
appropri-
ate neutral reference. Table
IV
gives an example
of
the optimal
portfolio
for this
type
of
analysis.
Again,
we
get
an unreasonable
portfolio.7
Of
course,
one
problem
with
this
approach
is
that
equal expected
excess returns do not
compen-
sate investors
appropriately
for
the different levels
of risk in
as-
sets of different countries. Inves-
tors
diversify globally
to reduce
risk. Everything
else
being equal,
they prefer
assets whose
returns
are less volatile and less corre-
lated with
those of
other assets.
Although
such
preferences
are
obvious,
it
is
perhaps surprising
how unbalanced the
optimal
portfolio weights
can
be,
as Table
IV
illustrates,
when we
take
"ev-
erything
else
being equal"
to
such
a literal extreme. With no
constraints,
the
largest position
is
short Australian bonds.
Risk-Adjusted Equal
Means
Our third naive
approach
to de-
fining a neutral reference point is
to assume that bonds and equities
have the same expected excess
return per unit of risk, where
the




 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助 
 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜

 信息提交成功
信息提交成功