
电力系统基于matlab多时间尺度滚动优化的多能源微网双层调度模型【含Matlab源码 2281期】.zip

2.虚拟产品一经售出概不退款(资源遇到问题,请及时私信上传者)
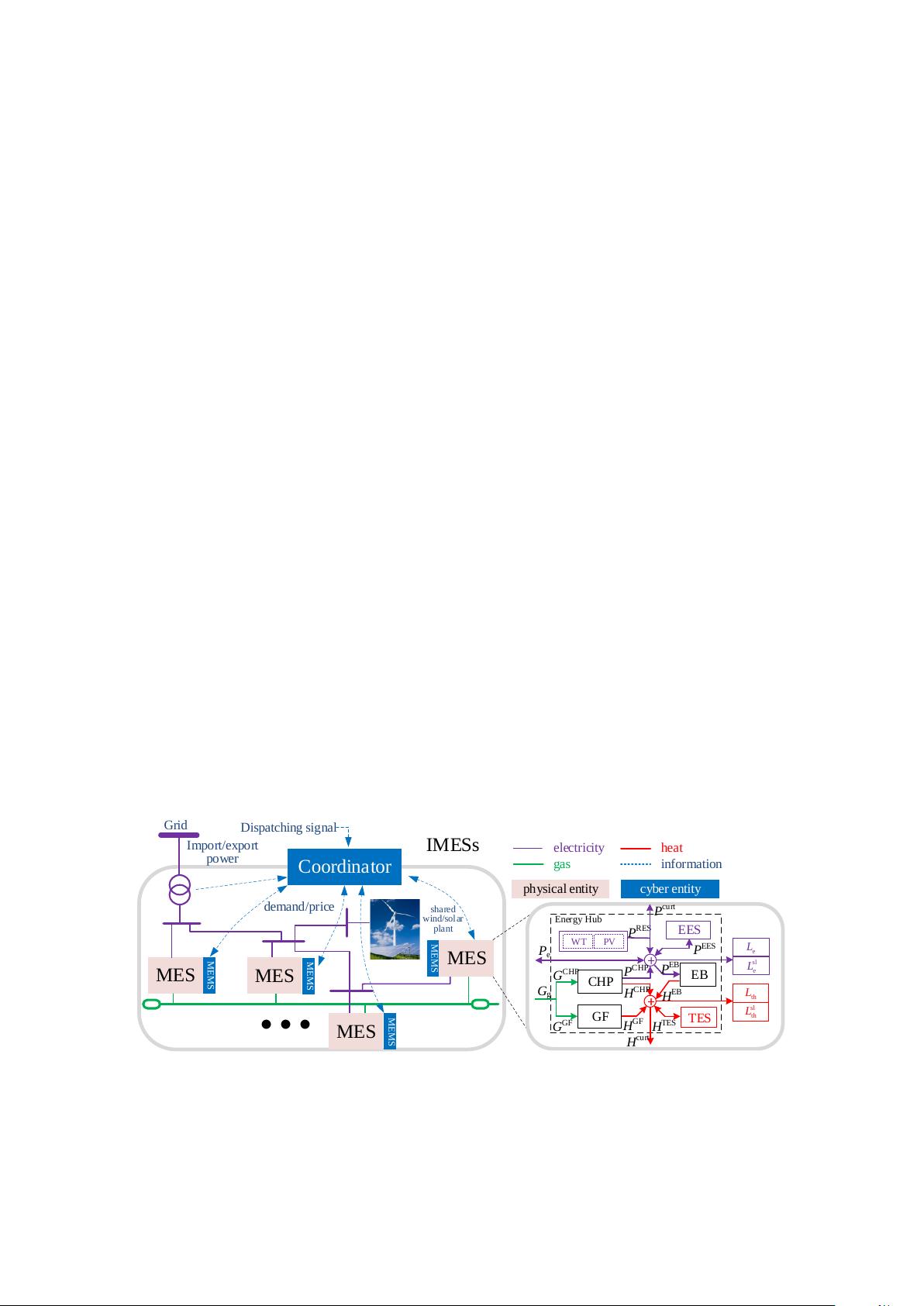
电力系统在当今能源结构中的地位日益重要,而随着可再生能源的普及,多能源微网的调度问题成为研究焦点。基于MATLAB的多时间尺度滚动优化技术在此类问题中发挥着关键作用,它能够有效地整合不同能源类型,实现高效、经济且可靠的微网运行。这个项目“电力系统基于matlab多时间尺度滚动优化的多能源微网双层调度模型”提供了完整的源码,适合于学习和实践这一先进理念。 我们要理解多时间尺度的概念。在电力系统调度中,时间尺度可以分为小时级、分钟级甚至秒级,不同的时间尺度对应着不同的决策周期。多时间尺度优化考虑了各个时间层次上的运行约束和目标,以适应系统动态变化,确保系统的稳定性和经济效益。 滚动优化是一种动态规划策略,它在每次迭代时更新未来预测,逐步逼近最优解。在多能源微网调度中,滚动优化可以应对不确定性,如风能、太阳能的波动,以及负荷需求的变化,确保微网的供需平衡。 双层调度模型则将整个微网的调度分为上层和下层两个层次。上层通常负责全局优化,考虑微网的整体运营策略,包括能源的采购、存储和转换等;下层则负责更具体的控制决策,如各个分布式能源的功率分配,储能设备的状态管理等。这种分层设计有助于分解复杂问题,提高求解效率。 在MATLAB环境下,我们可以利用其强大的数值计算和优化工具箱,如Global Optimization Toolbox(全局优化工具箱)和Optimization Toolbox(优化工具箱),实现多能源微网的双层调度模型。2014a和2019b版本的MATLAB都支持这些功能,用户可以根据自己的环境选择合适版本运行代码。 源码的提供使得学习者可以直接进行仿真实验,理解模型的构建、算法的实现以及结果的解析。通过实际操作,可以深入掌握电力系统调度的理论与方法,同时提升MATLAB编程能力。对于研究者和工程师来说,这是一份宝贵的参考资料,能够帮助他们在实际项目中应用这些先进的优化策略,提升微网运行的效率和可靠性。 这个项目涵盖了电力系统调度中的关键技术和应用,结合了多时间尺度优化、滚动优化和双层调度模型,是MATLAB在能源领域的典型应用案例。通过深入学习和实践,不仅可以提升对电力系统运行的理解,还可以为可再生能源的集成和智能电网的发展打下坚实基础。
 电力系统基于matlab多时间尺度滚动优化的多能源微网双层调度模型【含Matlab源码 2281期】.zip (111个子文件)
电力系统基于matlab多时间尺度滚动优化的多能源微网双层调度模型【含Matlab源码 2281期】.zip (111个子文件)  EH_local_170828_v3.m 43KB
EH_local_170828_v3.m 43KB EH_local_170828_v3.m 43KB
EH_local_170828_v3.m 43KB main_handle.m 16KB
main_handle.m 16KB main_handle.m 16KB
main_handle.m 16KB main_handle_MILP.m 10KB
main_handle_MILP.m 10KB main_handle_MILP.m 10KB
main_handle_MILP.m 10KB comments.m 9KB
comments.m 9KB comments.m 9KB
comments.m 9KB para_init.m 9KB
para_init.m 9KB para_init.m 9KB
para_init.m 9KB single_temporal.m 6KB
single_temporal.m 6KB single_temporal.m 6KB
single_temporal.m 6KB all_temporal.m 6KB
all_temporal.m 6KB all_temporal.m 6KB
all_temporal.m 6KB predict.m 5KB
predict.m 5KB predict.m 5KB
predict.m 5KB iterativeClear.m 5KB
iterativeClear.m 5KB iterativeClear.m 5KB
iterativeClear.m 5KB main_milp.m 4KB
main_milp.m 4KB main_milp.m 4KB
main_milp.m 4KB main_central.m 4KB
main_central.m 4KB main_central.m 4KB
main_central.m 4KB clearing.m 3KB
clearing.m 3KB clearing.m 3KB
clearing.m 3KB drawMES_stacked.m 3KB
drawMES_stacked.m 3KB drawMES_stacked.m 3KB
drawMES_stacked.m 3KB Grid_171118.m 3KB
Grid_171118.m 3KB Grid_171118.m 3KB
Grid_171118.m 3KB convex_relaxation.m 3KB
convex_relaxation.m 3KB convex_relaxation.m 3KB
convex_relaxation.m 3KB main_170828_v3.m 2KB
main_170828_v3.m 2KB main_170828_v3.m 2KB
main_170828_v3.m 2KB IESdemand_curve.m 1KB
IESdemand_curve.m 1KB IESdemand_curve.m 1KB
IESdemand_curve.m 1KB drawMES.m 1KB
drawMES.m 1KB drawMES.m 1KB
drawMES.m 1KB plotAux.m 1000B
plotAux.m 1000B plotAux.m 1000B
plotAux.m 1000B calculate_accomodation_rate.m 985B
calculate_accomodation_rate.m 985B calculate_accomodation_rate.m 985B
calculate_accomodation_rate.m 985B main_distribute.m 744B
main_distribute.m 744B main_distribute.m 744B
main_distribute.m 744B main.m 739B
main.m 739B main.m 739B
main.m 739B appendStairArray.m 505B
appendStairArray.m 505B appendStairArray.m 505B
appendStairArray.m 505B ColorHex.m 492B
ColorHex.m 492B ColorHex.m 492B
ColorHex.m 492B c.mat 112KB
c.mat 112KB c.mat 112KB
c.mat 112KB c.mat 112KB
c.mat 112KB collaborate.mat 99KB
collaborate.mat 99KB collaborate.mat 99KB
collaborate.mat 99KB d.mat 95KB
d.mat 95KB d.mat 95KB
d.mat 95KB d.mat 95KB
d.mat 95KB collaborate_feedin.mat 78KB
collaborate_feedin.mat 78KB collaborate_feedin.mat 78KB
collaborate_feedin.mat 78KB central.mat 73KB
central.mat 73KB central.mat 73KB
central.mat 73KB collaborate.mat 71KB
collaborate.mat 71KB collaborate_feedin.mat 63KB
collaborate_feedin.mat 63KB collaborate.mat 60KB
collaborate.mat 60KB autonomous.mat 53KB
autonomous.mat 53KB collaborate_feedin.mat 48KB
collaborate_feedin.mat 48KB autonomous.mat 41KB
autonomous.mat 41KB autonomous.mat 41KB
autonomous.mat 41KB autonomous.mat 34KB
autonomous.mat 34KB central.mat 28KB
central.mat 28KB tmp2.mat 15KB
tmp2.mat 15KB tmp2.mat 15KB
tmp2.mat 15KB tmp2.mat 15KB
tmp2.mat 15KB tmp1.mat 4KB
tmp1.mat 4KB tmp1.mat 4KB
tmp1.mat 4KB tmp1.mat 4KB
tmp1.mat 4KB solarValue.mat 3KB
solarValue.mat 3KB solarValue.mat 3KB
solarValue.mat 3KB solarValue.mat 3KB
solarValue.mat 3KB windValue.mat 2KB
windValue.mat 2KB windValue.mat 2KB
windValue.mat 2KB windValue.mat 2KB
windValue.mat 2KB gridPriceRecord.mat 1KB
gridPriceRecord.mat 1KB gridPriceRecord.mat 1KB
gridPriceRecord.mat 1KB gridPriceRecord.mat 1KB
gridPriceRecord.mat 1KB singleLoadValue.mat 957B
singleLoadValue.mat 957B singleLoadValue.mat 957B
singleLoadValue.mat 957B singleLoadValue.mat 957B
singleLoadValue.mat 957B singleLoadValue1.mat 947B
singleLoadValue1.mat 947B singleLoadValue1.mat 947B
singleLoadValue1.mat 947B singleLoadValue1.mat 947B
singleLoadValue1.mat 947B singleWindValue.mat 795B
singleWindValue.mat 795B singleWindValue.mat 795B
singleWindValue.mat 795B singleWindValue.mat 795B
singleWindValue.mat 795B renewableName.mat 338B
renewableName.mat 338B renewableName.mat 338B
renewableName.mat 338B renewableName.mat 338B
renewableName.mat 338B 多时间尺度微网双层市场交易优化.pdf 676KB
多时间尺度微网双层市场交易优化.pdf 676KB 复现Paper.pdf 676KB
复现Paper.pdf 676KB 55.png 149KB
55.png 149KB 66.png 125KB
66.png 125KB- 1
- 2


- 粉丝: 5w+
- 资源: 6477
 我的内容管理
展开
我的内容管理
展开
 我的资源
快来上传第一个资源
我的资源
快来上传第一个资源
 我的收益 登录查看自己的收益
我的收益 登录查看自己的收益 我的积分
登录查看自己的积分
我的积分
登录查看自己的积分
 我的C币
登录后查看C币余额
我的C币
登录后查看C币余额
 我的收藏
我的收藏  我的下载
我的下载  下载帮助
下载帮助

 前往需求广场,查看用户热搜
前往需求广场,查看用户热搜最新资源
- OA办公自动化管理系统(Struts1.2+Hibernate3.0+Spring2+DWR).rar
- OA办公自动化管理系统(Struts1.2+Hibernate3.0+Spring2+DWR)130224.rar
- shopxx_src.rar
- 聊天系统项目全套技术资料100%好用.zip
- tot-jsp-cms.rar
- s2shDemo.rar
- webdgs.rar
- vijun-1.0-release.rar
- 博客系统网站(JSP+SERVLET+MYSQL).rar
- 博客系统网站(JSP+SERVLET+MYSQL)130222.rar
- 博客系统(struts+hibernate+spring)130225.rar
- 超市综合管理信息系统.rar
- 数据爬虫项目全套技术资料100%好用.zip
- 车辆管理系统(struts+hibernate+spring+oracle)130225.rar
- 车辆管理系统(struts+hibernate+spring+oracle).rar
- 共创在线考试系统(JSP+SERVLET).rar


 信息提交成功
信息提交成功


- 1
- 2
前往页